A person with body resistance between his hands of 10KΩ accidentally grasps the terminals of a 18kV power supply.
(i) If the internal resistance of the power supply is 2000Ω, what is the power dissipated in his body?
(ii) What is the power dissipated in his body?
(iii) If the power supply is to be made safe by increasing its internal resistance, what should the internal resistance be for the maximum current in the above situation to be 1.00mA or less?
Video Solution
Text Solution
Given R=10kΩandV=18Kv.
(i) To find the current fl,owing through the body , we need to sum up the resistances present in the circuit and divide the voltage by it .
I=VR+r=18×10310×103+2×103=18×10312×103
=32=1.5A
(ii) Power dissipated is
V1=I2R=(1.5)2(10000)=2.25×10000
=22500=22.5Kw
(iii) To find the internal resistance for the safe limit of power , we can use the formula as in part i. The only difference here is I is given and r is to be calculated.
R+r=VI=18×1031×10−3=18×106
or r=18×106−10×103=(18MΩ−10KΩ)
≅18MΩ
|
Topper's Solved these Questions
- HEATING EFFECT OF CURRENTBook:CENGAGE PHYSICSChapter:HEATING EFFECT OF CURRENTExercise:Exercise 7.1
Explore 22 Videos
- HEATING EFFECT OF CURRENTBook:CENGAGE PHYSICSChapter:HEATING EFFECT OF CURRENTExercise:Subjective
Explore 8 Videos
- HEATING EFFECT OF CURRENTBook:CENGAGE PHYSICSChapter:HEATING EFFECT OF CURRENTExercise:Thermal Power in Resistance Connected in Circuit
Explore 28 Videos
- GRAVITATIONBook:CENGAGE PHYSICSChapter:GRAVITATIONExercise:Question Bank
Explore 39 Videos
- INDUCTANCEBook:CENGAGE PHYSICSChapter:INDUCTANCEExercise:Concept Based
Explore 9 Videos
Similar Questions
Knowledge Check
If the power dissipated in 5Ω is 20W then power dissipated in 4Ω is:-
 A4WB6WC10WD20W
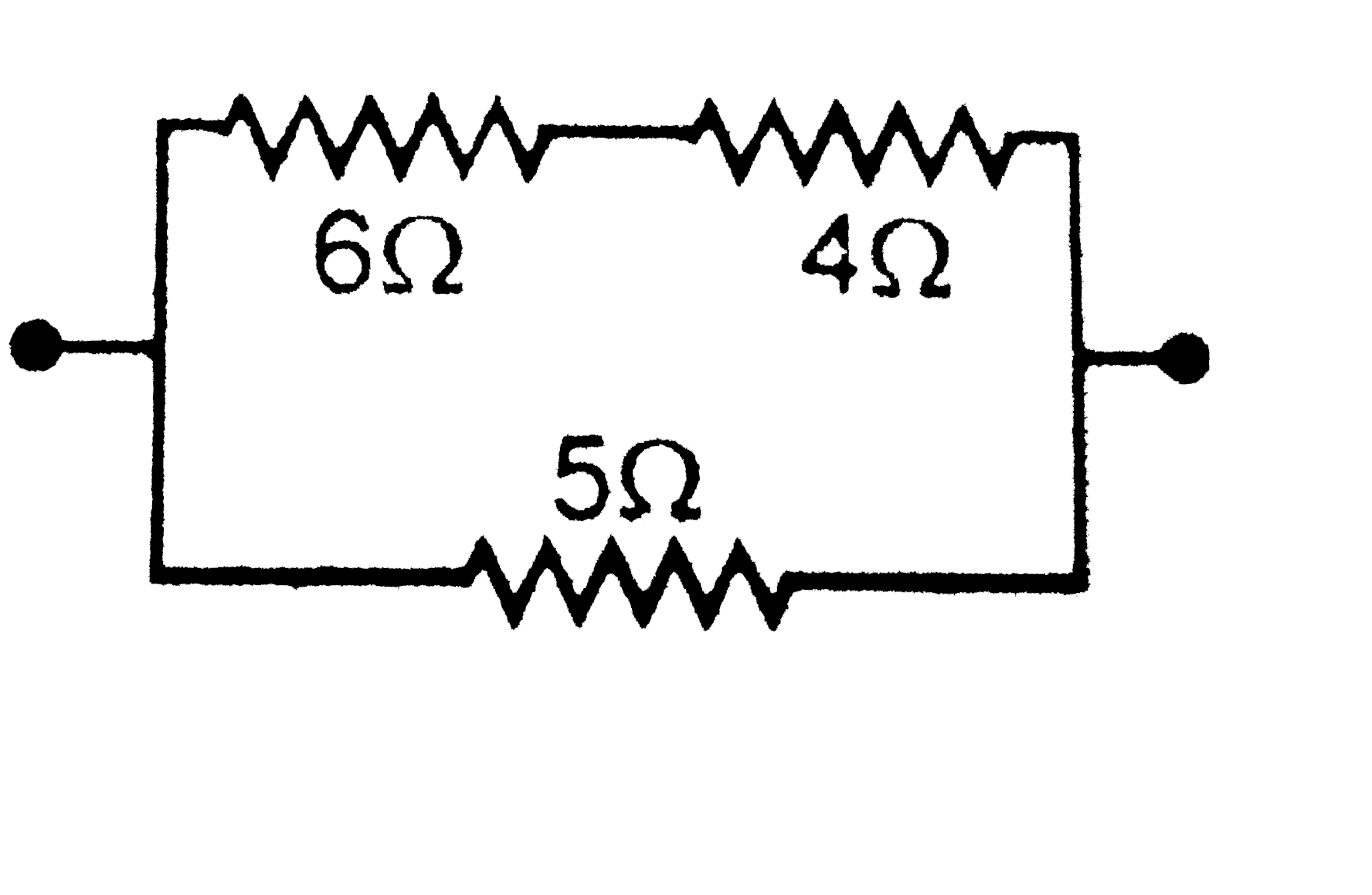
A4WB6WC10WD20WThe power dissipated 6Ω resistor is
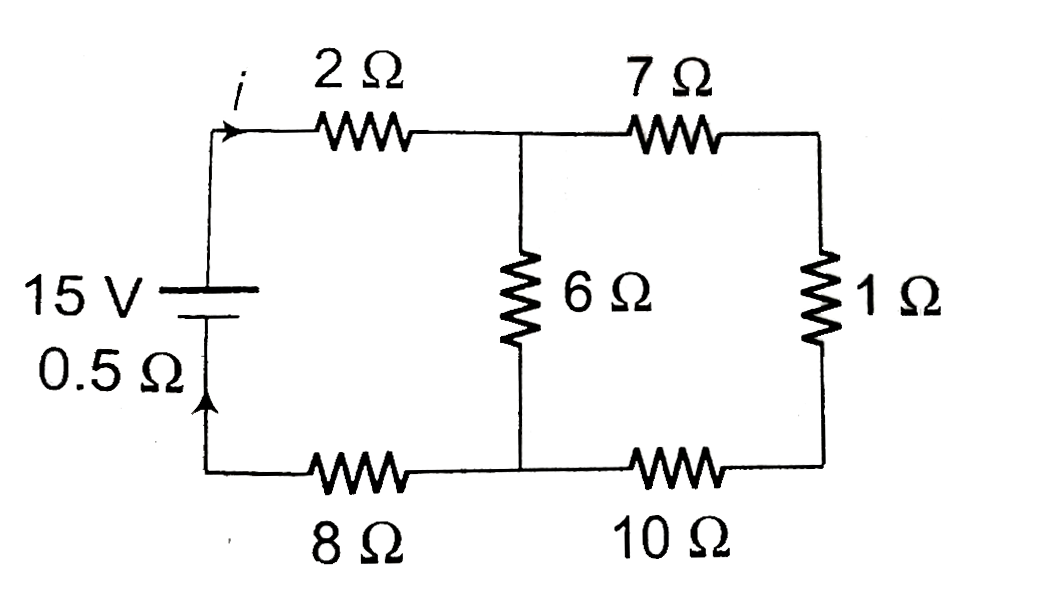 A278WB916WC616WD316W
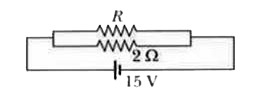
A278WB916WC616WD316WIf in the circuit, power dissipation is 150 W. then R is
 A2ΩB6ΩC5ΩD4Ω
A2ΩB6ΩC5ΩD4Ω
If in the circuit, power dissipation is 150 W, then R is :
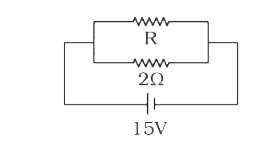
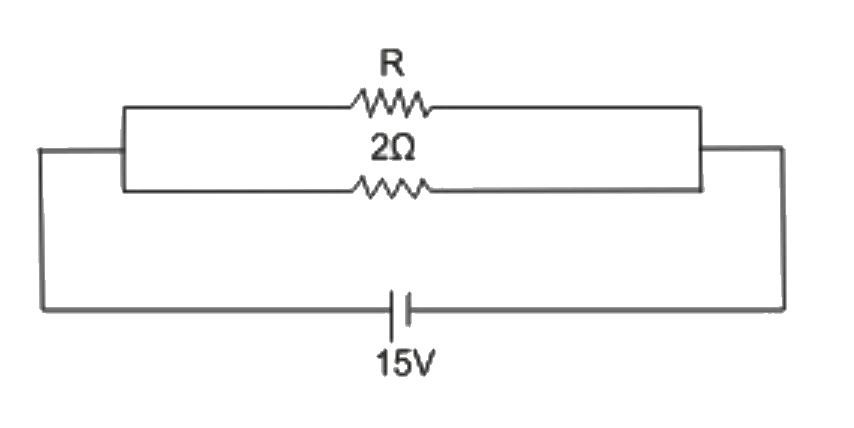
View SolutionIf in the circuit , power dissipation is 150 W, then R is
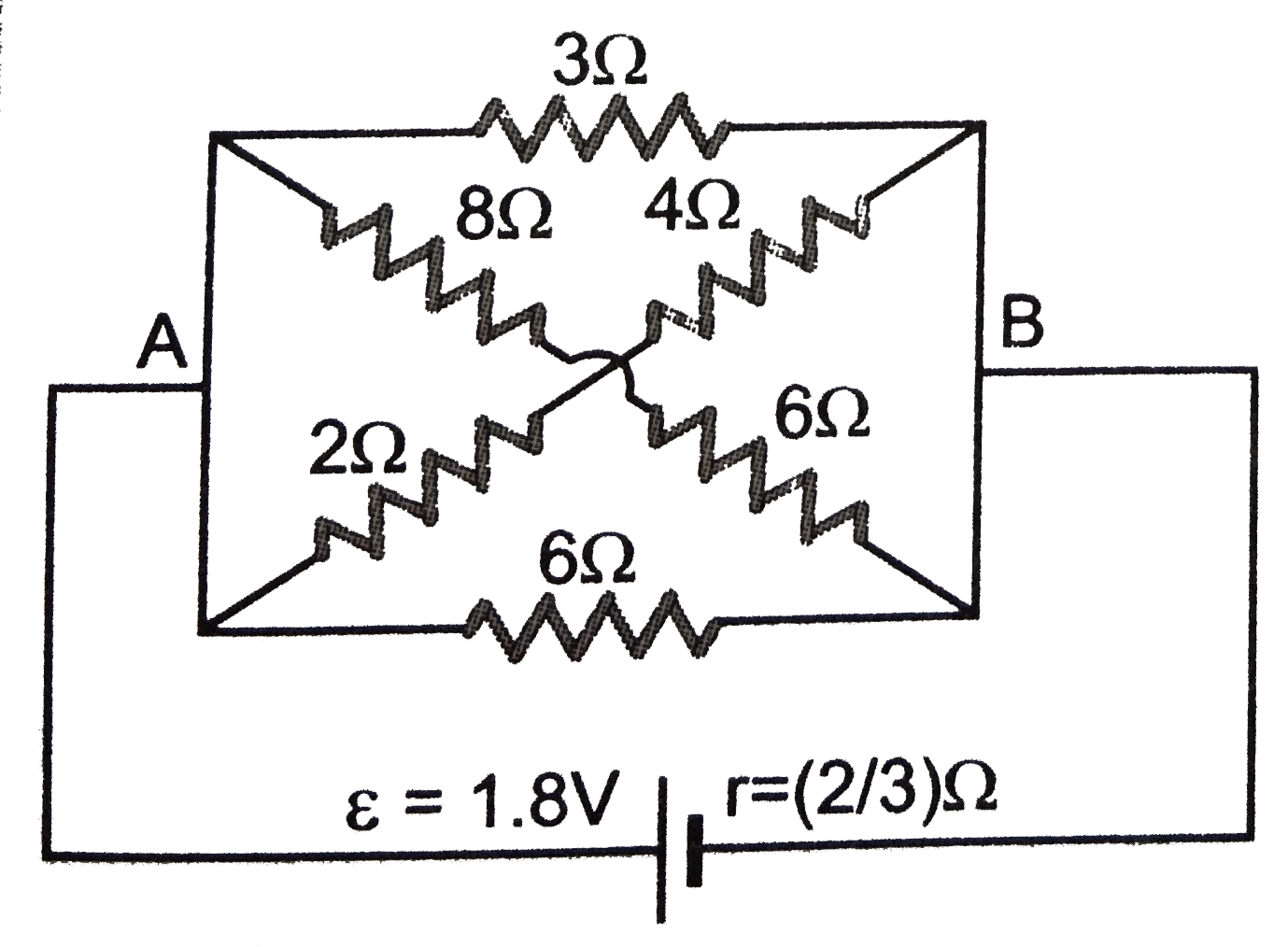
View SolutionThe emf of the cell is 1.8 V and internal resistance is 2/3Ω, calculate the current in the 3Oemga resistance and the power dissipated in the whole circuit.
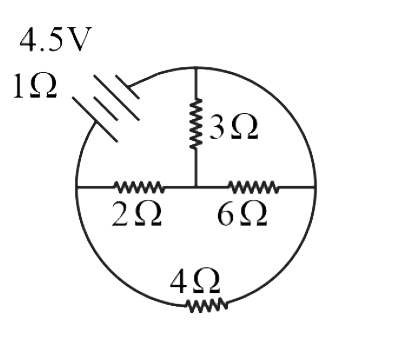
View SolutionThe power dissipated (in watt) in 3Ω resistance in the following circuit is
View SolutionResistors P,Q,andR in the circuit have equal resistances. If the battery is supplying a total power of 12W , what is the power dissipated as heat in resistors R?
View Solution