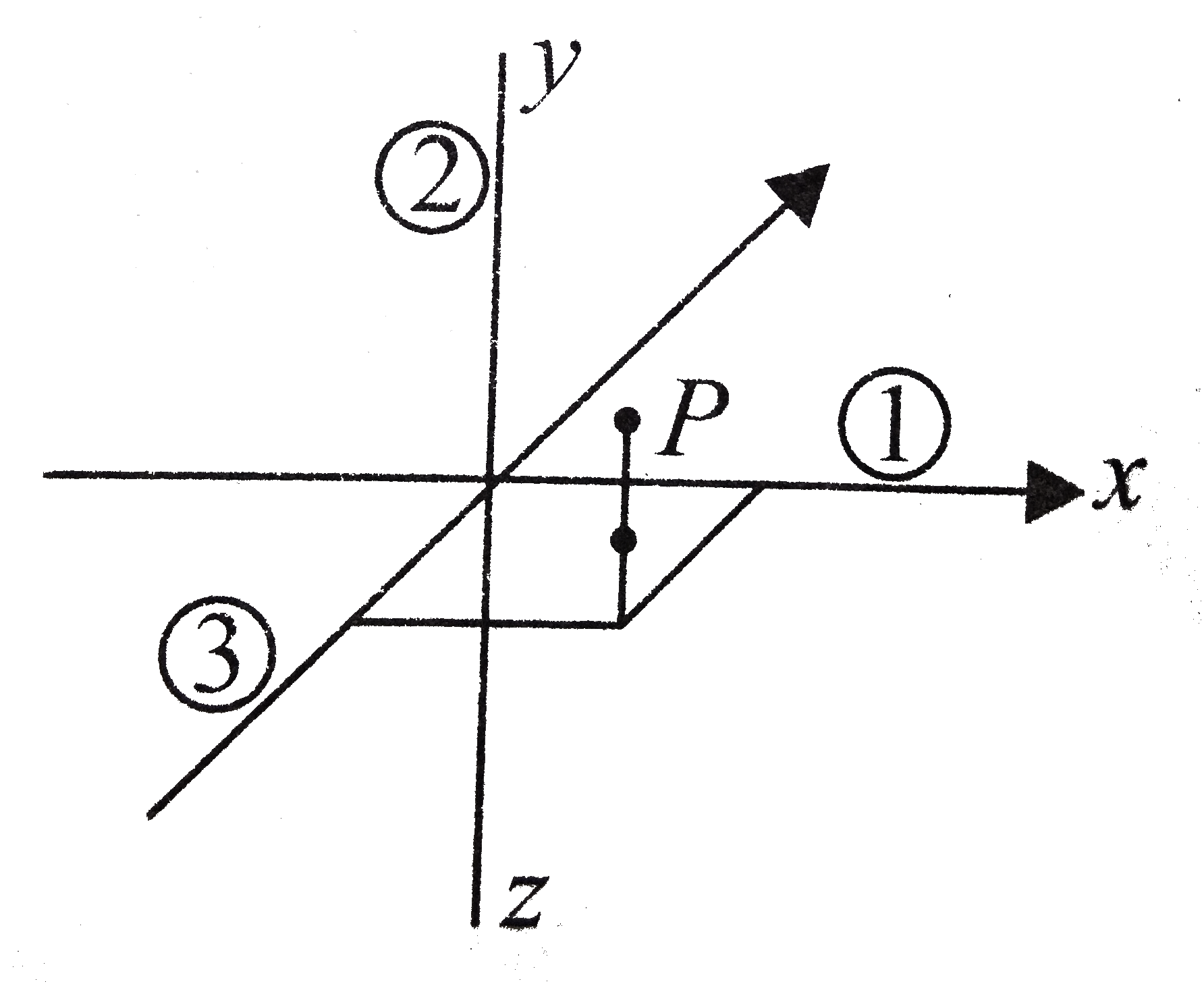
Find the electric field vector at P (a,a,a) due to three infinitely long lines of charges along the x-, y- and z-axis, respectively. The charge density i.e. charge per unit length of each wire is (lamba),
λ3πε0a(ˆi+ˆj+ˆk)
λ2πε0a(ˆi+ˆj+ˆk)
λ2√2πε0a(ˆi+ˆj+ˆk)
2λπε0a(ˆi+ˆj+ˆk)
The correct Answer is:B
Let us consider the electric field due to wire (3) only

→E3=Eˆu
=λ2πε0(a2+a2)1/2(ˆicos45∘+ˆj45∘)
λ2√2πε0a1√2(ˆi+ˆj)
λ4πε0a(ˆi+ˆj)
Similarly, electric field due to wires (1) and (2)
−→E1=λ4πε0a(ˆj+ˆk) and −→E2=λ4πε0a(ˆi+ˆk)
→Enet=→E1+→E2+→E3=λ4πε0a(ˆi+ˆj+ˆk).
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Multiple Correct
Explore 8 Videos
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Comprehension
Explore 25 Videos
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Subjective
Explore 32 Videos
- COMMUNICATION SYSTEMBook:CENGAGE PHYSICSChapter:COMMUNICATION SYSTEMExercise:QUESTION BANK
Explore 19 Videos
- Current ElectricityBook:CENGAGE PHYSICSChapter:Current ElectricityExercise:QUESTION BANK
Explore 40 Videos
Similar Questions
Knowledge Check
The electric field vector at point P(a,a,a) due to three uniformly charged infinite wires 1, 2 and 3 kept the x, y and z - axes, respectively as shown in the shown in figure is (Charge unit length of each wire is λ)
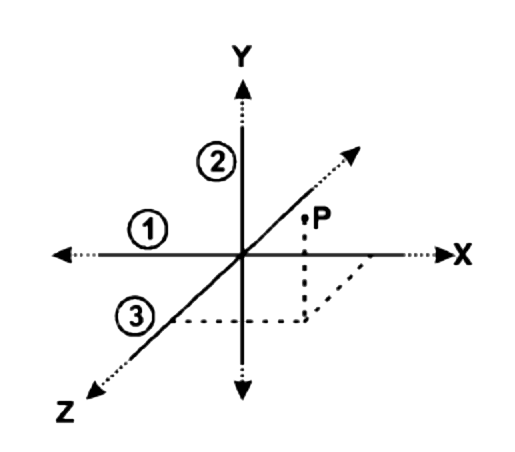 Aλ3πε0a(ˆi+ˆj+ˆk)Bλ2πε0a(ˆi+ˆj+ˆk)Cλ2√2πε0a(ˆi+ˆj+ˆk)D√2λπε0a(ˆi+ˆj+ˆk)
Aλ3πε0a(ˆi+ˆj+ˆk)Bλ2πε0a(ˆi+ˆj+ˆk)Cλ2√2πε0a(ˆi+ˆj+ˆk)D√2λπε0a(ˆi+ˆj+ˆk)The electric field intensity due to a thin infinity long straight wire of uniform linear charge density λ at O is-
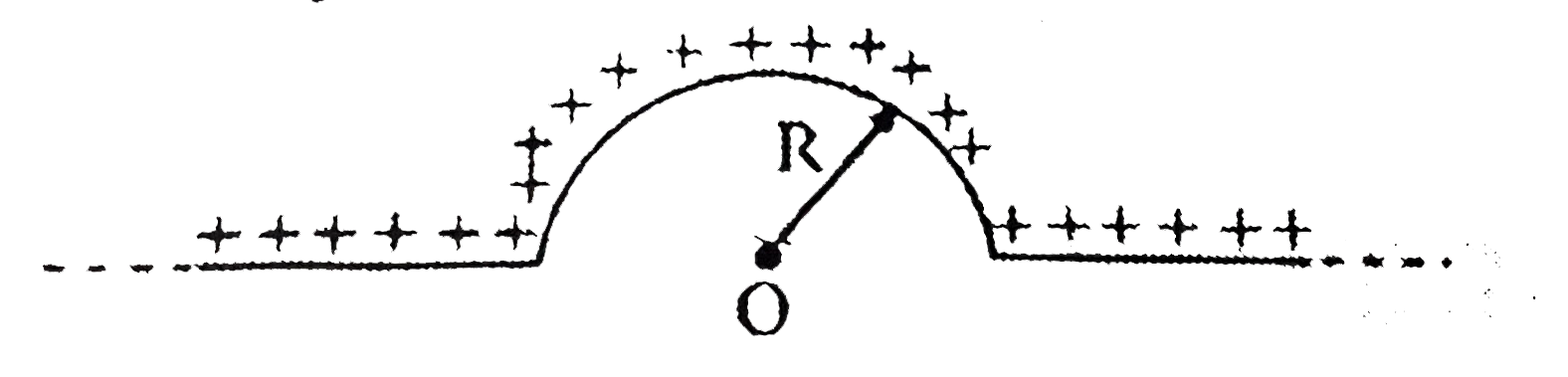 Aλ2πε0RBλ√22πε0RCλ√52πε0RDzero
Aλ2πε0RBλ√22πε0RCλ√52πε0RDzeroThe diagram shows three infinitely long uniform line charges placed on the X,Y and Z axis . The word done in moving a unit positive charge from (1,1,1) to (0 , 1,1,) is equal to
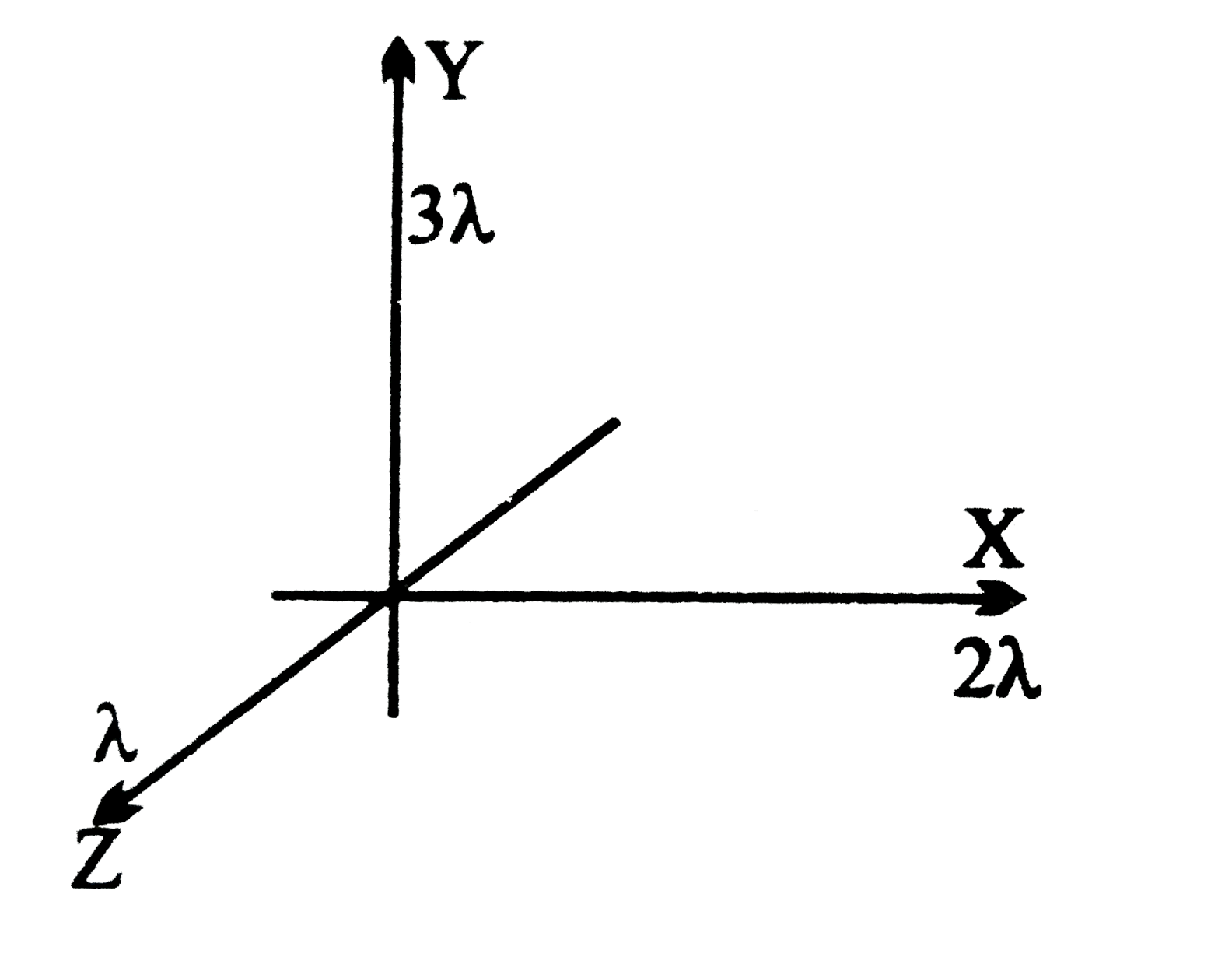 .Aλln22πɛ0B(λln2)/πɛ0C(3λlln2)/2πɛ0DNone
.Aλln22πɛ0B(λln2)/πɛ0C(3λlln2)/2πɛ0DNone
Find the electric field due to an infinitely long cylindrical charge distribution of radius R and having linear charge density λ at a distance half of the radius from its axis.
View SolutionCalculate the potential due to a thin charged rod of length L at the points along and perpendicular to the length. Charge per unit length on the rod is λ.
View SolutionThe electric field at a distance r from a long wire having charge per unit length λ is
View SolutionElectric field intensity at a point due to an infinite sheet of charge having surface charge density σ is E.If sheet were conducting electric intensity would be
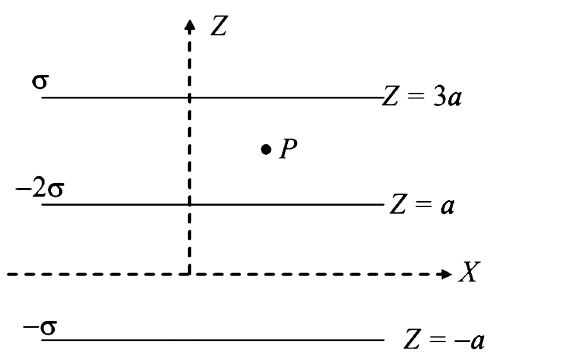
View SolutionThree infinitely long charge sheets are placed as shown in figure. The electric field at point P is
View Solution

