A steel bolt of cross-sectional area $A_{b} = 5 \times 10^{- 5} m^{2}$ is passed through a cylindrical tube made of aluminium. Cross-sectional area of the tube material is $A_{t} = 10^{- 4} m^{2}$ and its length is $l = 50 c m$ . The bolt is just taut so that there is no stress in the bolt and temperature of the assembly increases through $△ θ = 10^{\circ} C$ . Given, coefficient of linear thermal expansion of steel, $α_{b} = 10^{- 5} /^{\circ} C$ .
$A steel bolt of cross-sectional area A(b) = 5 xx 10^(-5) m^(2) is passed through a cylindrical tube made of aluminium. Cross-sectional area of the tube material is A(t)= 10^(-4) m^(2) and its length is l = 50 cm. The bolt is just taut so that there is no stress in the bolt and temperature of the assembly increases through /\theta=10^@C. Given, coefficient of linear thermal expansion of steel, alpha(b) = 10^(-5)//^@C. 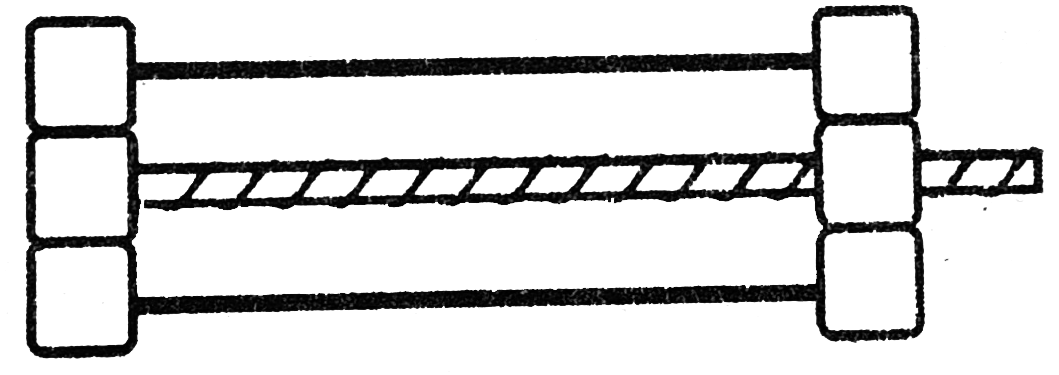Young's modulus of steel Y(b)=2xx10^(11)N//m^(2) Young's modulus of Al, Y(t)=10^(11) N//m^(2), coefficient of linear thermal expansion of Al alpha(t)=2xx10^(-5)//^@C The compressive stress in tube is$
Young's modulus of steel $Y_{b} = 2 \times 10^{11} N / m^{2}$
Young's modulus of $A l, Y_{t} = 10^{11} N / m^{2}$ , coefficient of linear thermal expansion of $A l$ alpha_(t)=2xx10^(-5)//^@C
The compressive stress in tube is

$5 \times 10^{6} N / m^{2}$

$10^{5} N / m^{2}$

$10^{8} N / m^{2}$

$10^{3} N / m^{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Compressive stress in tube
$σ_{t} = E_{t} Y_{t} = 5 \times 10^{6} N / m^{2}$

Updated on:21/07/2023

Class 11 PHYSICS PROPERTIES OF SOLIDS AND FLUIDS

Topper's Solved these Questions

Knowledge Check

A steel bolt of cross-sectional area $A_{b} = 5 \times 10^{- 5} m^{2}$ is passed through a cylindrical tube made of aluminium. Cross-sectional area of the tube material is $A_{t} = 10^{- 4} m^{2}$ and its length is $l = 50 c m$ . The bolt is just taut so that there is no stress in the bolt and temperature of the assembly increases through $△ θ = 10^{\circ} C$ . Given, coefficient of linear thermal expansion of steel, $α_{b} = 10^{- 5} /^{\circ} C$ .

Young's modulus of steel $Y_{b} = 2 \times 10^{11} N / m^{2}$
Young's modulus of $A l, Y_{t} = 10^{11} N / m^{2}$ , coefficient of linear thermal expansion of $A l α_{t} = 2 \times 10^{- 5} /^{\circ} C$
The compressive strain in tube is
A $10^{- 4}$
B $5 \times 10^{- 5}$
C $2 \times 10^{- 3}$
D $10^{- 6}$
A steel bolt of cross-sectional area $A_{b} = 5 \times 10^{- 5} m^{2}$ is passed through a cylindrical tube made of aluminium. Cross-sectional area of the tube material is $A_{t} = 10^{- 4} m^{2}$ and its length is $l = 50 c m$ . The bolt is just taut so that there is no stress in the bolt and temperature of the assembly increases through $△ θ = 10^{\circ} C$ . Given, coefficient of linear thermal expansion of steel, $α_{b} = 10^{- 5} /^{\circ} C$ .

Young's modulus of steel $Y_{b} = 2 \times 10^{11} N / m^{2}$
Young's modulus of $A l, Y_{t} = 10^{11} N / m^{2}$ , coefficient of linear thermal expansion of $A l α_{t} = 2 \times 10^{- 5} /^{\circ} C$
The tensile stress in bolt is
A $10^{4} N / m^{2}$
B $10^{7} N / m^{2}$
C $2 \times 10^{8} N / m^{2}$
D $10^{10} N / m^{2}$
A steel wire of length $4.7 m$ and cross-sectional area $3 \times 10^{- 6} m^{2}$ stretches by the same amount as a copper wire of length $3.5 m$ and cross-sectional area of $4 \times 10^{- 6} m^{2}$ under a given load. The ratio of Young's modulus of steel to that of copper is
A $1.8$
B $3.6$
C $0.6$
D $8.7$