Question

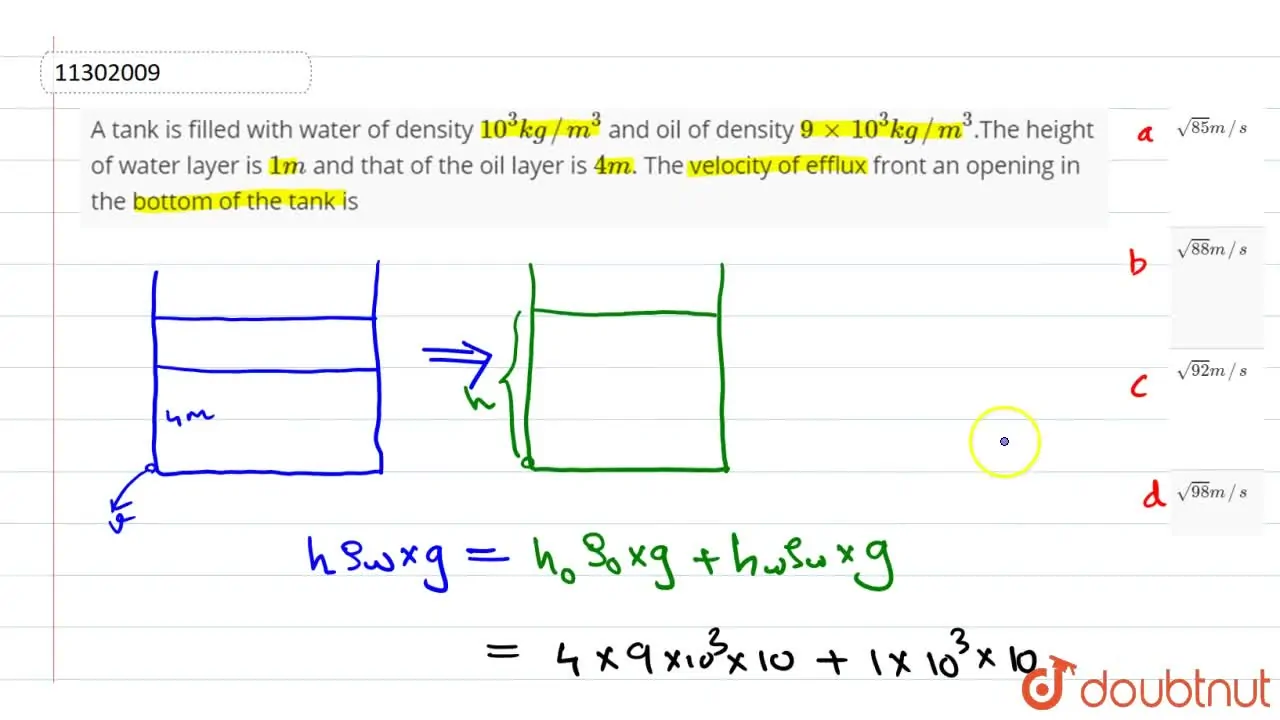
A tank is filled with water of density ${10}^{3}kg/m^3$ and oil of density $9\times {10}^{3}kg/m^3$.The height of water layer is $1m$ and that of the oil layer is $4M$. The velocity of efflux front an opening in the bottom of the tank is

Options

A. `sqrt(85)m//s`
B. `sqrt(88)m//s`
C. `sqrt(92)m//s`
D. `sqrt(98)m//s`

Answer

C

Explanation

To determine the velocity of efflux from an opening at the bottom of the tank, we can use Torricelli's theorem, which states that the speed of efflux $$v$$ of a fluid under the force of gravity through an opening is given by:

$$v=\sqrt{2gh}$$

where:
- $$g$$ is the acceleration due to gravity (approximately $$9.81{\text{m/s}}^2$$),
- $$h$$ is the effective height of the fluid column above the opening.

1. Identify the heights of the fluid layers:
- The height of the water layer \( h_w = 1 \, \text{m} \).
- The height of the oil layer \( h_o = 4 \, \text{m} \).

2. Calculate the effective height \( h \):
- The effective height \( h \) for the fluid column above the opening is the total height of the oil plus the height of the water, since the water is below the oil.
- Therefore, the total height \( h \) is:
\( h = h_o + h_w = 4 \, \text{m} + 1 \, \text{m} = 5 \, \text{m} \)

3. Substitute the values into Torricelli's theorem:
- Using \( g = 9.81 \, \text{m/s}^2 \) and \( h = 5 \, \text{m} \):
\( v = \sqrt{2gh} = \sqrt{2 \times 9.81 \, \text{m/s}^2 \times 5 \, \text{m}} \)

4. Calculate the value:
- First, calculate \( 2gh \):
\( 2gh = 2 \times 9.81 \times 5 = 98.1 \, \text{m}^2/\text{s}^2 \)
- Now, take the square root:
\( v = \sqrt{98.1} \approx 9.9 \, \text{m/s} \)

5. Final Answer:
- The velocity of efflux from the opening at the bottom of the tank is approximately \( 9.9 \, \text{m/s} \).