A block of mass 4kg pressed againest a rought wall by two perpendicular horizontal forces F1andF2as shown in figure Coefficient of static friction between the block and wall is 0.6 and that of kinetic friction is 0.5
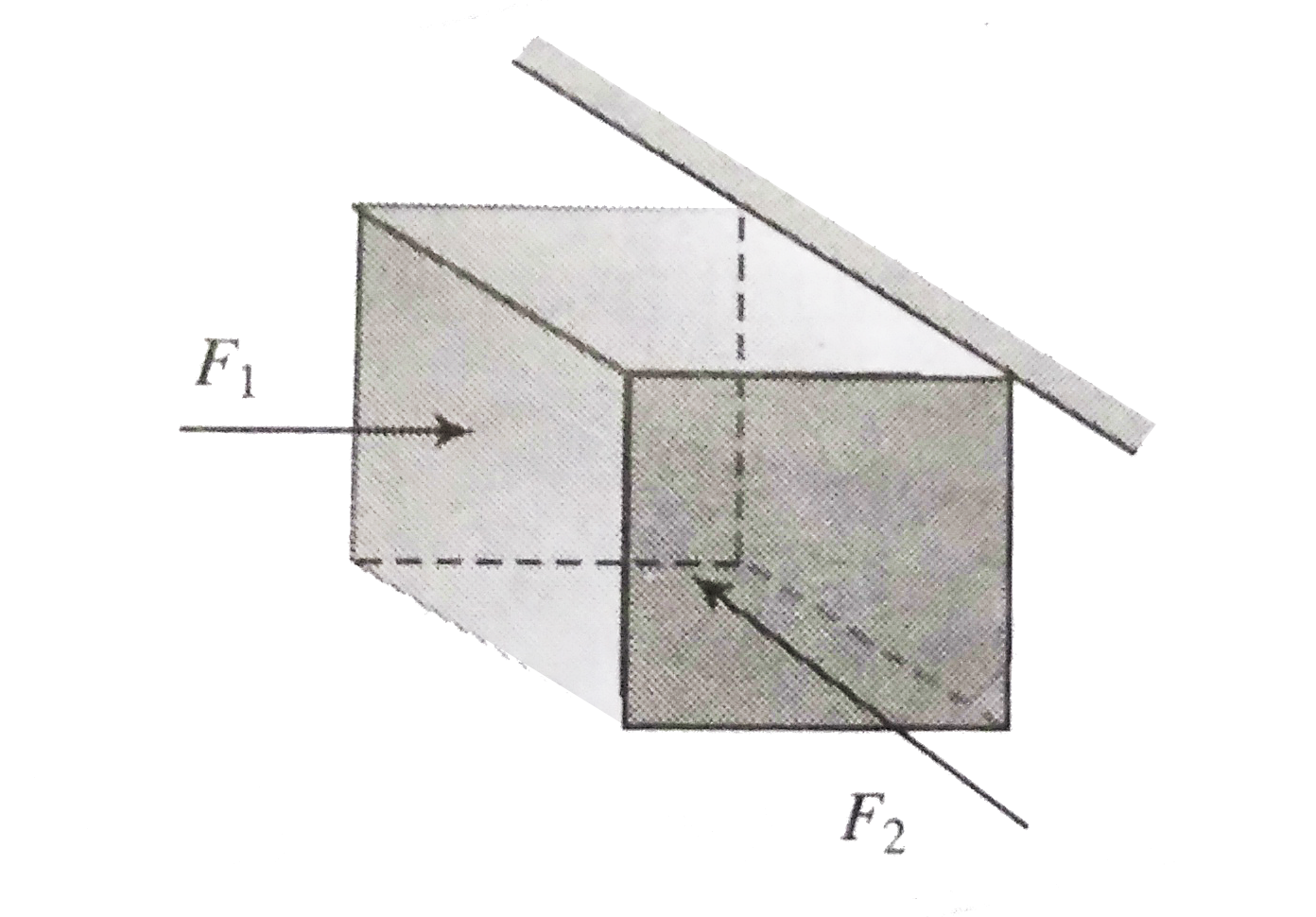
For the data of Q 28 ,Find the magnitude of acceleration of the block
Zero
22.5ms−2
26.925ms−2
9.175ms−2
The correct Answer is:d
Acceleration of block=107.7−754=8.175ms−2
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer
6 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - True And False
4 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Multiple Correct
2 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A block of mass 1kg is pressed against a wall by applying a horizontal force of 10N on the block. If the coefficient of friction between the block and the wall is 0.5 ,magnitude of the frictional force acting on the block is
View SolutionA block of mass m is supported on a rough wall by applying a force in figure Coefficient of static friction between block and wall is μ
For what range of value of p , the block remain in static equilibrium ?
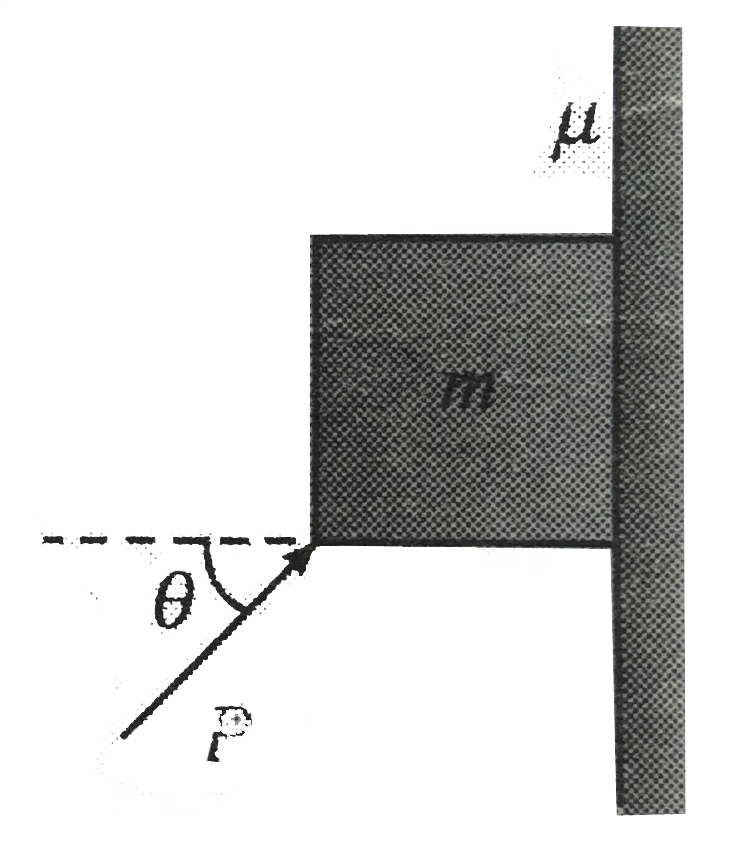
View Solution
Knowledge Check
A block of mass 4kg pressed againest a rought wall by two perpendicular horizontal forces F1andF2as shown in figure Coefficient of static friction between the block and wall is 0.6 and that of kinetic friction is 0.5
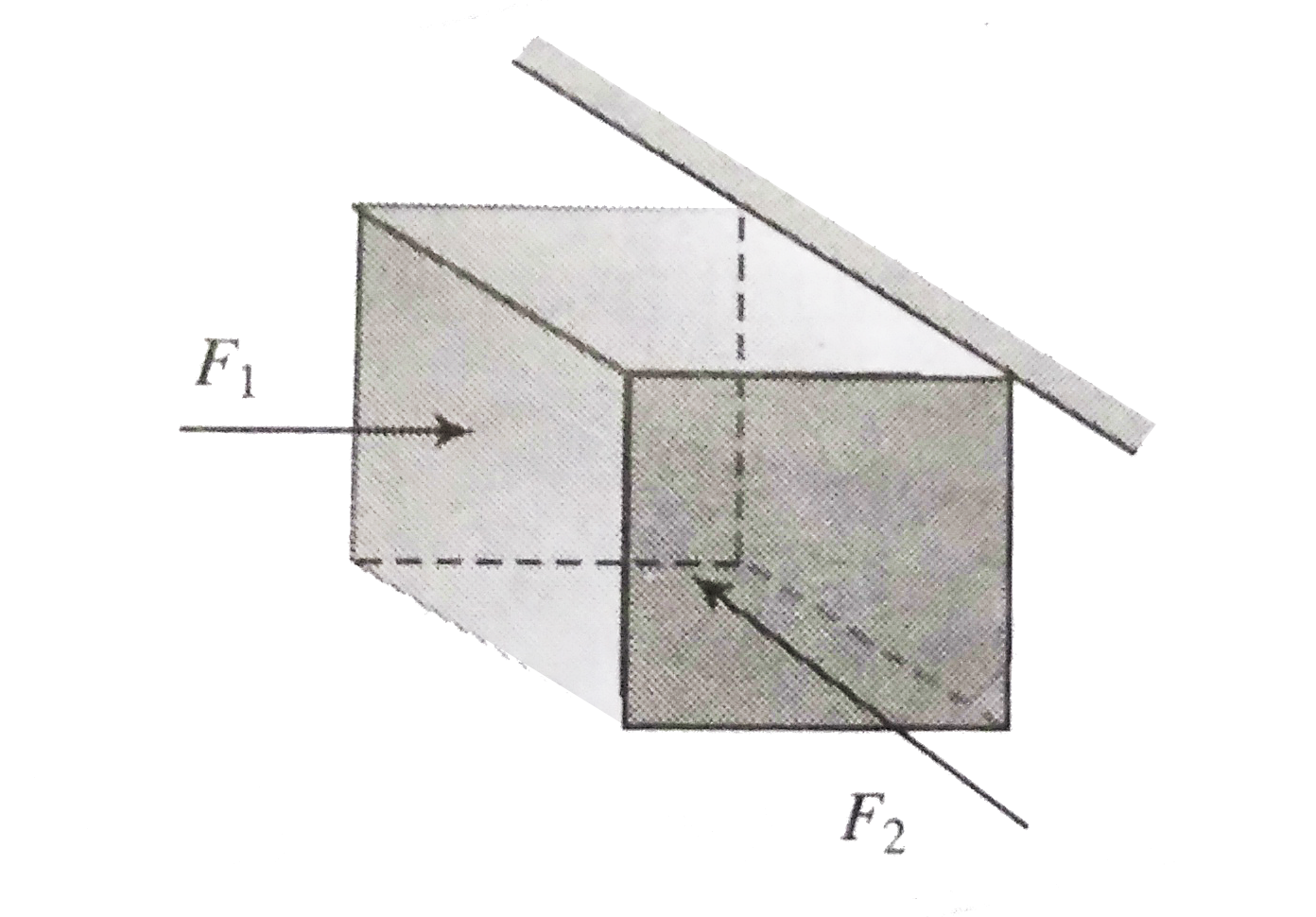
For the above data, what is the acceleration of block?AZeroB1404ms−2,upwardC1804ms−2,upwardD107.74ms−2at an tan−1(25) with the horizontal in the upward directionA block of mass 4kg pressed againest a rought wall by two perpendicular horizontal forces F1andF2as shown in figure Coefficient of static friction between the block and wall is 0.6 and that of kinetic friction is 0.5
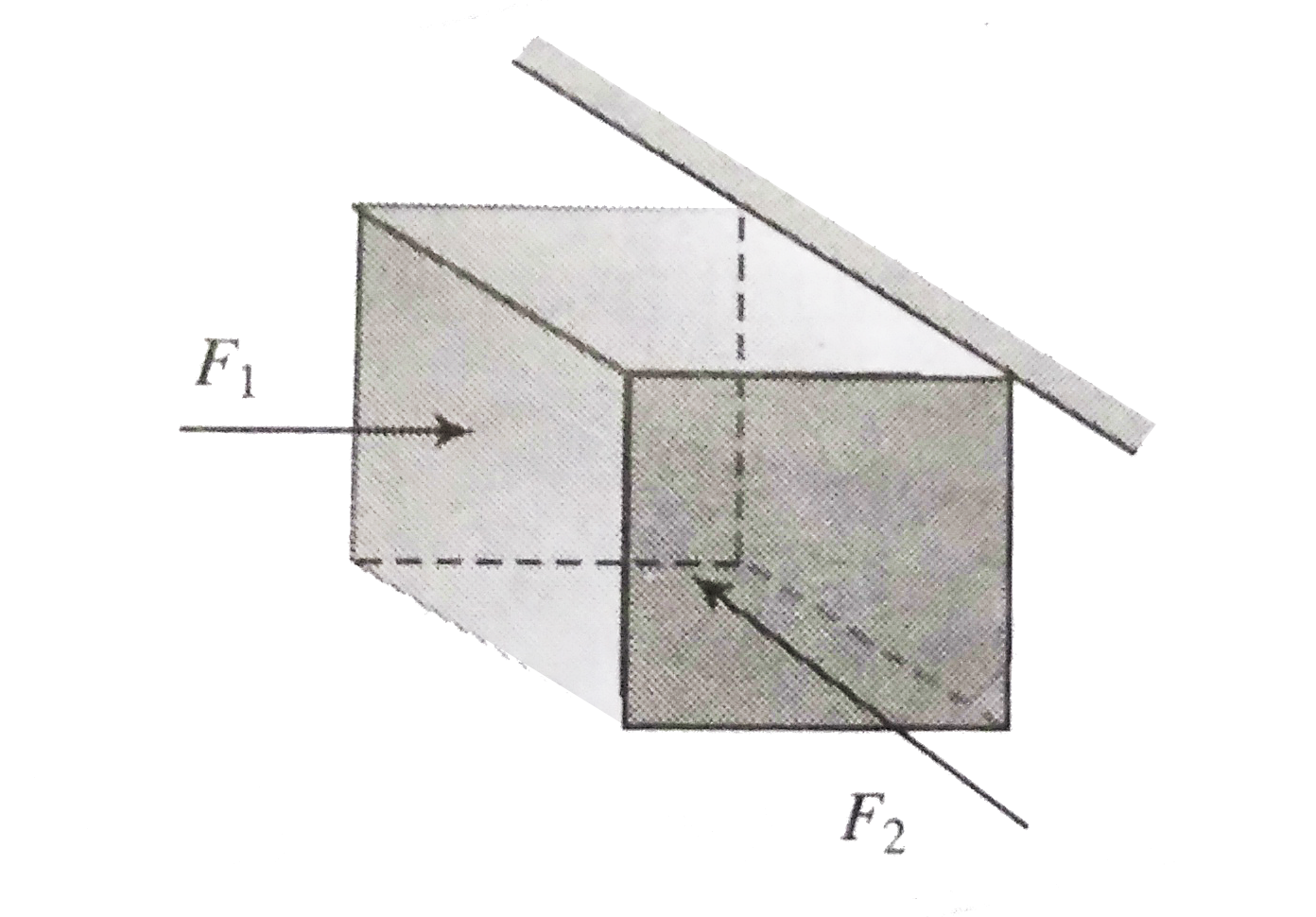
For F1=150NandF2=100N find the direction and magnitude of friction acting on the blockA90N making an angle of tan−1(25) with the horizontal in the upward directionB75N making an angle of tan−1(25) with the horizontal in the upward directionC107.7N making an angle of tan−1(25) with the horizontal in the upward directionDZeroA block of mass 4kg pressed againest a rought wall by two perpendicular horizontal forces F1andF2as shown in figure Coefficient of static friction between the block and wall is 0.6 and that of kinetic friction is 0.5
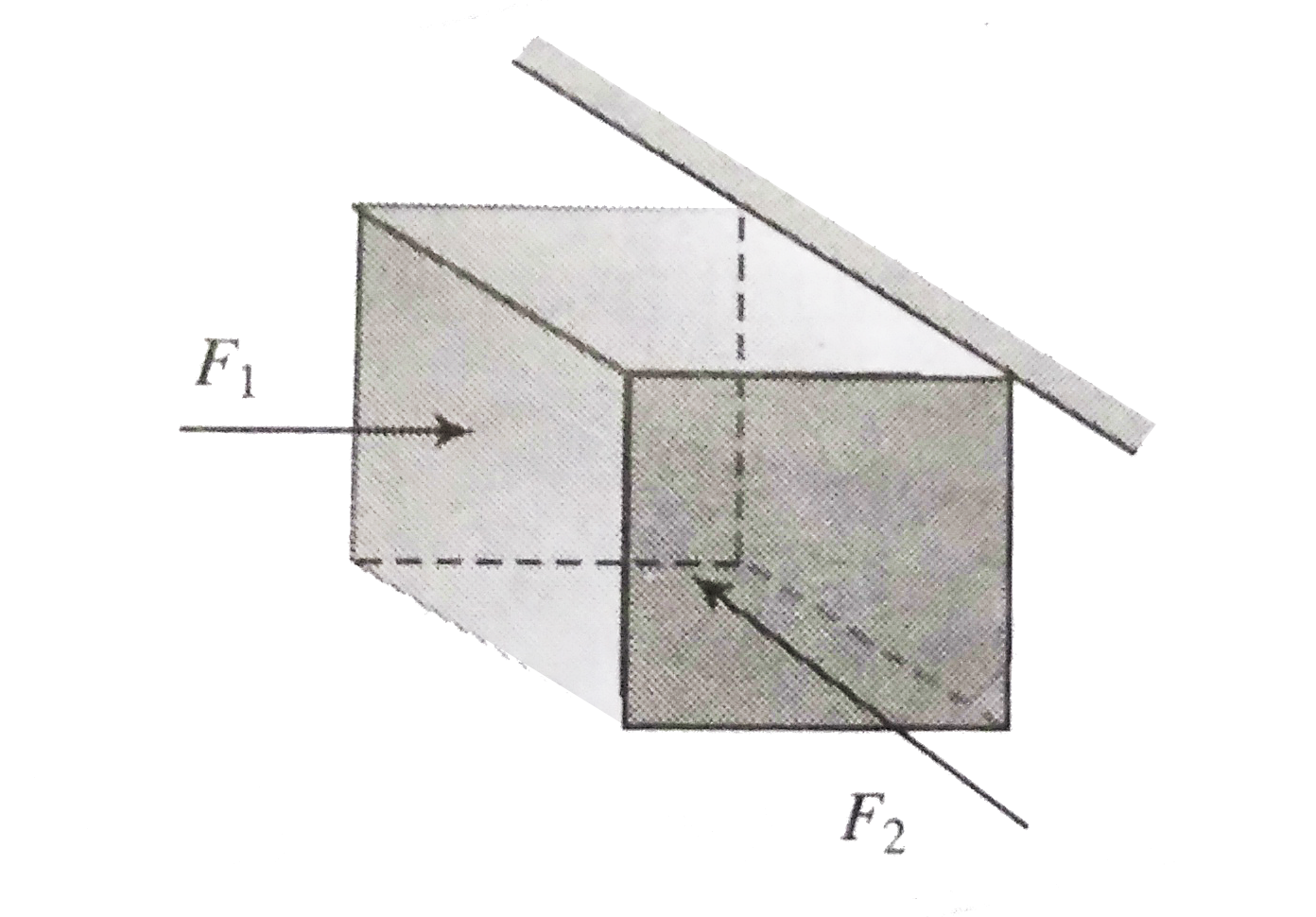
For F1=300NandF2=100N find the direction and magnitude of friction force acting on the blockA180N vertically upwardB40N vertically upwardC107.7N making an angle of tan−1(25) will the horizontal the upward directionD91.6N making an angle of tan−1(25) will the horizontal the upward direction
A block of mass m kg is pushed up against a wall by a force P that makes an angle 'θ' with the horizontal as shown in figure . The coefficient of static friction between the block and the wall is μ . Find the minimum value of P that allows the block to remain stationary .
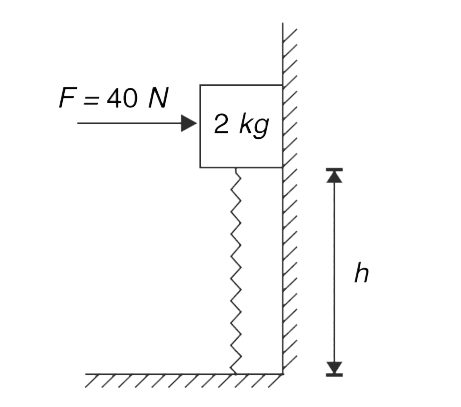
View Solution(i) In the arrangement shown in the figure the coefficient of friction between the 2 kg block and the vertical wall is μ=0.5. A constant horizontal force of 40 N keeps the block pressed against the wall. The spring has a natural length of 1.0 m and its force constant is k=400Nm–1. What should be the height h of the block above the horizontal floor for it to be in equilibrium. The spring is not tied to the block.
(ii) A block of mass M is pressed against a rough vertical wall by applying a force F making an angle of θ with horizontal (as shown in figure). Coefficient of friction between the wall and the block is μ=0.75.
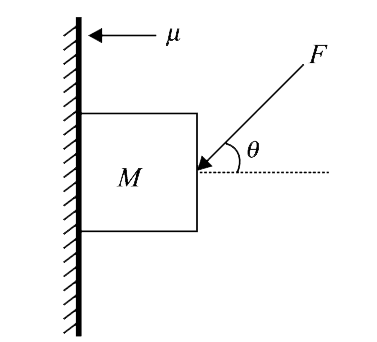
(a) If F = 2 Mg, find the range of values of θ so that the block does not slide
[Take tan37∘=0.75,sin24∘=0.4]
(b) Find the maximum value of θ above which equilibrium is not possible for any magnitude of force F.
View SolutionA block of mass 0.1 kg is held against a wall by applying a horizontal force of 5 N on the block. If the coefficient of friction between the block and the wall is 0.5, then the magnitude of the frictional force acting on the block is
View SolutionA block of mass 0.1 is held against a wall applying a horizontal force of 5N on block. If the coefficient of friction between the block and the wall is 0.5, the magnitude of the frictional force acting on the block is:
View SolutionA force of F = 12.0 N is applied to a 8.00 kg block at a downward angle of 30∘ as shown in the figure.

The coefficient of static friction between the block and the floor is 0.700 and the coefficient of kinetic friction is 0.400. What is the magnitude of the frictional force on the block?
View Solution
