A block of massm1=1kg is horizontally thrown with a velocity of v=10m/s on a statonary long plank of massm2=2kg whose surface has μ=0.5 plank rest on friction surface find the time when the block comes to rest w.r.t plank

Acceleration of block a1=μm1gm1=μg=0.5×10=5ms−2
(toward left)
Acceleration of block a2=μm1gm2=0.5×1×102=52m/s−2
(toward right)
The block comes to rest with respect block when the velocity of both and plane becomes equal
v1=v2⇒v−a1t=a2tor10−5×t=52t
Which gives t=43sec
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.3
27 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Subjective
21 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.1
25 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A block of mass 1kg is horizontal thrown with a velocity of 10ms−1 on a stationary long of mass 2kg whose surface has a μ=0.5. Plane rest on friction surface find the time when m1 comes to rest w.r.t. plane
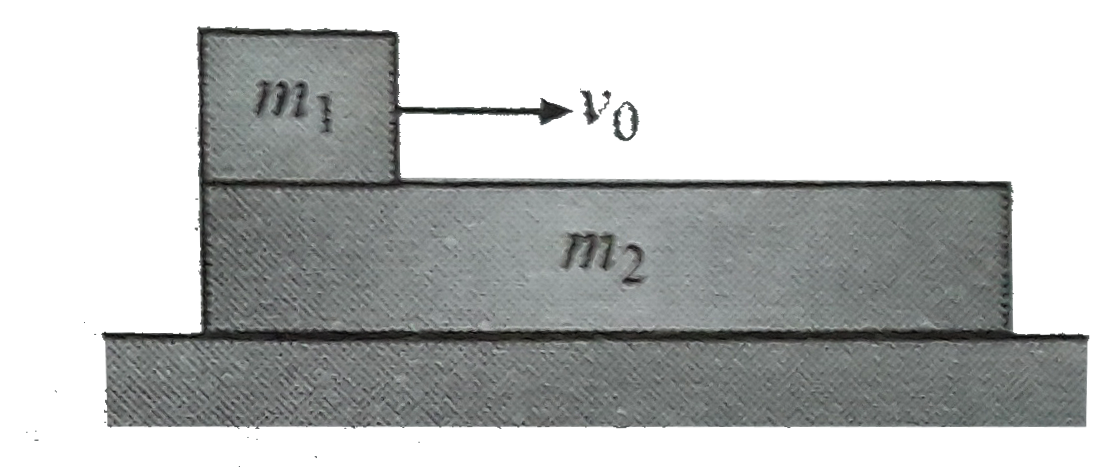
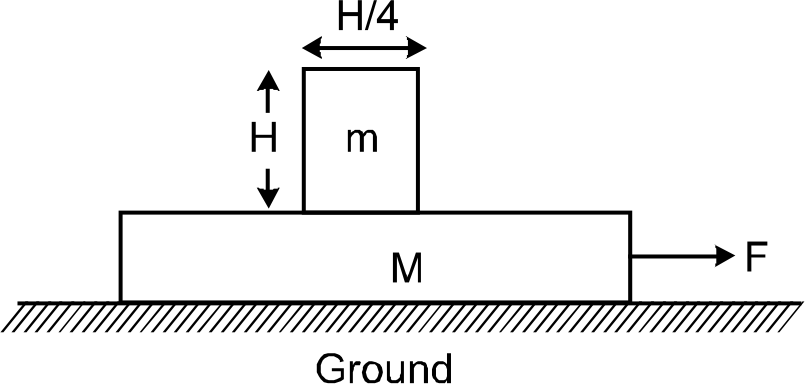
View SolutionA block of mass m=2kg of shown dimensions is placed on a plank of mass M = 6Kg which is placed on smooth horizontal plane. The coefficient of friction between the block and the plank is μ=13. If a horizontal froce F is applied on the plank, then find the maximum value of F (in N) for which the block and the plank move together
View Solution
Knowledge Check
A small block of mass 1 kg starts moving with constant velocity 2 m/s on a smoth long plank of mass 10 kg which is also pulled by a horizontal force F=10t where t is is in seconds and f in newton 's (the initial velocity of the plank is zero)

Relative velocity of plank with to block when acceleration of plank is 4m/s2 wil beAzeroB10 m/sC6 m/sD8 m/sA small block of mass 1 kg starts moving with constant velocity 2 m/s on a smoth long plank of mass 10 kg which is also pulled by a horizontal force F=10t where t is is in seconds and f in newton 's (the initial velocity of the plank is zero)

Displaacement of 1 kg block with respect to plank at the intant when both have same velocity isA443mB4mC83mD2mA small block of mass 1 kg starts moving with constant velocity 2 m/s on a smoth long plank of mass 10 kg which is also pulled by a horizontal force F=10t where t is is in seconds and f in newton 's (the initial velocity of the plank is zero)
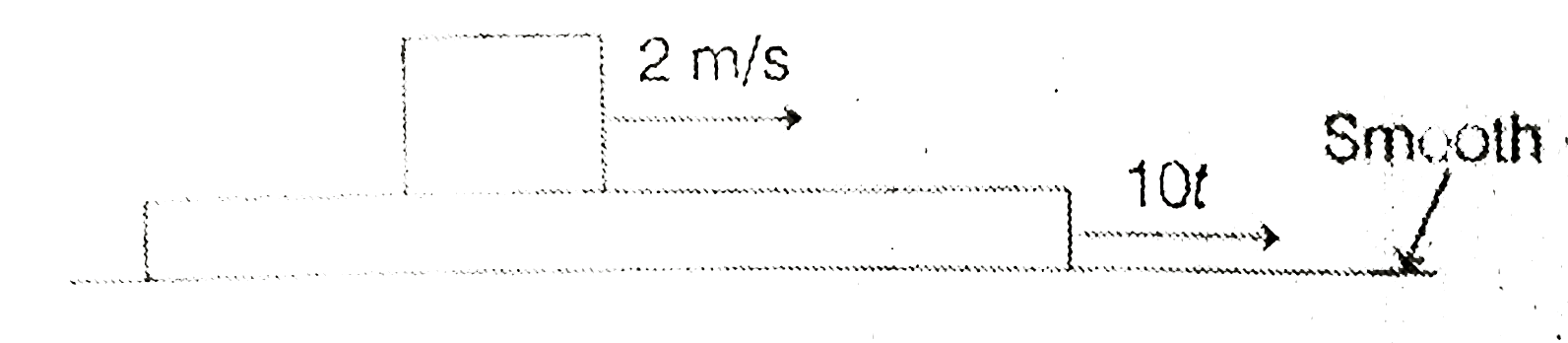
the time (t≠0) at which displacement of block and plank with esspect with repect to ground is same will beA2SB2√3sC3√3sD4s
A plank of mass 10kg rests on a smooth horizontal surface. Two blocks A and B of masses mA=2kgandmB=1kg lies at a distance of 3m on the plank as shown in the distance of 3m on the plank as shown in the figure. The friction coefficient between the blocks and plank are μA=0.3andμB=0.1. Now a force F = 15 N is applied to the plank in horizontal direction. Find the time after which block A collides with B.
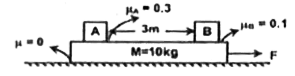
View SolutionA block is thrown with 14ms−1 over a long stationary plank as shown. Beofre the block comes at rest with respect to plank
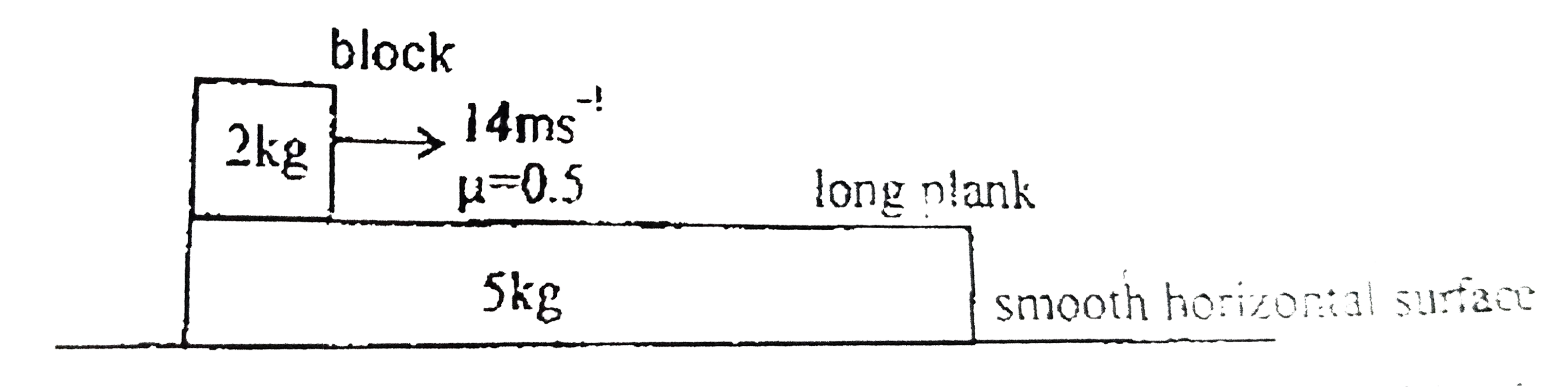
View SolutionA particle of mass m is projected at an angle of 60∘ with a velocity of 20m/s relative to the ground from a plank of same mass m which is placed on smooth surface Initially Plank was at rest The minimum length of the plank for which the ball will fall on the plank itself is (g=10m/s2)

View SolutionA sphere of mass m is given some angular velocity about a horizontal axis through the center, and gently placed on a plank of mass m. The coefficient of friction between the two is μ. The plank rests on a smooth horizontal surface. The intial acceleration of the sphere relative to the plank will be:
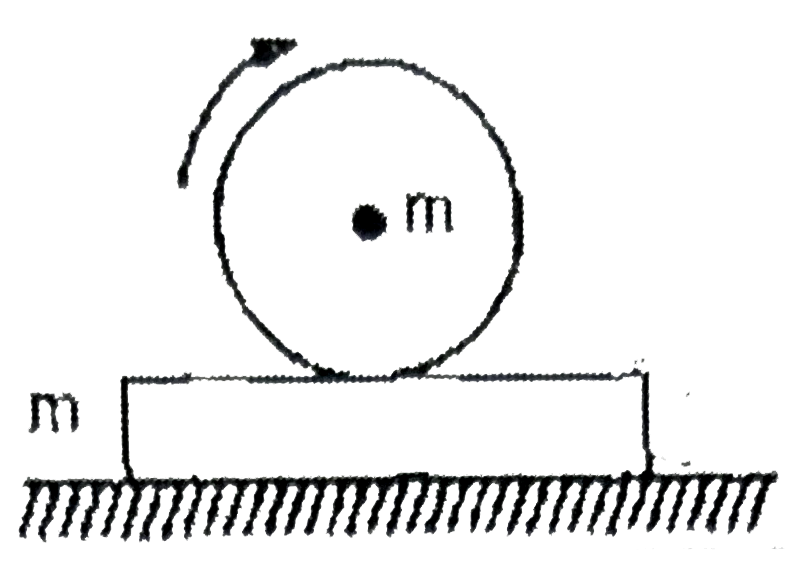
View SolutionA block of mass 2 kg rests on a rough horizontal plank , the coefficient of friction between the plank and block is 0.3. If the plank is pulled horizontally with a constant acceleration of 4m/s2 , the distance moved by the block on the plank in 5 second starting from rest (in m) is : (Take g = 10m/s2)
View Solution
