A plank of mass 10kg rests on a smooth horizontal surface. Two blocks A and B of masses mA=2kgandmB=1kg lies at a distance of 3m on the plank as shown in the distance of 3m on the plank as shown in the figure. The friction coefficient between the blocks and plank are μA=0.3andμB=0.1. Now a force F = 15 N is applied to the plank in horizontal direction. Find the time after which block A collides with B.
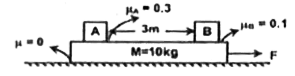
Maximum friction force between the plank (P) and A is FA=μAmAg=6N
Maximum friction force between the plank (P) and B is F8=μBmBg=1N
Let system moves together with acceleration a then a=FmA+mB+M=1513m/s
Now, friction force on ma,fB=mBa=1513N
Friction force on mA,fA=mAa=3013N
As fB>FB⇒ Hence B will not move with plank.
fA<FA⇒ Hence A will move with plank.
aB=FBmB=1m/s2
AP=F−FBmA+M=76m/s2
Now F.B.D. of the plank and A & B are Acceleration of B w.r.t. the plank
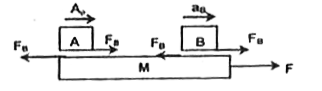
aBP=1−76=−16m/s2
Now LBP=12aBvt2⇒−3=12×(−16)t2
Time of collision t = 6 sec
Alternate
In ground frame, at time of collision:
12Apt2=L+12aBt2⇒12(Ap−aB)t2=L⇒12(76−1)t2=3⇒t=6sec
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - MATRIX MATCH TYPE
4 Videos
- LAWS OF MOTIONBOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - Exercise
15 Videos
- LAWS OF MOTIONBOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - ASSERTION REASONING TYPE
1 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - NUMERICAL BASED QUESTIONS DECIMAL TYPE
5 Videos
- MAGNETICBOOK - FIITJEECHAPTER - MAGNETICEXERCISE - Numerical Based Type
2 Videos
Similar Questions
A wooden plank of mass 20 kg is resting on a smooth horizontal floor. A man of mass 60 kg starts moving from one end of the plank to the other end. The length of the plank is 10 m. Find the displacement of the plank over the floor when the man reaches the other end of the plank.
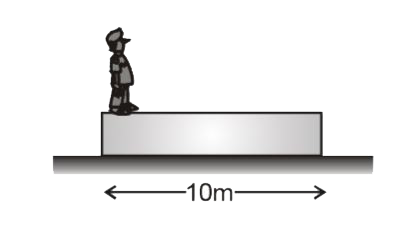
View SolutionA wooden plank of mass 20kg is resting on a smooth horizontal floor. A man of mass 60kg starts moving from one end of the plank to the other end. The length of the plank is 10m. Find the displacement of the plank over the floor when the man reaches the other end of the plank.

View Solution
Knowledge Check
Two block of masses m1 and m2 are connected with a massless unstretched spring and placed over a plank moving with an acceleration 'a' as shown in figure. The coefficient of friction between the blocks and platform is μ
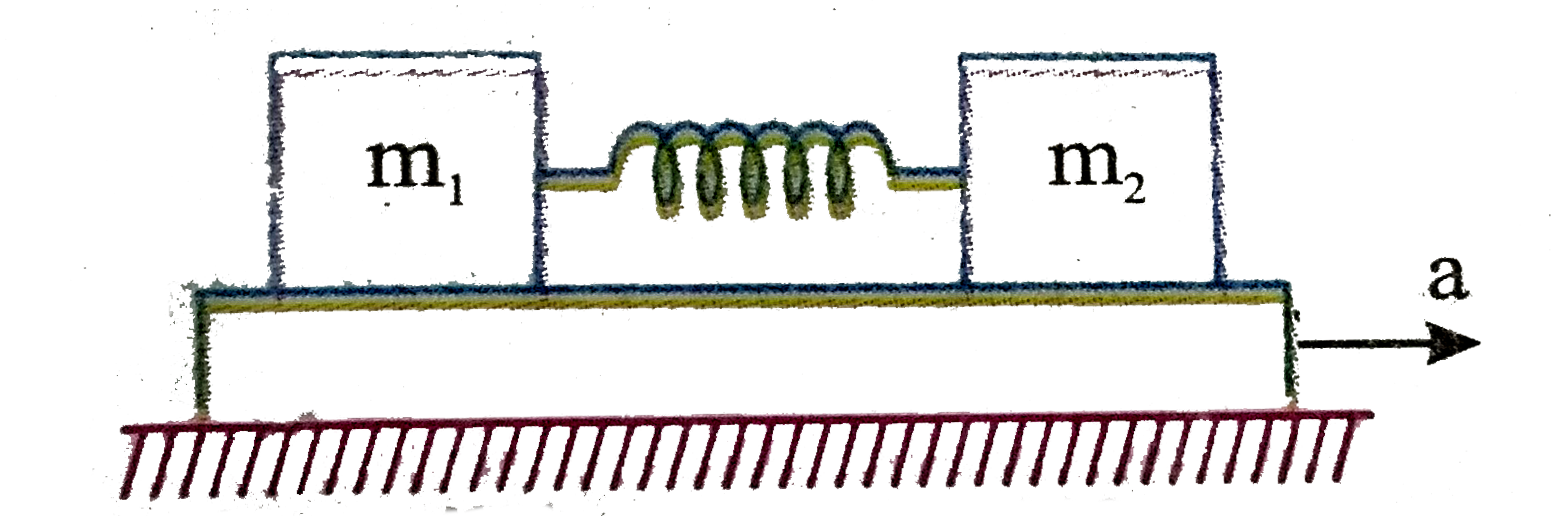 Aspring will be stretched if a>μgBspring will be commpressed if a≤μgCspring will neither be compressed nor be stretched for a<μg only.Dspring will be in its natural length under all conditions.
Aspring will be stretched if a>μgBspring will be commpressed if a≤μgCspring will neither be compressed nor be stretched for a<μg only.Dspring will be in its natural length under all conditions.A plank of mass 10 kg and a block of mass 2 kg are placed on a horizontal plane as shown in the figure. There is no friction between plane and plank. The coefficient of friction between block and plank is 0.5 .A force of 60 N is applied on plank horizontally. In first 2 s the work done by friction on the block is
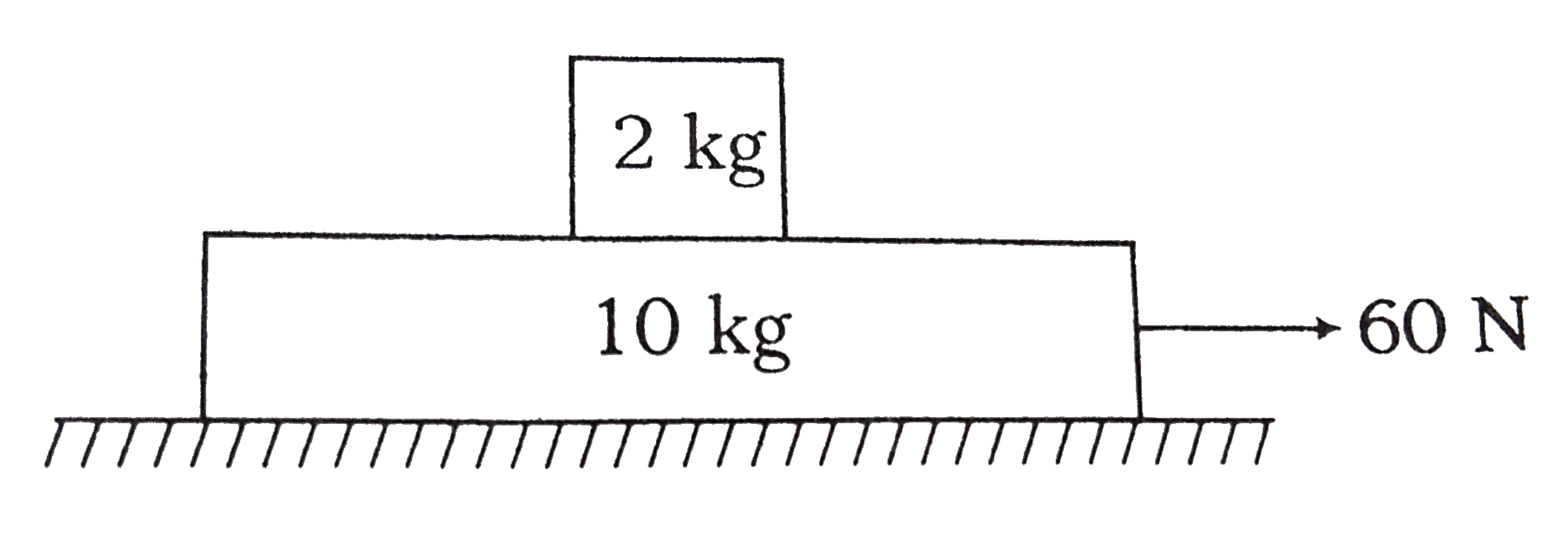 A−100JB100 JCzeroD200 J
A−100JB100 JCzeroD200 JA block of mass m =2 kg is placed on a plank of mass M = 10 kg, which is placed on a smooth horizontal plane as shown in the figure. The coefficient of friction between the block and the plank is μ=13. If a horizontal force F is applied on the plank, then the maximum value of F for which the block and the plank move together is (g=10m/s2)
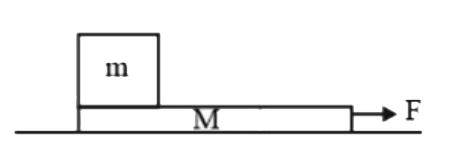 A50 NB40 NC120 ND100 N
A50 NB40 NC120 ND100 N
A thin but very large plank of mass 2 m is placed on a horziontal smooth surface. A solid cylinder of mass m and radius r is given only translational velocity v0 and gently placed on the plank as shown in the figure. The coefficient of kinetic friciton between the plank and the cylinder is μ.
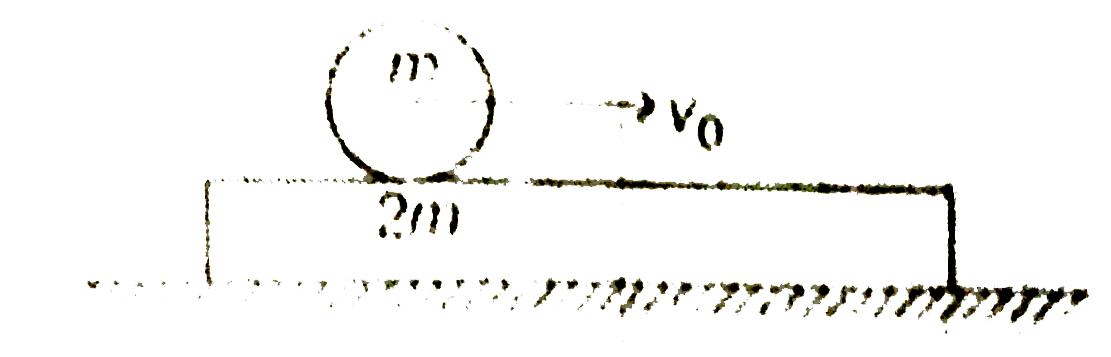
View SolutionA plank of mass 10 kg lies on a smoth horizontal surface .A constant forces F= 400 N is is applied on the plank as shown in the figure .(g=10m/s2)
match the statements from Table -1 with the the values in Table -2 and select the correct answer using the code given below.

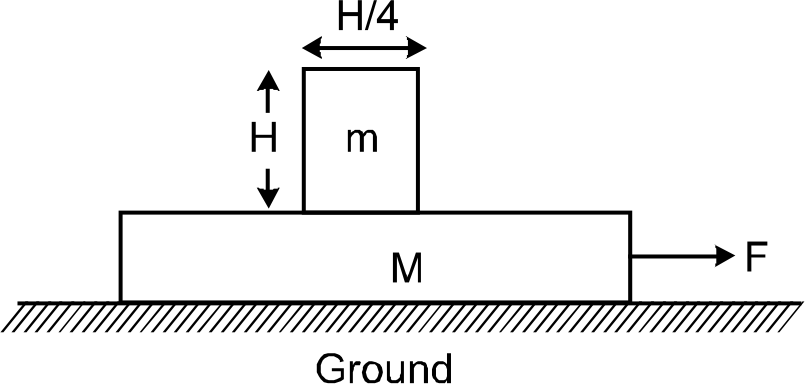
View SolutionA block of mass m=2kg of shown dimensions is placed on a plank of mass M = 6Kg which is placed on smooth horizontal plane. The coefficient of friction between the block and the plank is μ=13. If a horizontal froce F is applied on the plank, then find the maximum value of F (in N) for which the block and the plank move together
View SolutionA small block of mass 1kg is placed over a plank of mass 2kg. The length of the plank is 2 m. coefficient of friction between the block and the plnak is 0.5 and the ground over which plank in placed is smooth. A constant force F=30N is applied on the plank in horizontal direction. the time after which the block will separte from the plank is (g=10m/s2)
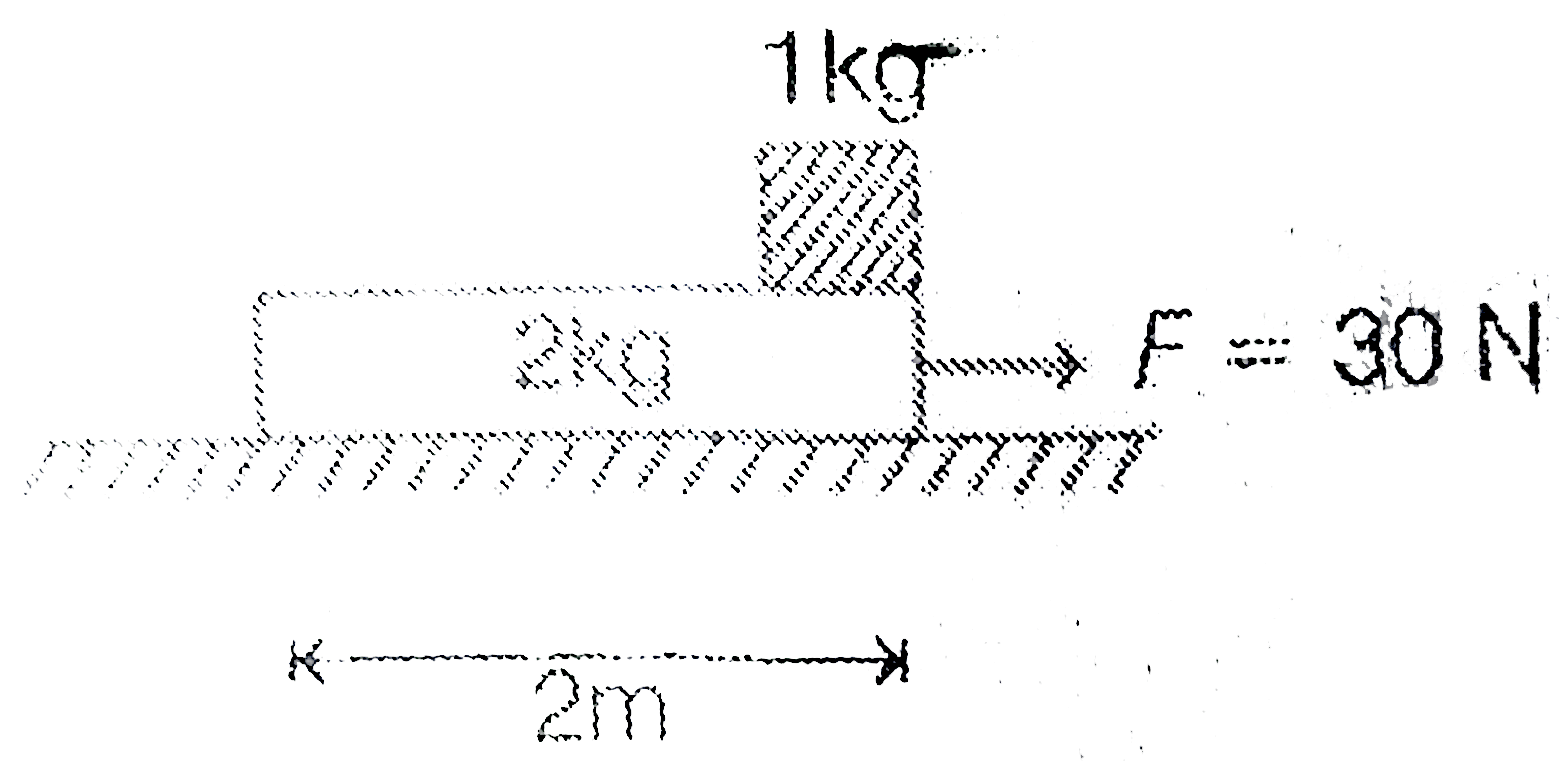
View SolutionA plank A of mass M rests on a smooth horizontal surface over which it can move without friction A cabe B of mass m lies on the plank at one edge .The coefficient of friction between the plank and the cube is μ The size of cube is very small in comparison to the plank.
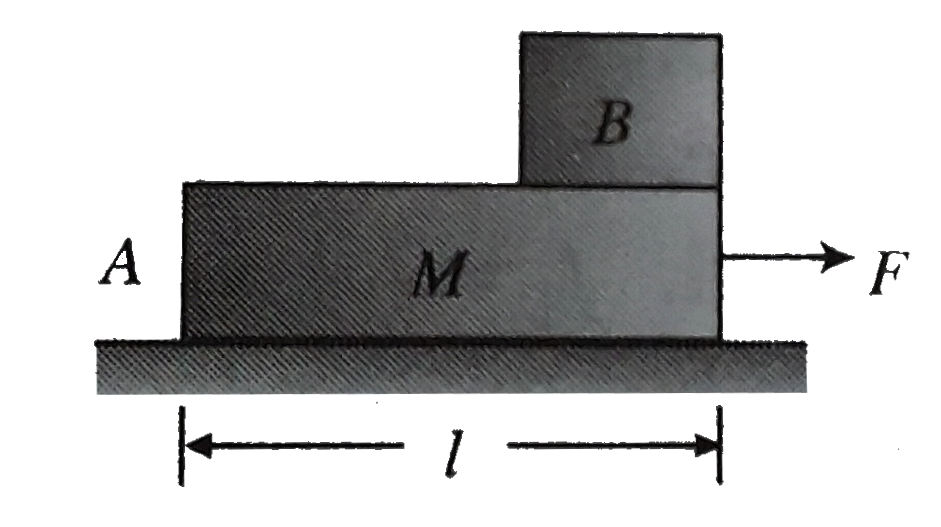
At what force F applied to the plank in the horizontal direction will be cube begin to slide towards the other end of the plank?
View Solution
