A particle of $m = 5 k g$ is momentarily at rest at $x = a t$ t=. It is acted upon by two forces ${\to F}_{1}$ and ${\to F}_{2}$ . $V e c {(F)}_{1} = 70 ˆ j$ N. The direction and manitude of ${\to F}_{2}$ are unknown. The particle experiences a constant acceleration, $\to a$ ,in the direction as shown in (figure) Neglect gravity.

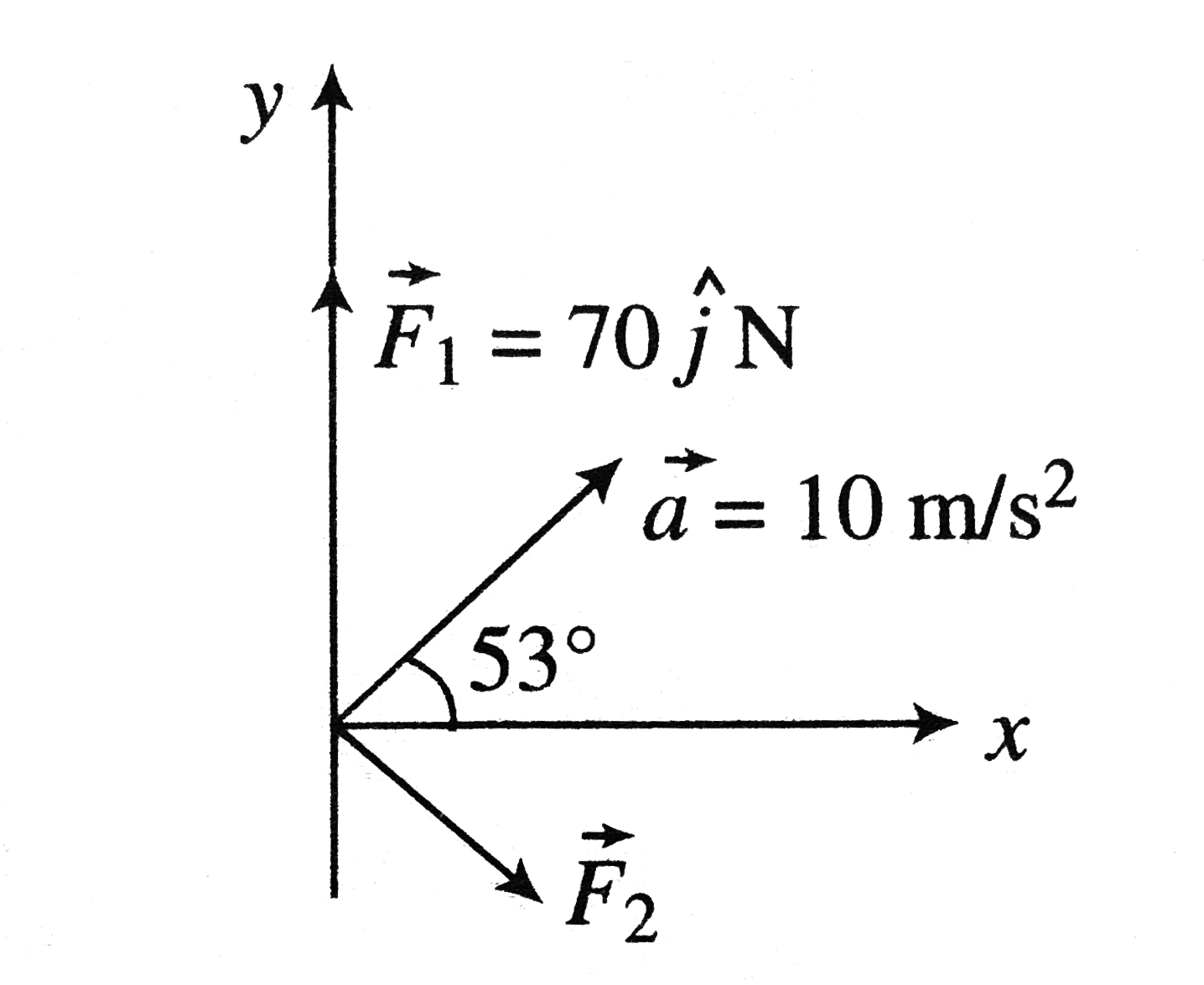
a.Find the missing force ${\to F}_{2}$ .
b. What is the velocity vector of the particle at $t = 10 s$ ?
c. What third force, ${\to F}_{3}$ is required to make the acceleration of the particle zero? Either give magnitude and direction of ${\to F}_{3}$ or its components.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:a. $30 \sqrt{2}$ ; b. $60 ˆ i + 80 ˆ j$ ; c. $30 ˆ i - 40 ˆ j$

${\to F}_{1} + {\to F}_{2} = m . \to a$
$(70 ˆ j + F_{2} ˆ i + F_{2} ˆ j) = 5 (10 x cos 53 ˆ i + 10 sin 53 ˆ j)$
$= 50 (10 \times \frac{3}{5} ˆ i + 10 \times \frac{4}{2} ˆ j)$
By comparing two sides. We get
$F_{2} ˆ i = 30 ˆ i \Rightarrow F_{2} ˆ j = - 30 ˆ j$
$∴ {\to F}_{2} = 30 ˆ i - 30 ˆ j$
$| F_{2} | = \sqrt{{(30)}^{2} + {(30)}^{2}} = 30 \sqrt{2} N$
At $t$ = $10 s$
$v = \to u + a t = 10 (6 ˆ i + 8 ˆ j) = 60 ˆ i + 80 ˆ j$
Acceleration will be zero if a third force makes resultant force =0
$∴ \to F + {\to F}_{2} + \to F + (3) = 0$
or ${\to F}_{3} = - ({\to F}_{1} + {\to F}_{2}) = - (70 ˆ j + 30 ˆ i - 30 ˆ j) = 30 ˆ i - 40 ˆ j$

|

Updated on:21/07/2023

Class 11 PHYSICS VECTOR

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
When force ${\to F}_{1}, {\to F}_{2}, {\to F}_{3}$ are acting on a particle of mass $m$ , the particle remains in equilibrium. If the force ${\to F}_{1}$ is now removed then the acceleration of the particle is :
A ${\to F}_{1} / m$
B ${\to F}_{1} / m$
C ${\to F}_{2} - {\to F}_{3} / m$
D ${\to F}_{2} / m$
Question 2 - Select One
When force $- \to F_{1}, - \to F_{2}, - \to F_{3} \dots... - \to F_{n}$ act on a particle , the particle remains in equilibrium . If $- \to F_{1}$ is now removed then acceleration of the particle is
A $\frac{- \to F_{1}}{m}$
B $- \frac{- \to F_{1}}{m}$
C $- \frac{- \to F_{2} + - \to F_{3} + ..... - \to F_{n} - - \to F_{1}}{m}$
D $\frac{- \to F_{1}}{m}$
Question 3 - Select One
Two forces ${\to F}_{1} = 500 N$ due east and ${\to F}_{2} = 250 N$ due north have their common initial point. ${\to F}_{2} - {\to F}_{1}$ is
A $250 \sqrt{5} N, {tan}^{- 1} (2) W o f N$
B $250 N, {tan}^{- 1} (2) W$ of N
CZero
D $750 N, {tan}^{- 1} (3 / 4) N o f W$

CENGAGE PHYSICS-VECTORS-Exercise Subjective

Home
Ask Doubt
Profile