O is the circumecentre of ΔABCandD is the mid point of BC. If ∠BAC=40∘, then find the value of ∠BOD. Solution in Bengali
D is the mid-point of BC,∴BD=CD
Now, in triangles ΔBODandΔCOD,OB=OC [radii of the same circle]
BD=CDand∠OBD=∠OCD[∴=OC]
∴ΔBOD≅ΔCOD [ by the conditon of S-A-S congruent]
∴∠BOD=∠COD[∴ smiliar angles of congruent triangles]
∴∠BOD=12∠.........(1)[∴∠BOC=∠BOD+∠COD=∠BOD+∠BOD=2∠BOD]
Now,the central angle produced by the are ⌢BC=∠BOC and its angle is circle =∠BAC.
∴∠BOC=2∠BAC[ by theorem-34]
=2×40∘[∴∠BAC=40∘]=80∘
The from (1) we get, ∠BOD=12∠BOC=12×80∘[∴∠BOC=80∘]=40∘

Topper's Solved these Questions
- THEOREMS RELATED TO ANGLES IN A CIRCLEBOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (Long Answer Type Questions)
31 Videos
- THEOREMS RELATED TO ANGLES IN A CIRCLEBOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (True or False)
4 Videos
- THEOREMS RELATED TO ANGLES IN A CIRCLEBOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (Fill in the blanks)
9 Videos
- THEOREMS RELATED TO CYCLIC QUADRILATERAL BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CYCLIC QUADRILATERAL EXERCISE - Long -answer type questions (L.A.)
12 Videos
- THEOREMS RELATED TO TANGENT IN A CIRCLE BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO TANGENT IN A CIRCLE EXERCISE - EXERCISE 4.2
23 Videos
Similar Questions
In the isosceles ΔABC,AB=AC. The circumcentre of ΔABC is the centre O lies on the opposite side of BC in which a lies. If ΔBOC=100∘, then find the value of ∠ABC∠ABO.
View SolutionIn the adjoining figure AD and BE are perpendicular ot BC and AC respectively of the ΔABC. The four points A,B,D,E are concylic.
View SolutionOn the same circular arc, the angle in circle is __________ of its central angle.
View SolutionIn a cyclic quadrilateral, the ratio of three consecutive angle is 1 : 2 : 3 Find the value of first and third angles.
View SolutionThe distance of the point from the centre of a circle with diameter of 16 cm is 17 cm. Then the length of the tangent from the point to the circle is
View SolutionIn the given figure , D is a point on the side BC of ΔABC such that ∠ADC=∠BAC. Prove that CA2=CB×CD.
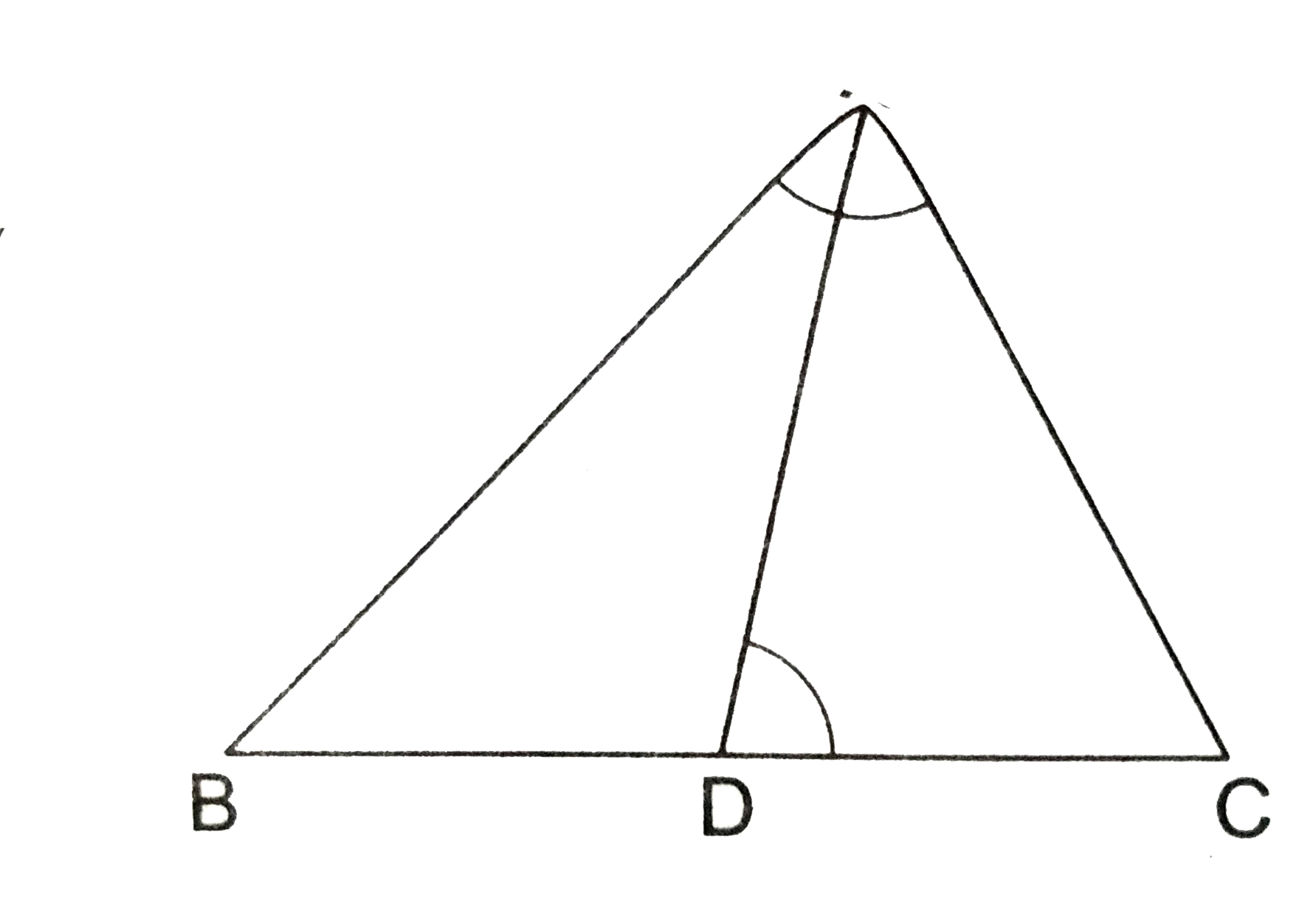
View SolutionO is the circumcentre of ΔABC and OD is perpendicular on the side BC, prove that ∠BOD=∠BAC
View SolutionO is the circumcentre of ΔABCandOD⊥BC. Prove that ∠BOD=∠BAC
View Solution(ii) Orthocentre of the triangle ABC is O, If ∠BAC=40∘, then ∠BOC=
View Solution(iii) Incentre of the triangle ABC is O, If ∠BAC=40∘, then ∠BOC=
View Solution(viii) O is the orthocentre of ΔABC. If ∠BOC=4∠BAC, then find ∠BOCand∠BAC.
View SolutionFrom the circumcentre O of traingle ABC , perpendicular OD is drawn on BC.If angle BAC =60 , Then the value of angle BOD
View SolutionIn a triangle ABC with side AB = AC and ∠BAC=20∘, D is a point on side AC and BC = AD. Find ∠DBC
View Solutionयदि ΔABC का परिकेन्द्र बिन्दु O तथा OD⊥BC हो, तो सिद्ध करो कि ∠BOD=∠A होगा।
View SolutionIn a triangle ABC with side AB = AC and ∠BAC=20∘, D is a point on side AC and BC = AD. Find ∠DBC
View Solution
