In the following figure AP⊥BC,AD∣∣BC, then find A(ΔABC):A(ΔBCD).
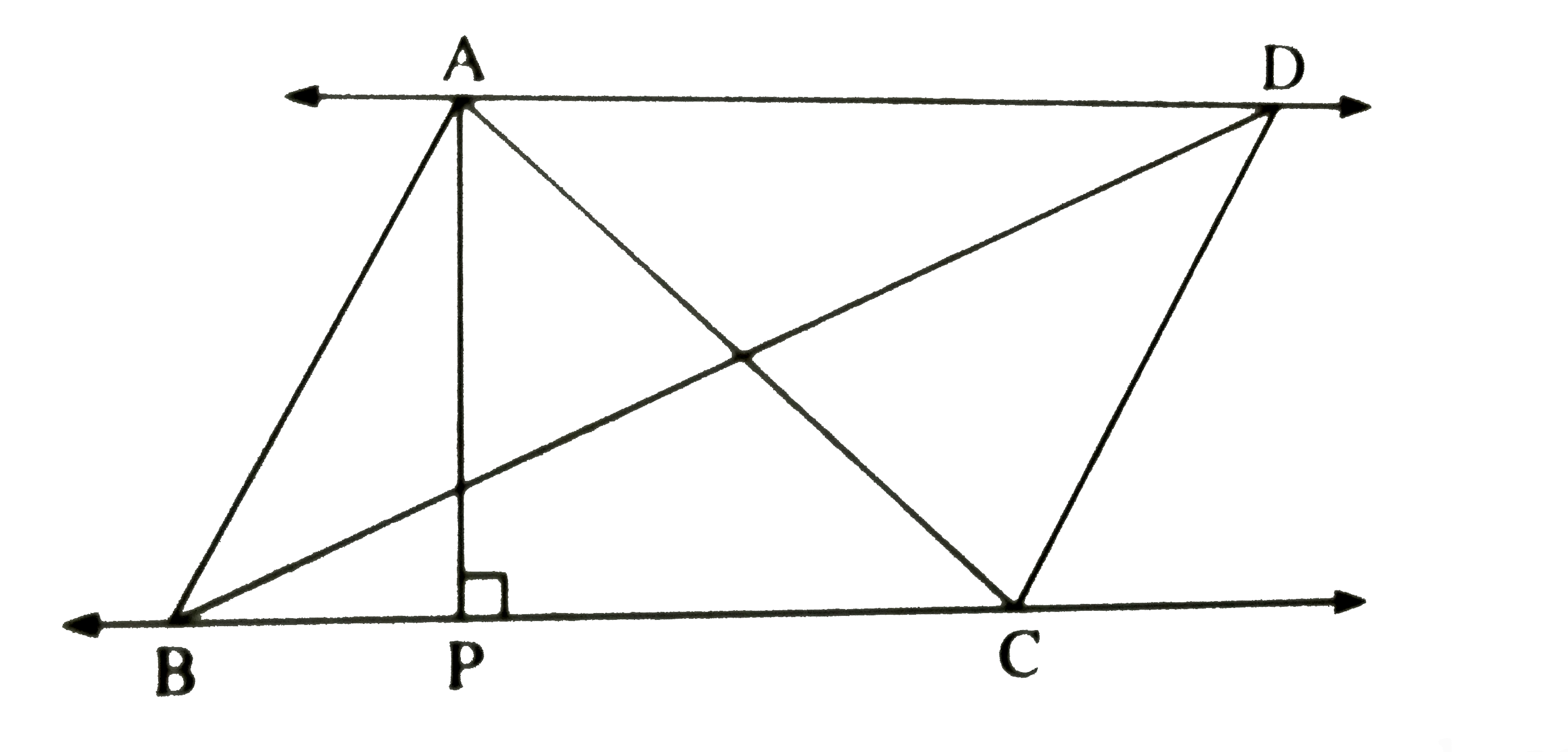
ΔABC and ΔBCD have same base BC. The vertices of ΔABC and ΔBCD lie on the parallel lines AD and BC. The distance between the two parallel lines is the height of the triangles.
Distance between parallel lines is constant.
∴ΔABC and ΔBCD have equal heights.
Areas of two traingles of equal bases and equal heights are equal.
∴A(ΔABC)=A(ΔBCD)
∴A(ΔABC)A(ΔBCD)=11
∴A(ΔABC):A(ΔBCD)=1:1
A(D≤taABC):A(ΔBCD)=1:1
Topper's Solved these Questions
- SIMILARITYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - CHALLENGING QUESTIONS
8 Videos
- SIMILARITYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - MCQ TYPE
15 Videos
- SIMILARITYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - 2.5 (4 mark each)
3 Videos
- QUADRATIC EQUATIONS BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUADRATIC EQUATIONS EXERCISE - ASSIGNEMENT 2.4
8 Videos
- STATISTICSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - STATISTICSEXERCISE - EXAMPLES FOR PRACTICE (MCQs)
35 Videos
Similar Questions
In the adjoining figure, AP⊥BC ,AD||BC ,then find A(ΔABC):A(ΔBCD) .
View SolutionIn the adjoining figure, AP⊥BC,AD||BC, then find A(ΔABC):A(ΔBCD).
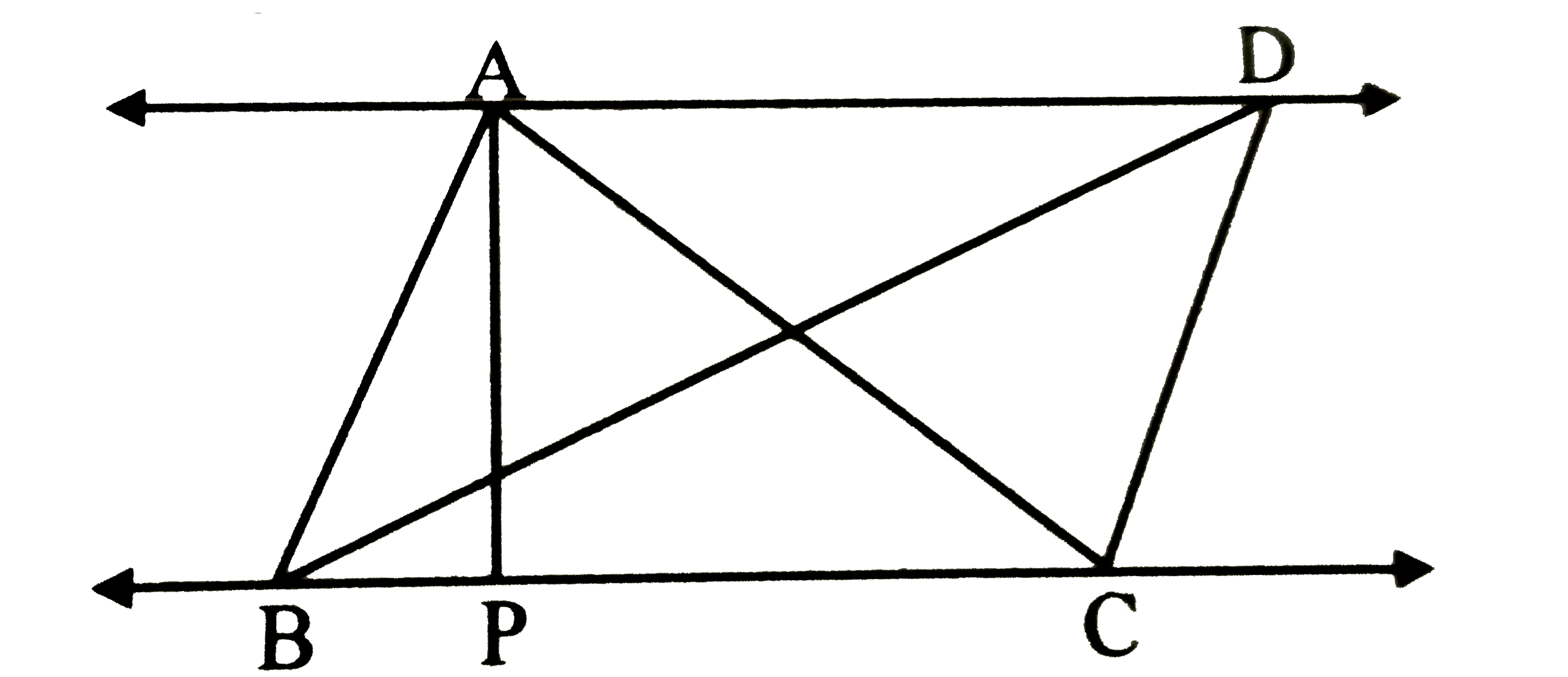
View SolutionIn adjoining figure,AP⊥BC , AD∣∣BC ,then find Ar.(ΔABC):Ar.(ΔBCD)Fig.
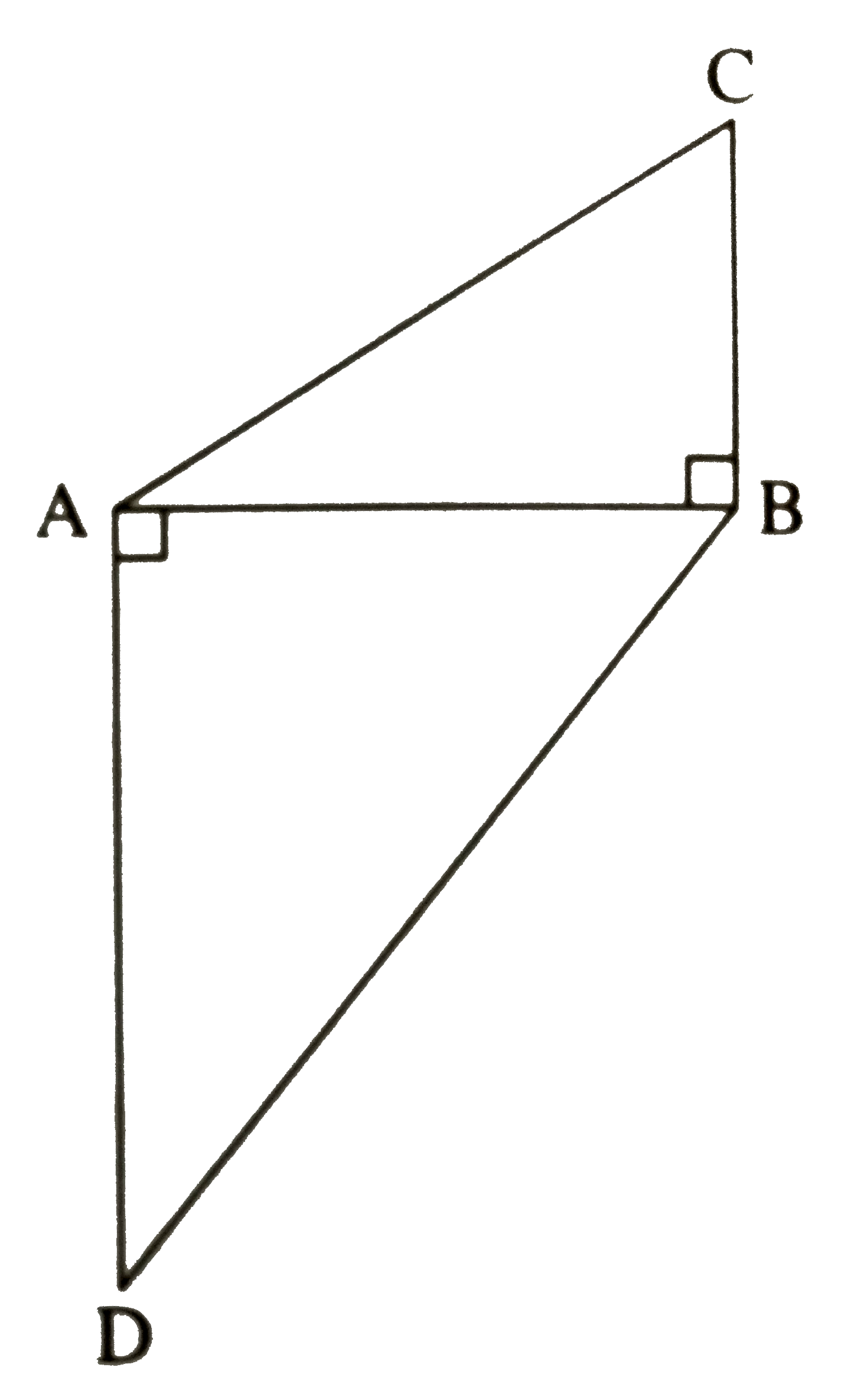
View SolutionIn the figure BC⊥AB,AD⊥AB,BC=4,AD=8, then find A(ΔABC)A(ΔADB).
View SolutionIn the adjoining figure, PQ⊥BC,AD⊥BC, then find ratio
A(ΔPBC)A(ΔABC).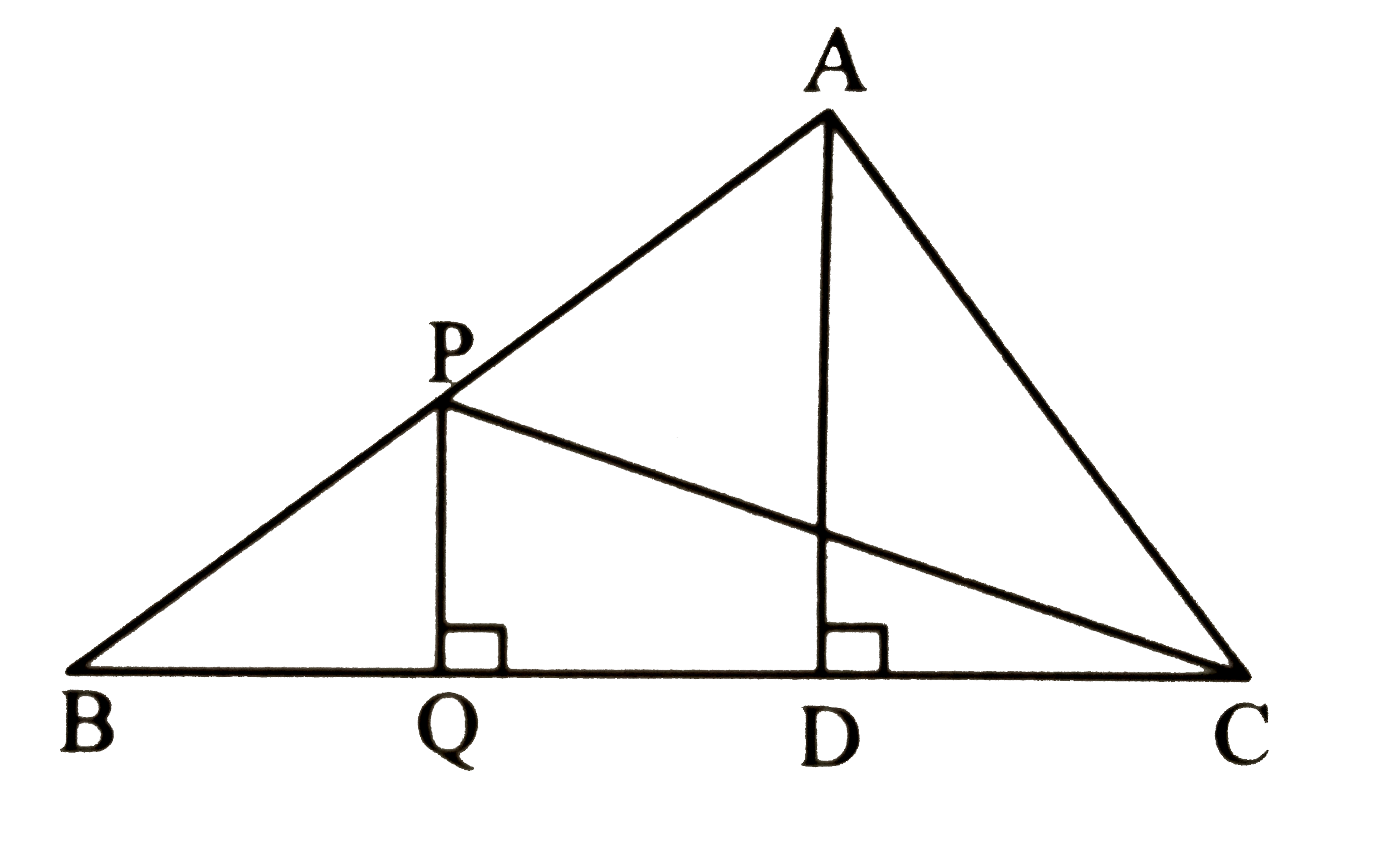
View SolutionIn the adjoining figure, PQ⊥BC,AD⊥BC, then find ratio
A(ΔABC)A(ΔADC).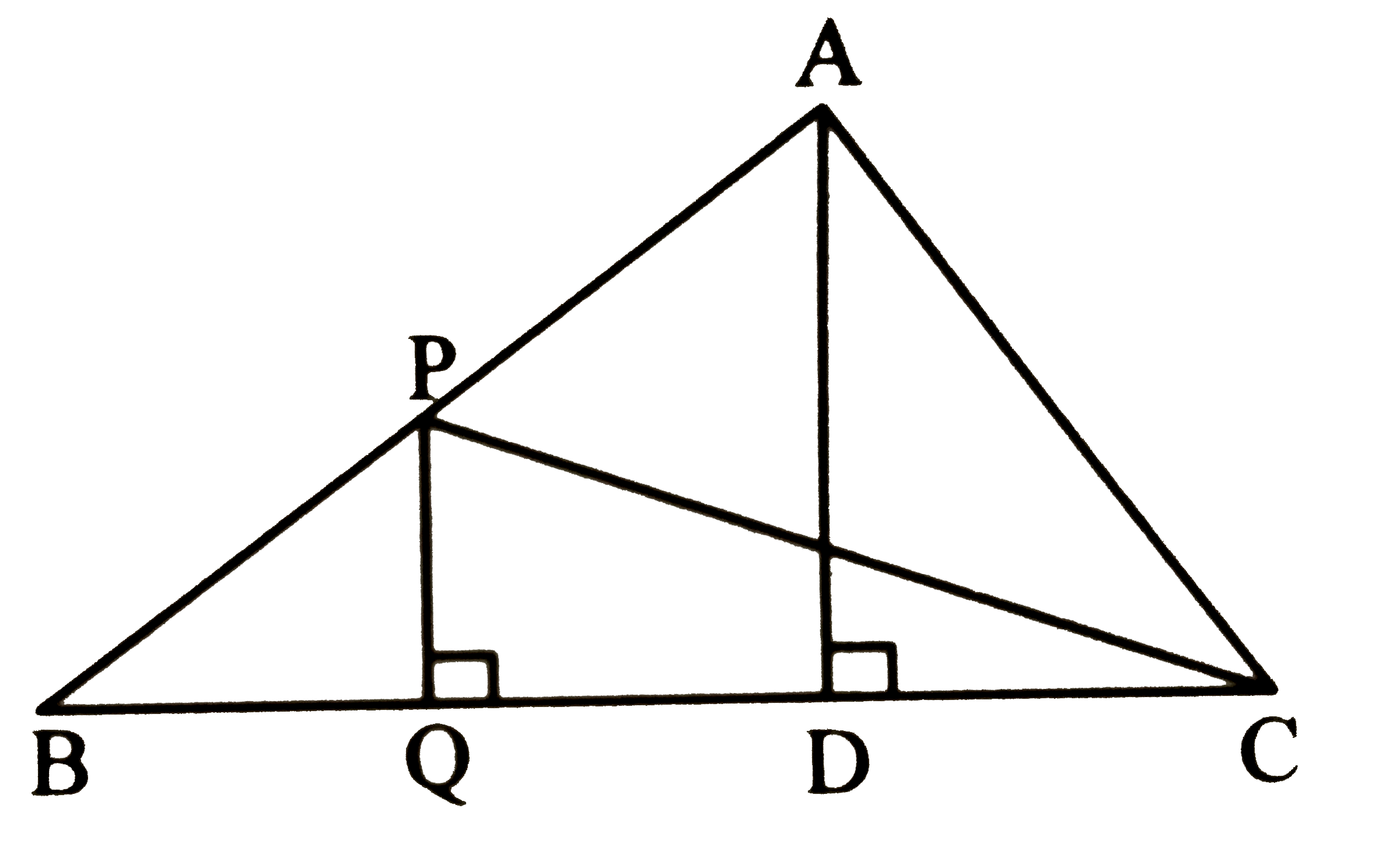
View SolutionIn the adjoining figure, PQ⊥BC,AD⊥BC, then find ratio
A(ΔPQB)A(ΔPBC).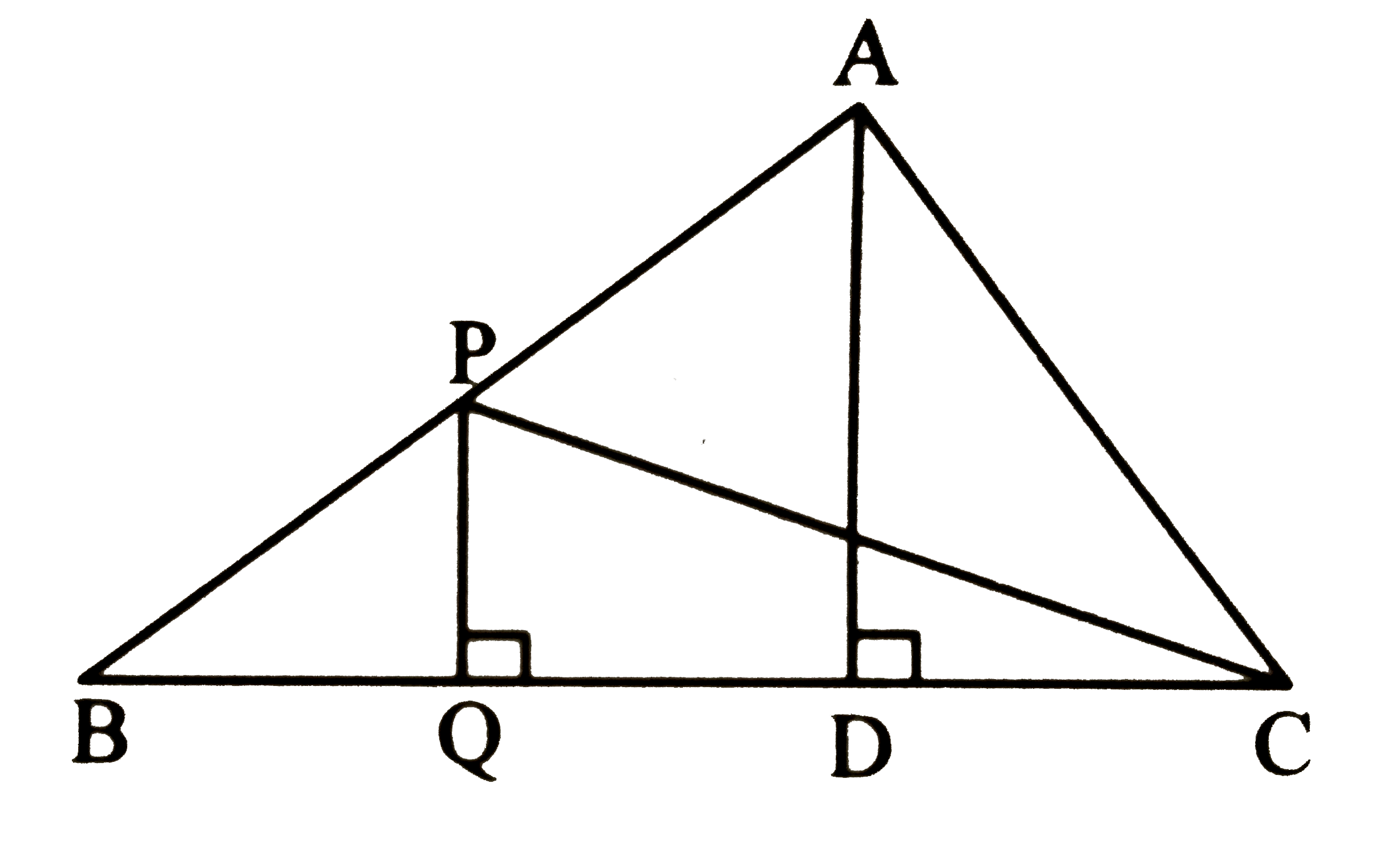
View SolutionIn the adjoining figure, PQ⊥BC,AD⊥BC, then find ratio
A(ΔADC)A(ΔPQC)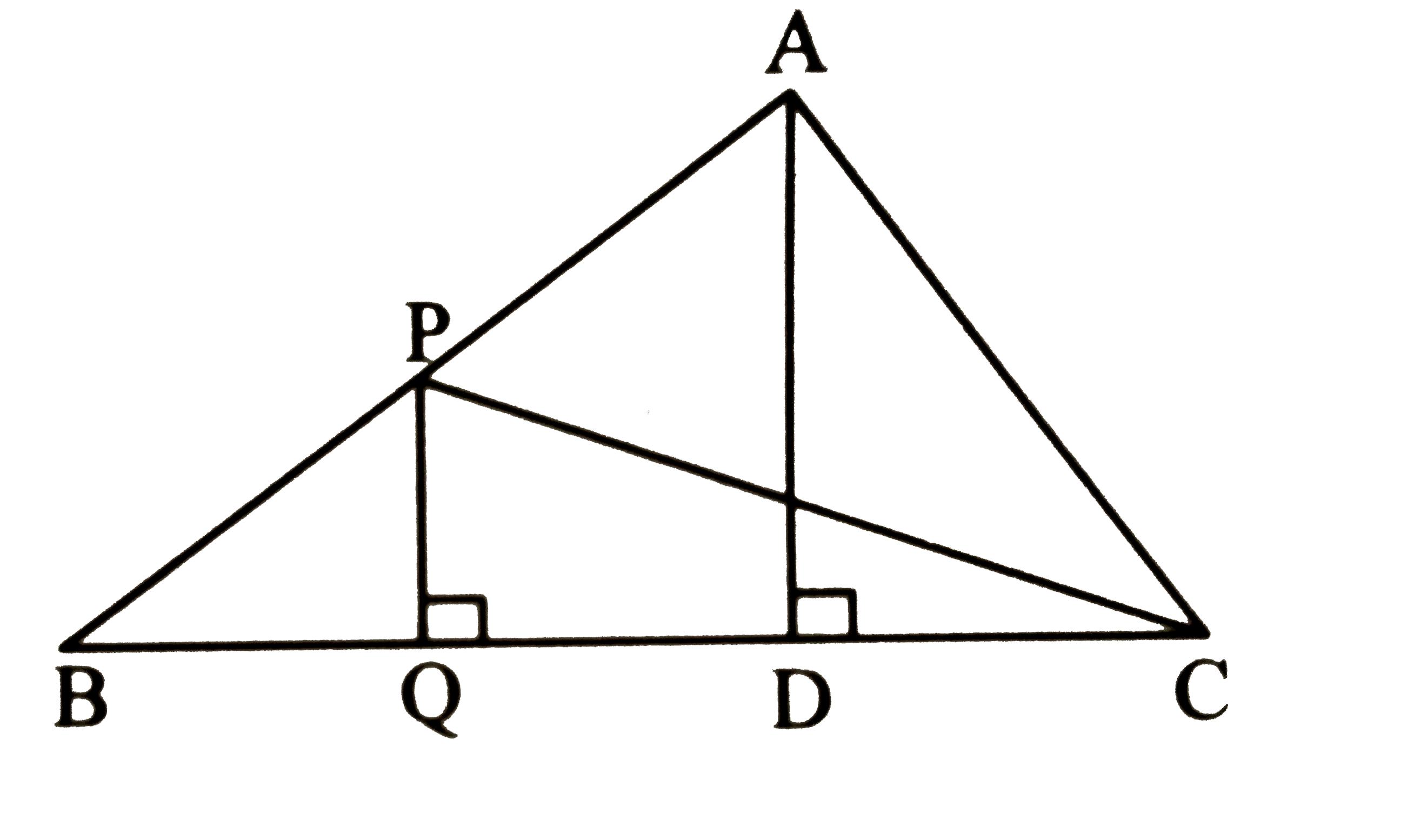
View SolutionIn the adjoining figure PQ⊥BC,AD⊥BC, then find following ratios:
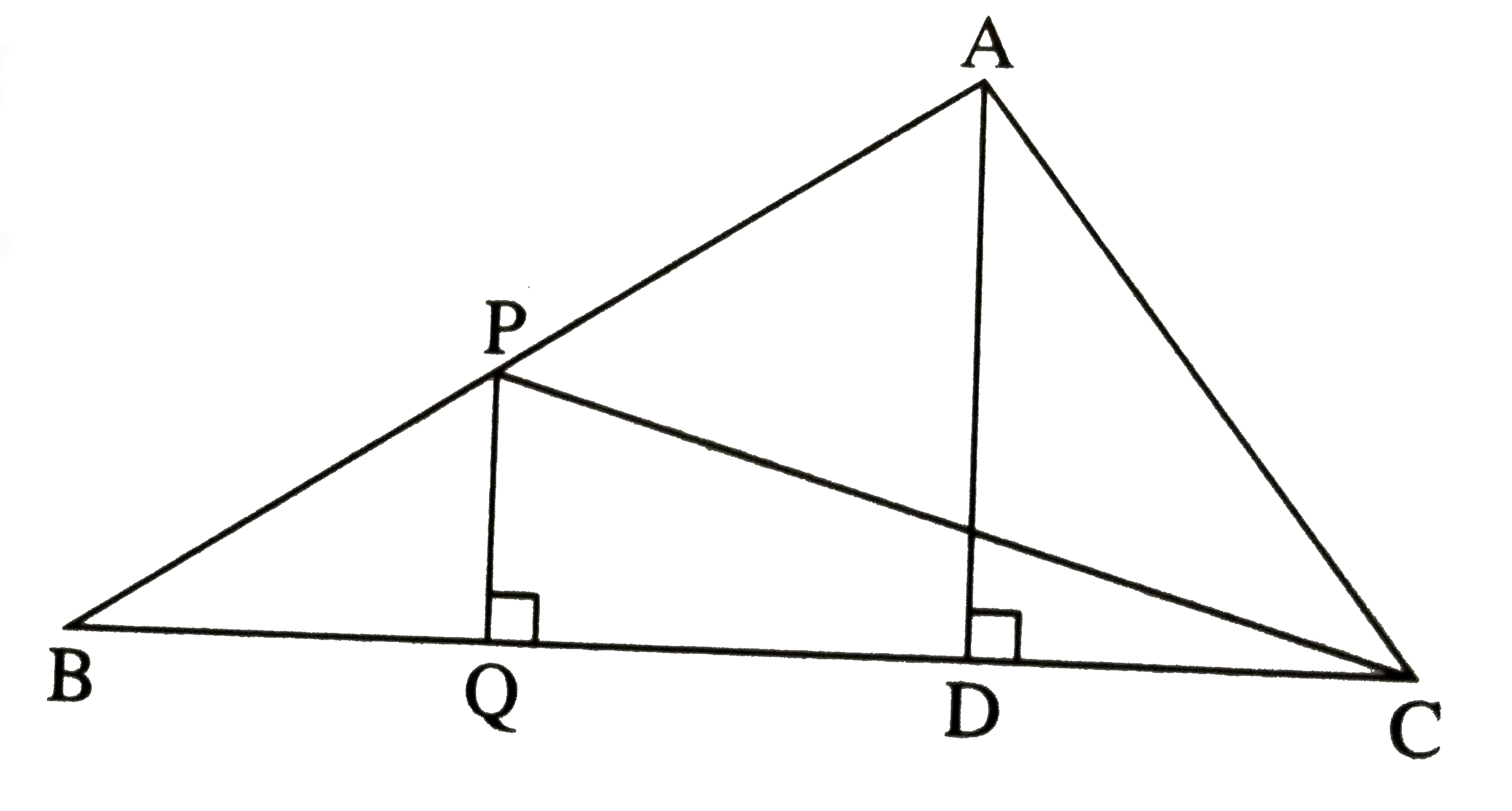
(i( A(ΔPQB)A(ΔPBC) (ii) A(ΔPBC)A(ΔABC)
(iii) A(ΔABC)A(ΔADC)(iv) A(ΔADC)A(ΔPQC)
View SolutionIn the given figure if AD||BC, find the value of x.
View Solution
