An element A decays into element C by a two-step process :
A→B+.2He4
B→C+2e¯
Then.
A and C are isotopes
A and C are isobars
B and C are isotopes
A and B are isobars
The correct Answer is:A
.AZA→.A−4Z−2B+.42He
.A−4Z−2B→.A−4ZC+20−1e
Hence, A and C are isotopes
Topper's Solved these Questions
- NUCLEIBOOK - NCERT FINGERTIPSCHAPTER - NUCLEIEXERCISE - Nuclear Energy
10 Videos
- NUCLEIBOOK - NCERT FINGERTIPSCHAPTER - NUCLEIEXERCISE - Higher Order Thinking Skills
8 Videos
- NUCLEIBOOK - NCERT FINGERTIPSCHAPTER - NUCLEIEXERCISE - Mass Energy And Nuclear Binding Energy
9 Videos
- MOVING CHARGES AND MAGNETISM BOOK - NCERT FINGERTIPSCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - Assertion And Reason
15 Videos
- PRACTICE PAPPERBOOK - NCERT FINGERTIPSCHAPTER - PRACTICE PAPPEREXERCISE - Practice Paper 3
50 Videos
Similar Questions
Knowledge Check
An element A decays into an element C by a two
AA and C are isotopesBA and C are isobarsCB and C are isotopesDA and B are isobarsAn element X decays into element Z by two -steps process
X→Y+He42
Y→Z+2e thenAX and Z are isobars.BX and Y are isotopesCX and Z are isotopesDX and Z are isotopes.A fixed mass of gas is taken through a process A→B→C→A. Here A→B is isobaric, B→C is adiabatic and C→Ais isothermal.
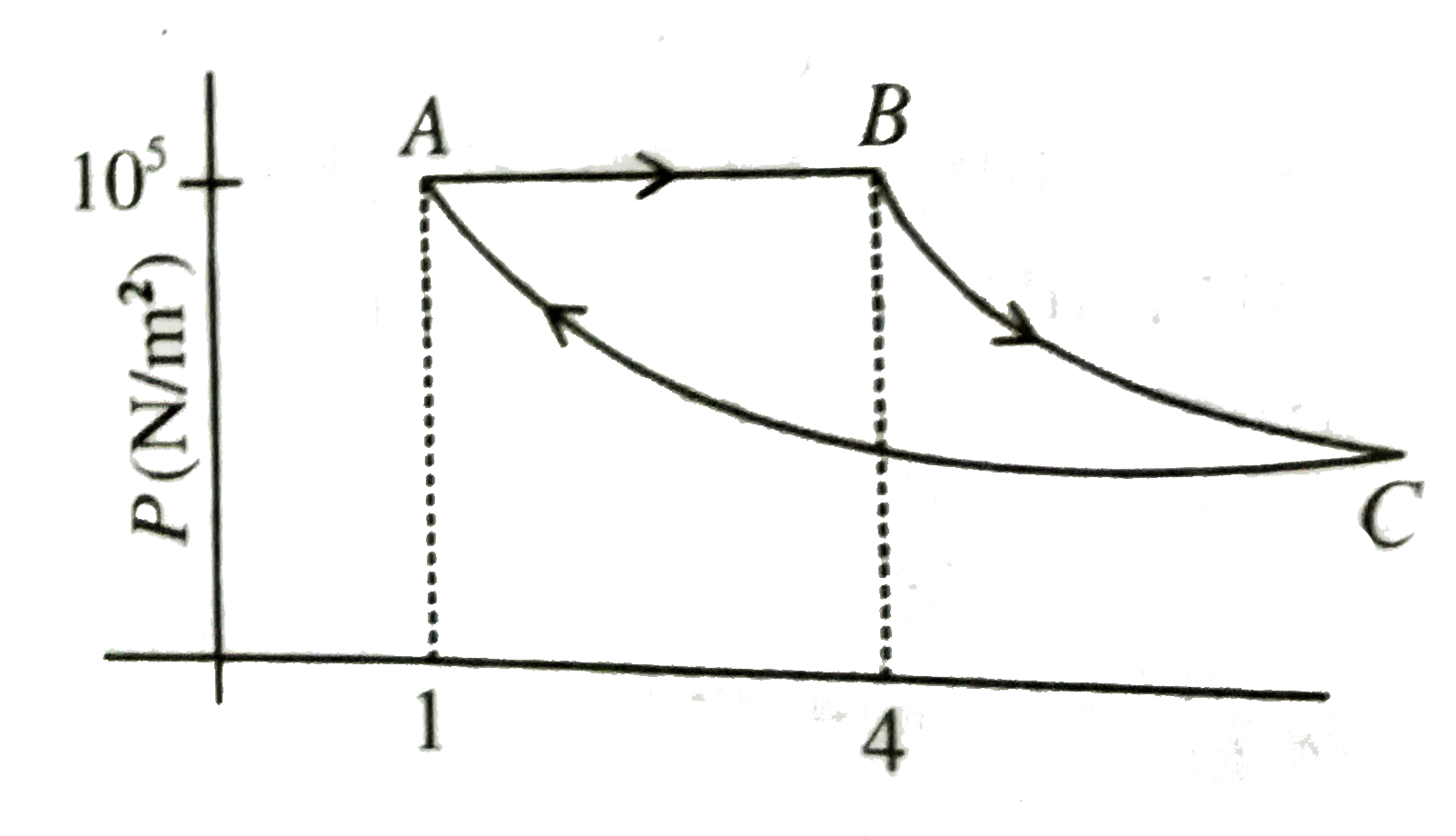
Find pressure at C.A10564N/m2B10532N/m2C10512N/m2D1056N/m2
An ideal gas (2.0 moles) is carried round a cycle as shown. If the process b→c is isothermal and CV=3cal/mol/K. Determine
a. work done,
b. change in internal energy,
c. heat supplied to the system during processes a→b, b→c and c→a.
View SolutionA fixed mass of gas is taken through a process A→B→C→A. Here A→B is isobaric, B→C is adiabatic and C→Ais isothermal.
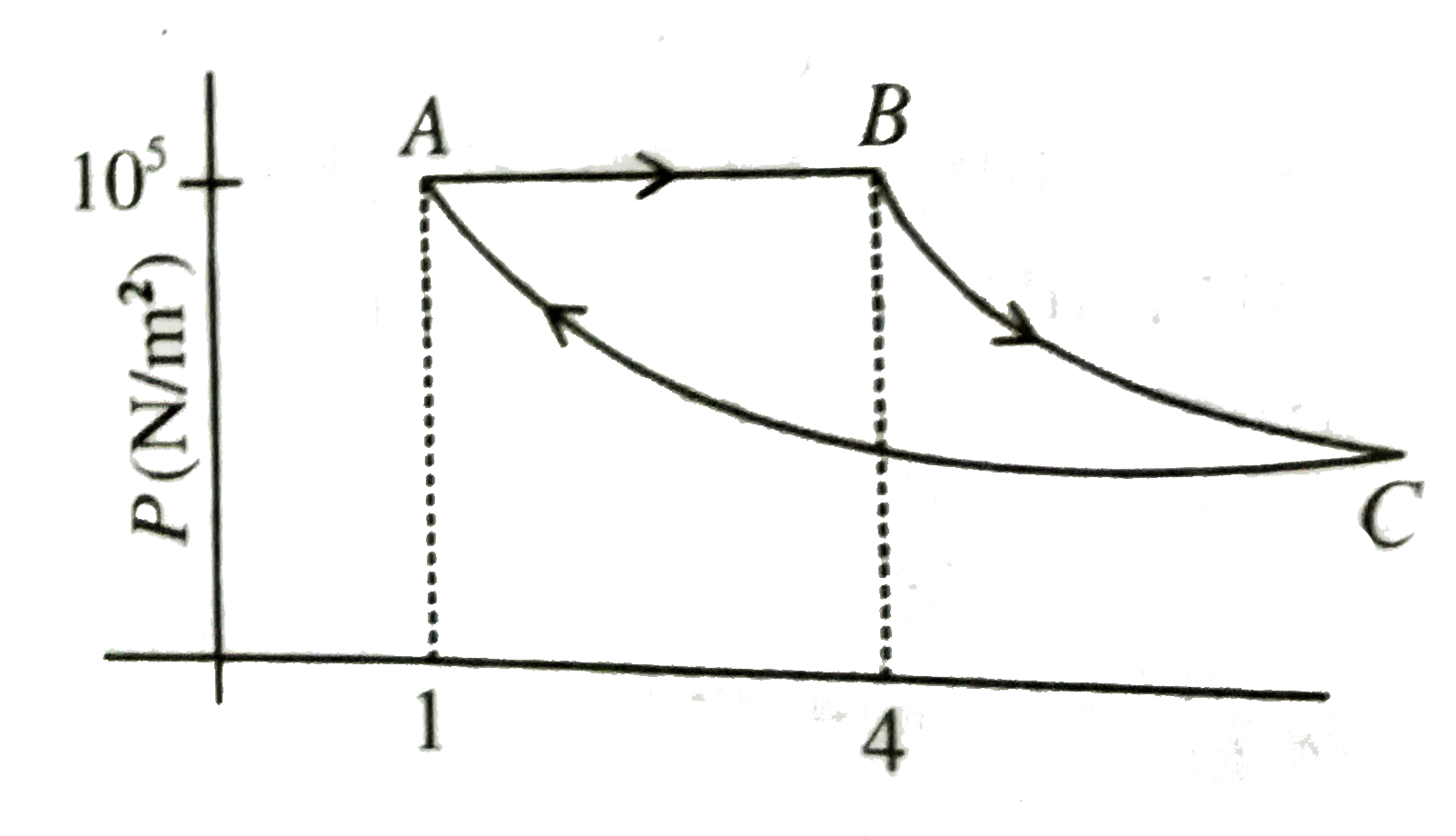 Find volume at C.
Find volume at C.
View SolutionA fixed mass of gas is taken through a process A→B→C→A. Here A→B is isobaric, B→C is adiabatic and C→Ais isothermal.
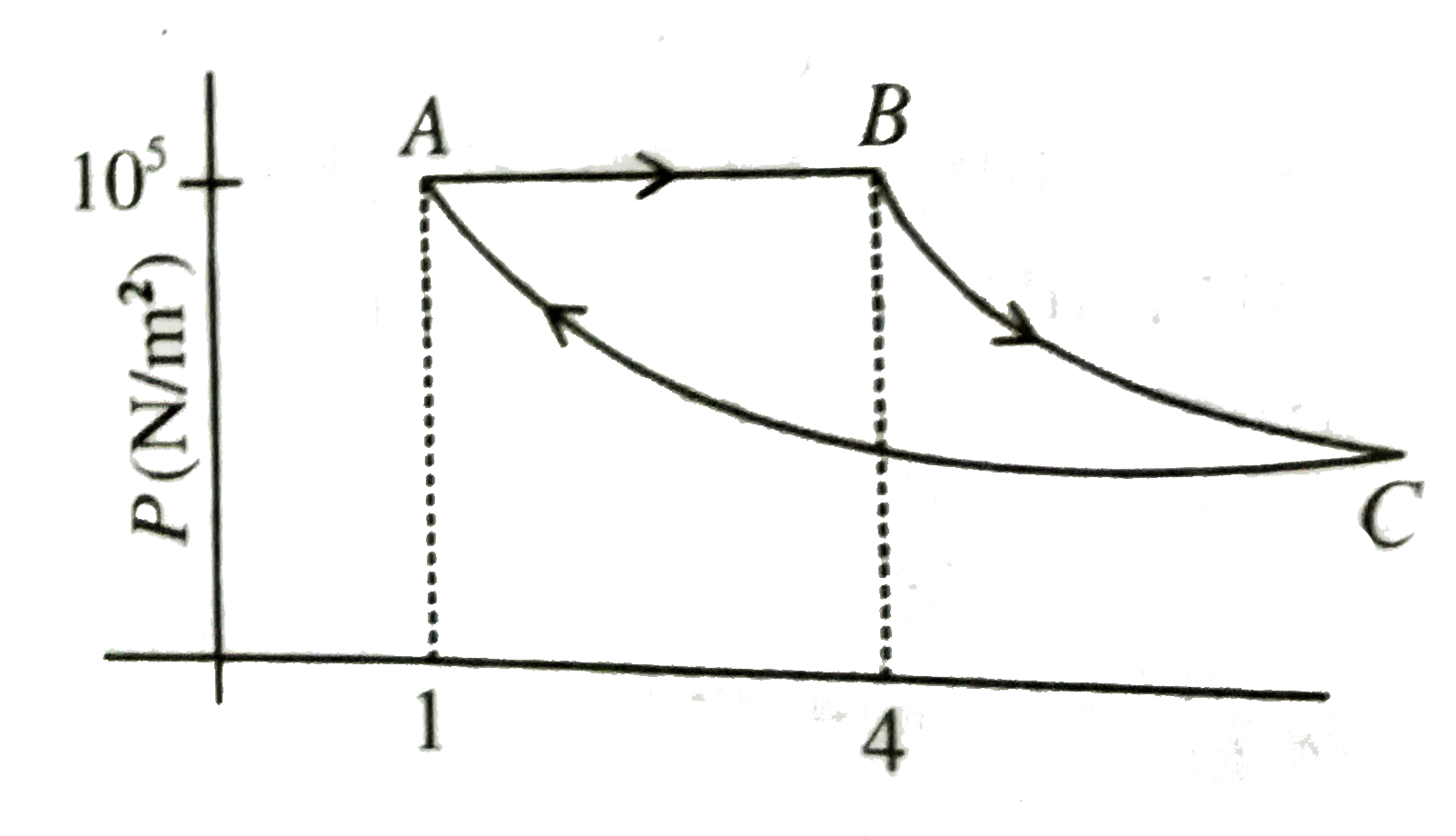
Find work done is the process ( take γ=1.5).
View SolutionA hypothetical reaction, A→2B proceeds through following sequence of steps
A→C,ΔH=a
C→D,ΔH=b
12D→B:ΔH=c
The heat of reaction is :
View SolutionIn the decay process:
A−α−−→B−β−−→C−β−−→D
View Solution
