In ΔABC, ∠C is an acute angle, seg AD⊥ seg BC. Prove AB2=BC2+AC2−2BC×DC by completing the following activity.
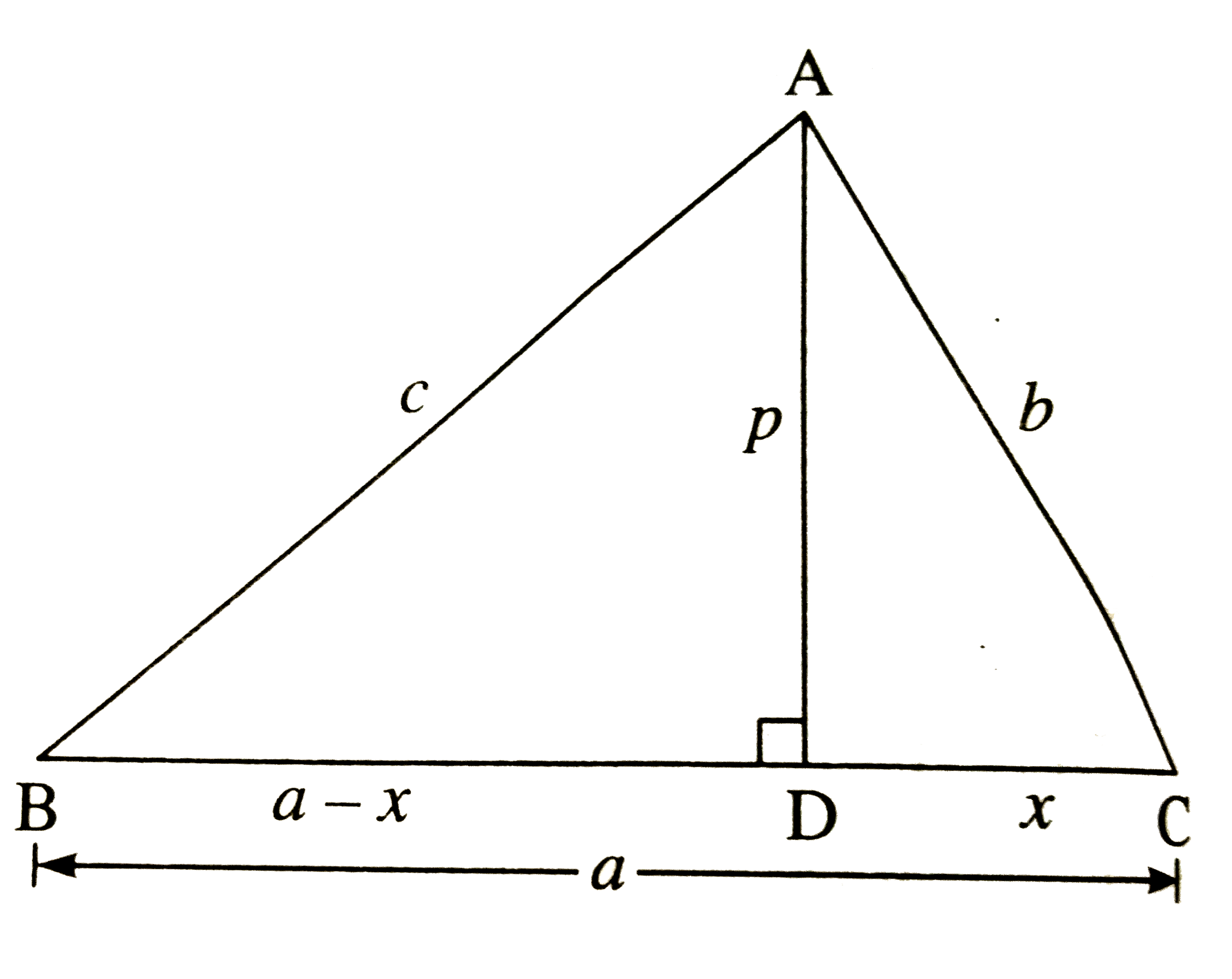
Let AB=c, AC=b, AD=p, BC=a, DC=x
∴BD=a−x
In ΔADB, by Pythagoras theorem,
c2=(a−x)2+□
c2=a2−2ax+x2+□...........(1)
In ΔADC, by Pythagoras theorem,
b2=p2+□
p2=b2−□ ..........(2)
Substituting value of p2 from (2) in (1)
c2=a2−2ax+x2+□
c2=a2+b2−□
∴AB2=BC2+AC2−2BC×DC.
Video Solution
Text Solution
In ΔADB, by Pythagoras theorem,
c2=(a−x)2+p2
c2=a2−2ax+x2+p2.......(1)
In ΔADC, by Pythagoras theorem,
b2=p2+x2
p2=b2−x2..........(2)
Substituting value of p2 from (2) in (1)
c2=a2−2ax+x2+b2−x2
c2=a2+b2−2ax
∴AB2=BC2+AC2−2BC×DC.
|
Topper's Solved these Questions
- PYTHAGORAS THEOREMBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PYTHAGORAS THEOREMEXERCISE - 3.5 (4 mark each)
4 Videos
- PYTHAGORAS THEOREMBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PYTHAGORAS THEOREMEXERCISE - Assignment 3.1
8 Videos
- PYTHAGORAS THEOREMBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PYTHAGORAS THEOREMEXERCISE - 3.3 (2 mark each)
8 Videos
- PROBABILITY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY EXERCISE - ASSIGNEMENT 5.4
12 Videos
- QUADRATIC EQUATION BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUADRATIC EQUATION EXERCISE - CHALLENGIN QUESTIONS
2 Videos
Similar Questions
In a ΔABC, ∠B is an acute-angle and AD⊥BC. Prove that:
AB2+CD2=AC2+BD2
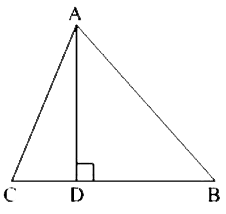
View SolutionIn ΔABC, seg AD⊥ seg BCDB=3CD.
Prove that : 2AB2=2AC2+BC2
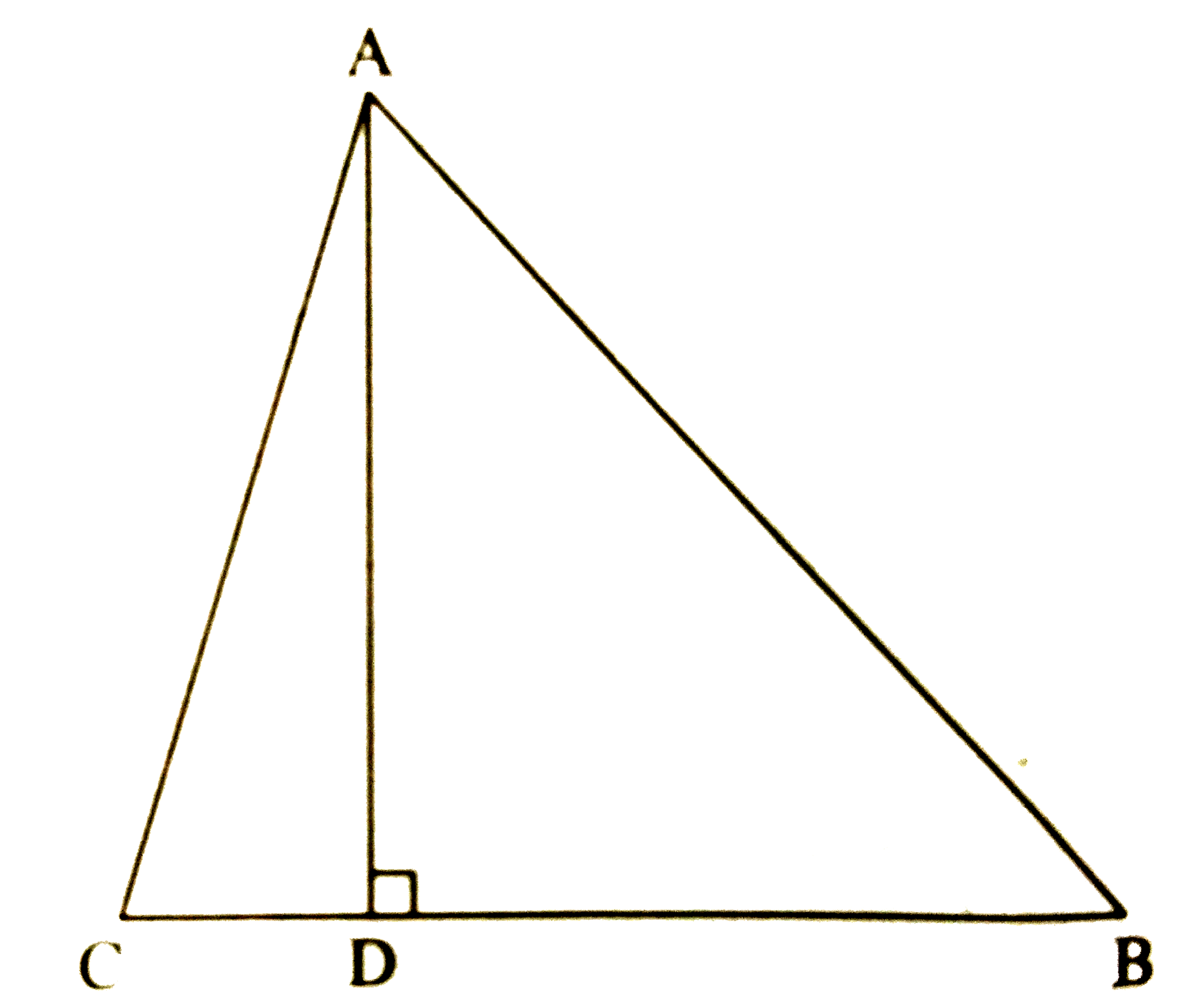
View SolutionIn ΔABC,
seg AD⊥ seg BC,
DB=3CD.
Prove that 2AB2=2AC2+BC2.
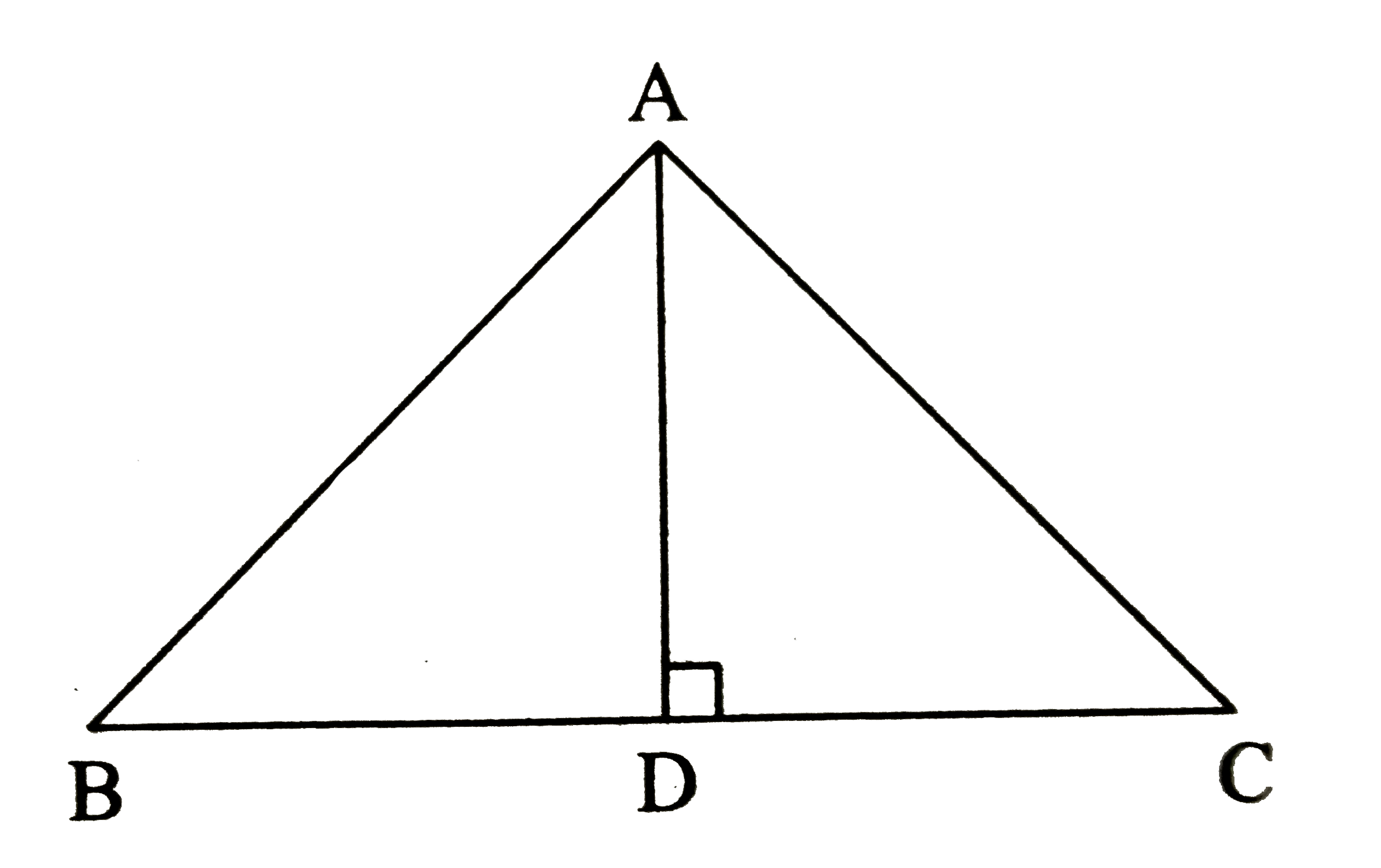
View SolutionIn ΔABC seg AD⊥segBC,DB=3CD Prove that 2AB2=2AC2+BC2
View SolutionIn the figure, seg AD⊥ side BC and B−D−C, then prove AB2−BD2=AC2−CD2.
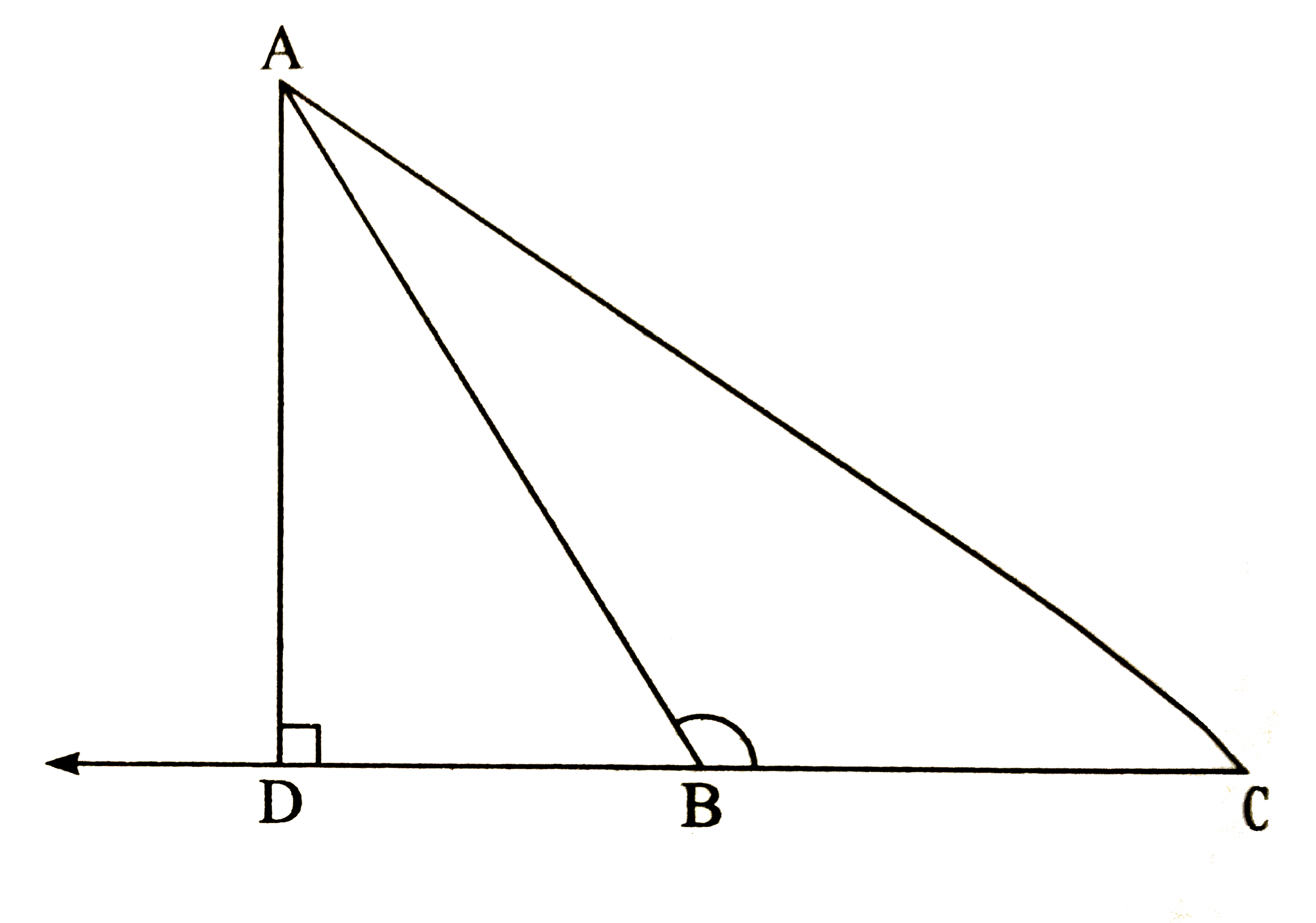
View SolutionIn figure, ∠B<90∘andAD⊥BC. Prove that AC2=AB2+BC2−2BC.BD
View SolutionIn fig. ∠B of △ABC is an acute angle and AD⊥BC ; prove that AC2=AB2+BC2−2BC×BD
View SolutionIn ΔABC, if M is the midpiont of BC and seg AM ⊥ seg BC, then prove that prove that AB2+AC2=2AM2+2BM2.
View Solution(a+b+c)(a2+b2+c2−ab−bc−ac)
View SolutionIn obtuse angled ΔABC, ∠B>90∘. If seg Ad⊥ ray CB and D−B−C, then prove that AC2=AB2+BC2+2BC⋅DB.
View Solution
