Entries of column I are to be matched with one or more entries of column II.

Video Solution

Text Solution

Verified by Experts

(A) $r \to (r, s, t), (B) \to (p, q, r), (C) \to (r, s, t)$
(A)Let $f (x) = a x^{2} + b x + c$
Then $f (1) = a + b + c = - c [∵ a + b + 2 c = 0]$
and $f (0) = c$
$∴ f (0) f (1) = - c^{2} < 0 [∵ c \neq 0]$
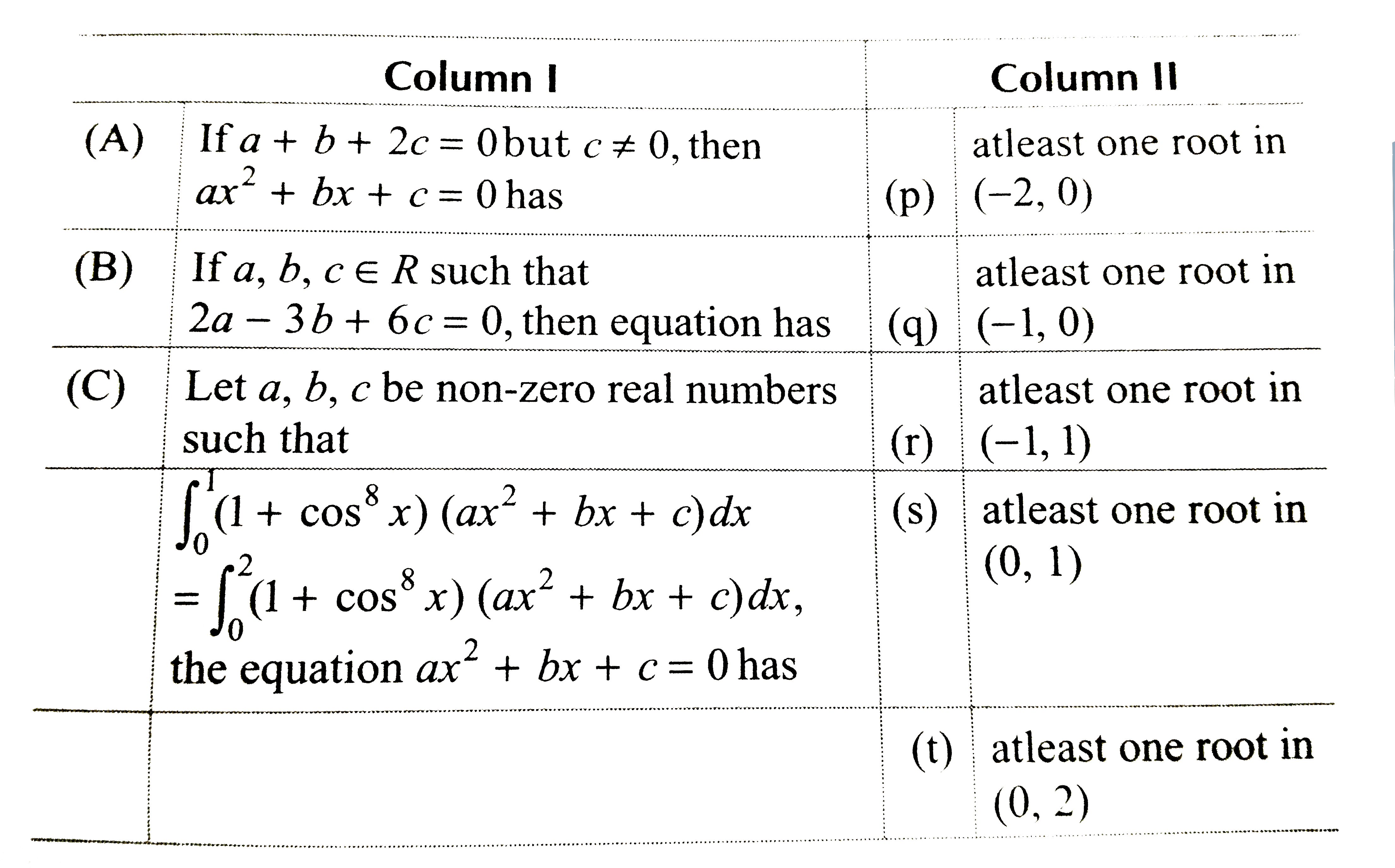
$∴$ Equation $f (x) = 0$ has a root in (0,1).
$∴ f (x)$ has a root in (0,2) as well as in $(- 1, 1) (r)$
(B)Let $f' (x) = a x^{2} + b x + c$
$∴ f (x) = \frac{a x^{3}}{3} + \frac{b x^{2}}{2} + c x + d$
$∴ f (0) = d$
and $f (- 1) = - \frac{a}{3} + \frac{b}{2} + c = d = - (\frac{2 a - 3 b + 6 c}{6}) + d$
$= 0 + d = d [∵ 2 a - 3 b + 6 c = 0]$
Hence $f (0) = (f - 1)$
Hence $f' (x) = 0$ has atleast one root in $(- 1, 0) (q)$
$∴ f (x) = 0$ has a root in $(- 2, 0) (p)$ as well as $(- 1, 1) (r)$
(C)Let $f (x) = \int (1 + \cos^{8} x) (x^{2} + b x + c) d x$
Given $f (1) - f (0) = f (2) - f (0)$
$\Rightarrow f (1) = f (2)$
$\Rightarrow f' (x) = 0$ has atleast one root in (1,2).
$\Rightarrow (1 + \cos^{8} x) (a x^{2} + b x + c) = 0$ has atleast one root in (1,2).
$\Rightarrow a x^{2} + b x + c = 0$ has atleast one root in (0,2) (t)

Updated on:21/07/2023

|

Class 12 MATHS QUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions

ARIHANT MATHS-THEORY OF EQUATIONS-Exercise (Questions Asked In Previous 13 Years Exam)

Home
Ask Doubt
Profile