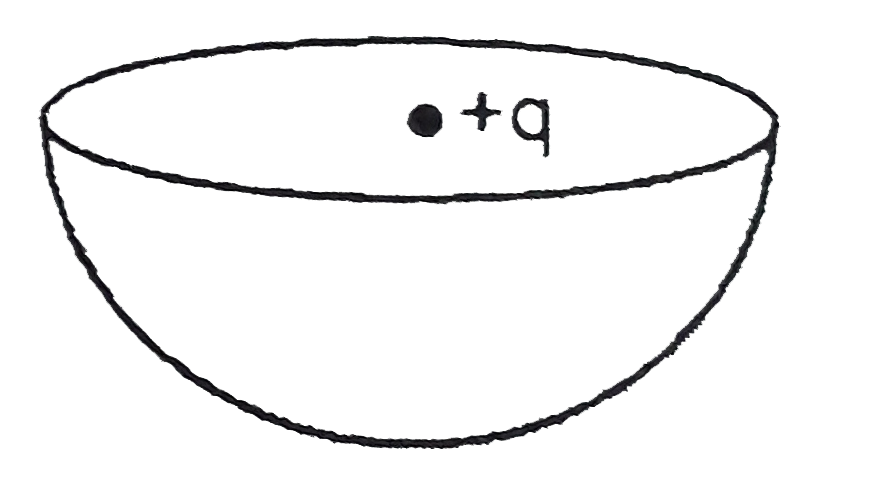
A point charge q is placed at the origin. Calculate the electric flux through the open hemispherical surface (x−a)2+y2+z2=a2,x≥a
Video Solution
Text Solution
The correct Answer is:A, B
a. 
Flux passing through hemisphere = flux passing through circular surface of the hemisphere. For finding flux through circular surface of hemisphere we can again use the concept used i above problem.

ϕ=q(1−cosθ)2ε0
=12ε0(1−cos45∘)
=q2ε0(1−1√2)
|
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - SUBJECTIVE_TYPE
6 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Assertion And Reason
19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
Similar Questions
Knowledge Check
A charge 2q is placed at the mouth of a conical flask. The electric flux through the flask will be
AzeroBQε0CQ2ε0D<Q2ε0Charge of 2 C is placed at the centre of a cube. What is the electric flux passing through one face ?
A1(3ε0)B(14)ε0C2ε0D3ε0A point charge Q is placed at one of the vertices of a cubical block. The electric flux flowing through this cube is
AQ6ε0BQ4ε0CQ8ε0DQε0
A charge Q is placed at the centre of a cub. Find the flux of the electric field through the six surfaced of the cube.
View SolutionA point charge +Q is placed at a point. Calculate the electric flux associated with one sphere of radius r , which the given point charge at its centre.
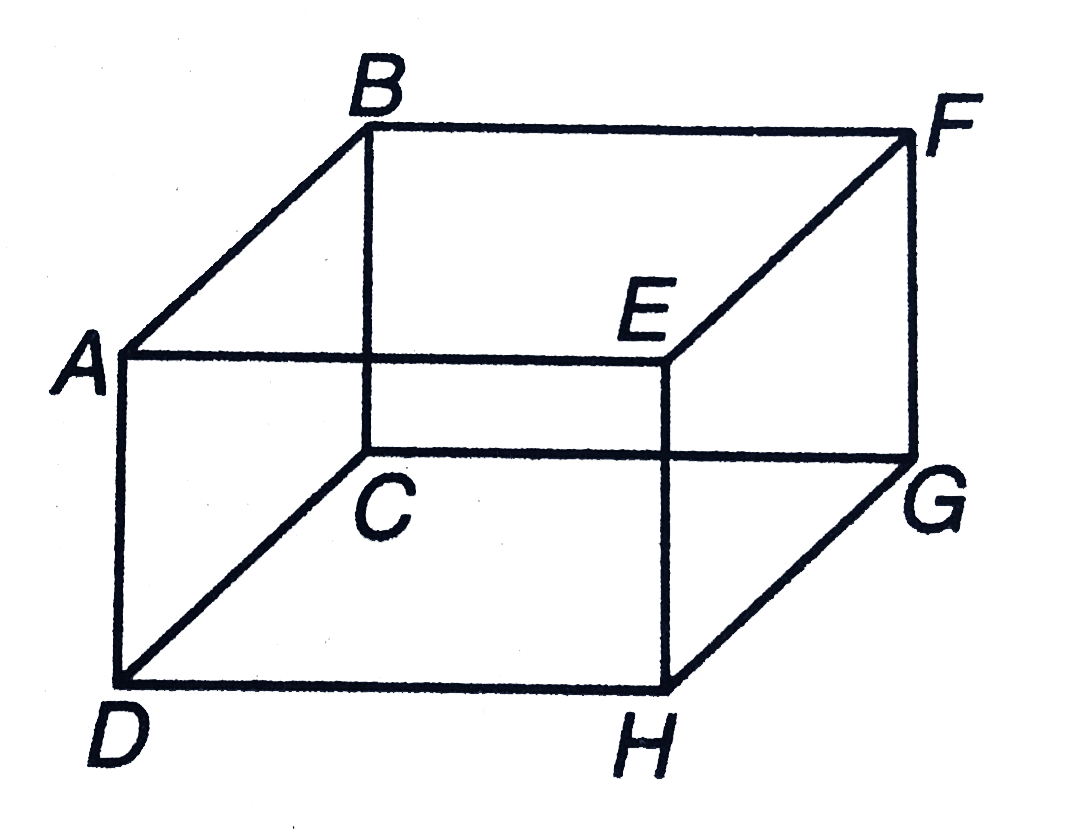
View SolutionA charge q is placed at point D of the cube. Find the electric flux passing through the EFGH and face AEHD.
View SolutionAn electric charge q is placed at the centre of a cube of side l. The electric flux through one of its faces will be
View Solutiona point charge q is placed at the centre of a cylinder of length L and radius R the electric flux through the curved surface of the cylinder is
View Solution