Charge 2qand−q are placed at (a,0)and(−a,0) as shown in the figure. The coordinates of the point at which electric field intensity is zero will be (x,0) where
Charge 2q and -q are placed at (a,0) and (-a, 0) as shown in the figure. The coordinates of the point at which electric field intensity is zero will be (x,0) where

A

−a<x<a

B

x<−a

C

x>−a

D

0<x<a

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B

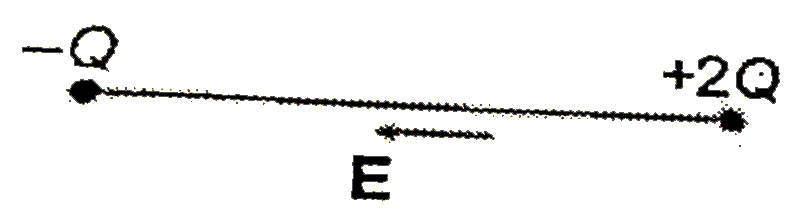
Between 2q and −q, two electric fields are in same direction. So their resultant can't be zero. To the right of 2q left of -q they are in opposite directions. So net field will be zero nearer to charge having small magnitude.

Updated on:21/07/2023
|
Class 12PHYSICSELECTRIC CHARGES AND FIELDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Charges +2Q and −Q are placed as shown in figure. The point at which electric field intensity is zero will be
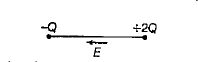    ocr_image

    ASomewhere between −Q and +20
    BSomewhere on the left of −Q
    CSomewhere on the right of +2Q
    DSomewhere on the right bisector of line Joining−Q and +2Q

  • Two charges e and 3e are placed at a distance r. The distance of the point where the electric field intensity will be zero is

    Ar(1+√3) from 3e charge
    Br(1+√3) from e charge
    Cr(1−√3) from 3e charge
    Dr1+√13 from e charge

  • Two charge 9e and 3e are placed at a distance r. The distance of the point where the electric field intensity will be zero is :

    A(r1+√3) from 9e charge
    B(r1+√1/3) from 9e charge
    C(r1−√3) from 3e charge
    D(r1+√1/3) from 3e charge