For a cell, the terminal potential difference is 2.2V, when circuit is open and reduces to 1.8V. When cell is connected to a resistance R=5Ω, the internal resistance of cell (R) is
109Ω
910Ω
119Ω
59Ω
The correct Answer is:A
To find the internal resistance of the cell, we can follow these steps:
Step 1: Understand the given values
- The terminal potential difference when the circuit is open (no load) is E=2.2V.
- The terminal potential difference when connected to a resistance R=5Ω is V=1.8V.
Step 2: Calculate the current flowing through the circuit
When the resistance R is connected, we can calculate the current I using Ohm's law:
I=VR=1.8V5Ω=0.36A
Step 3: Apply Kirchhoff's loop law
According to Kirchhoff's loop law, the sum of the potential differences in a closed loop is equal to zero. For our circuit, we can express this as:
E−I⋅R−I⋅r=0
Where:
- E is the EMF of the cell (2.2 V),
- I is the current (0.36 A),
- R is the external resistance (5 Ω),
- r is the internal resistance of the cell.
Rearranging the equation gives us:
E=I⋅R+I⋅r
Step 4: Substitute the known values
Substituting the known values into the equation:
2.2=(0.36⋅5)+(0.36⋅r)
Step 5: Simplify the equation
Calculating 0.36⋅5:
0.36⋅5=1.8
So the equation becomes:
2.2=1.8+0.36⋅r
Step 6: Solve for the internal resistance r
Subtract 1.8 from both sides:
2.2−1.8=0.36⋅r
0.4=0.36⋅r
Now, divide both sides by 0.36:
r=0.40.36≈1.11Ω
Step 7: Conclusion
The internal resistance of the cell is approximately 1.11Ω.
---
- The terminal potential difference when the circuit is open (no load) is E=2.2V.
- The terminal potential difference when connected to a resistance R=5Ω is V=1.8V.
When the resistance R is connected, we can calculate the current I using Ohm's law:
I=VR=1.8V5Ω=0.36A
According to Kirchhoff's loop law, the sum of the potential differences in a closed loop is equal to zero. For our circuit, we can express this as:
E−I⋅R−I⋅r=0
Where:
- E is the EMF of the cell (2.2 V),
- I is the current (0.36 A),
- R is the external resistance (5 Ω),
- r is the internal resistance of the cell.
E=I⋅R+I⋅r
Substituting the known values into the equation:
2.2=(0.36⋅5)+(0.36⋅r)
Calculating 0.36⋅5:
0.36⋅5=1.8
So the equation becomes:
2.2=1.8+0.36⋅r
Subtract 1.8 from both sides:
2.2−1.8=0.36⋅r
0.4=0.36⋅r
r=0.40.36≈1.11Ω
The internal resistance of the cell is approximately 1.11Ω.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - OBJECTIVE_TYPE
1 Video
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - Level 1 Subjective
53 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - Level 1 Assertion And Reason
11 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
When a resistor of 5Ω is connected across a cell, its terminal potential differnce is balanced by 140 cm of potentiometer wire and when a resistance of 8Ω is connected across the cell, the terminal potential difference is balanced by 160 cm of the potentiometer wire. Find the internal resistance of the cell.
View SolutionIn the given circuit, an ideal voltmeter connected across the 10Ω resistance reads 2V. The internal resistance r, of each cell is:
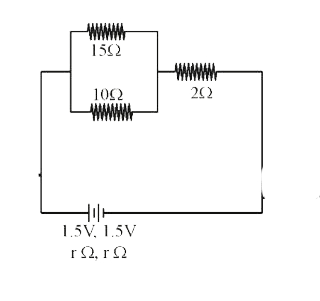
View Solution
Knowledge Check
For a cell terminal potential difference is 2.2 V when circuit is open and reduces to 1.8V when cell is connected to a resistance of R=5Ω then determine internal resistance of cell is:-
A109ΩB910ΩC119ΩD59ΩThe potential difference between the terminals of a cells is found to be 3 volts when it is connected to a resistance equal to its internal resistance. The e.m.f. of the cell is :–
A3VB6VC1.5VD4.5VThe terminal potential difference for a cell is 8.5 V when current is 2A and 9 V when current is 1.5A. What is the internal resistance of cell ?
A4ΩB2ΩC3ΩD1Ω
The reading of a voltmoter when a cell is connected to it is 2.2 V. When the terminals of the cell are connected to a resistance of 4Ω, the voltmeter reading drops to 2 V. Find the internal resistance of the cell.
View SolutionWhen a resistor of 5Ω is connected across a cell, its terminal potential differnce is balanced by 140 cm of potentiometer wire and when a resistance of 8Ω is connected across the cell, the terminal potential difference is balanced by 160 cm of the potentiometer wire. Find the internal resistance of the cell.
View SolutionWhen current supplied by a cell to a circuit is 0.3A, its terminal potential difference is 0.9 A. When the current supplied becomes 0.2 A, its terminal potential difference becomes 1.0 V . The internal resistance of the cell is
View SolutionWhen current supplied by a cell to a circuit is 0.3A, its terminal potential difference is 0.9 A. When the current supplied becomes 0.2 A, its terminal potential difference becomes 1.0 V . The internal resistance of the cell is
View SolutionThe emf of a cell is 2 V. When the terminals of the cell is connected to a resistance 4Ω. The potential difference across the terminals, if internal resistance of cell is 1Ω is
View Solution
