When a resistor of is connected across a cell, its terminal potential differnce is balanced by 140 cm of potentiometer wire and when a resistance of is connected across the cell, the terminal potential difference is balanced by 160 cm of the potentiometer wire. Find the internal resistance of the cell.
Here, ,
Let emf of the cell be balanced by length l of the potnetiometer wire. Then as per question,
In the first case,
In the second case,
Subtracting (ii) from (i), we get
or
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:PRADEEPChapter:CURRENT ELECTRICITYExercise:Conceptual Problems
Explore 3 Videos
- CURRENT ELECTRICITYBook:PRADEEPChapter:CURRENT ELECTRICITYExercise:Very short Q/A
Explore 7 Videos
- COMMUNICATION SYSTEMSBook:PRADEEPChapter:COMMUNICATION SYSTEMSExercise:MODEL TEST PAPER-2
Explore 9 Videos
- DUAL NATURE OF RADIATION AND MATTERBook:PRADEEPChapter:DUAL NATURE OF RADIATION AND MATTERExercise:Exercise
Explore 191 Videos
Similar Questions
When a resistor of 5Ω is connected across a cell, its terminal potential differnce is balanced by 140 cm of potentiometer wire and when a resistance of 8Ω is connected across the cell, the terminal potential difference is balanced by 160 cm of the potentiometer wire. Find the internal resistance of the cell.
View SolutionWhen a resistance of 12 ohm is connected across a cell, its terminal potential difference is balanced by 120 cm length of potentiometer wire. When the resistance of 18 ohm is connected across the same cell, the balancing length is 150 cm. Find the balancing length when the cell is in open circuit. Also calculate the internal resistance of the cell.
View Solution
Knowledge Check
In the given figure, battery E is balanced on 55 cm length of potentiometer wire but when a resistance of 10Ω is connected in parallel with the battery then it balances on 50 cm length of the potentiometer wire then internal resistance r of the battery is
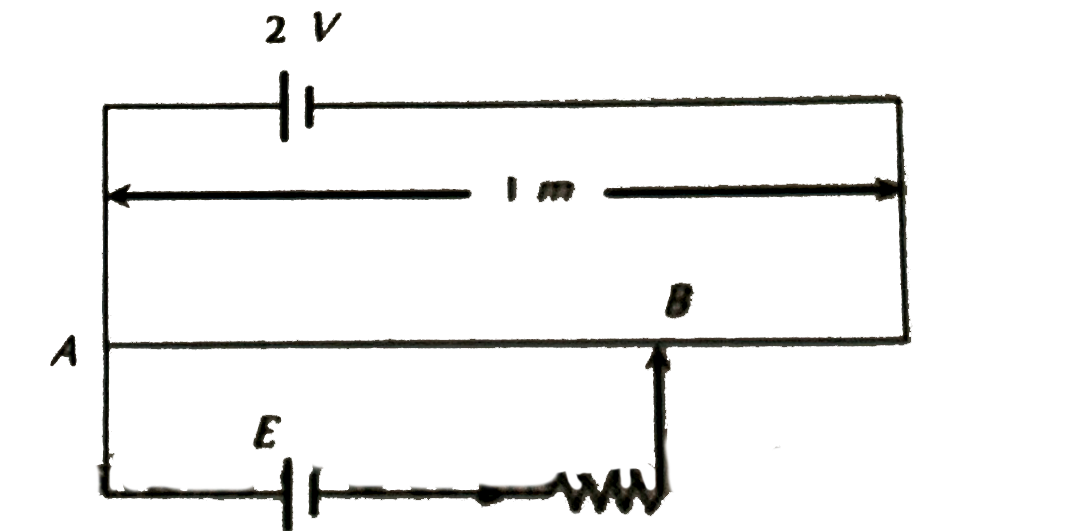 A1ΩB3ΩC10ΩD5Ω
A1ΩB3ΩC10ΩD5ΩIn fig. battery E is balanced on 55cm length of potentiometer wire but when a resistance of 10Ω is connected in parallel with the battery then it balances on 50cm length of the potentiometer wire then internal resistance r of the battery is:-
 A1ΩB3ΩC10ΩD5Ω
A1ΩB3ΩC10ΩD5ΩThe e.m.f. of a standard cell balances across 150 cm length of a wire of potentiometer. When a resistance of 2Ω is connected as a shunt with the cell, the balance point is obtained at 100cm . The internal resistance of the cell is
A0.1ΩB1ΩC2ΩD0.5Ω
The internal resistance of a cell is determined by using a potentiometer. In an experimetn, an external resistance of 60Ω is used across the given cell. When the key is closed, the balance length on the potentiometer decreases form 72 cm to 60 cm. calculate the internal resistance of the cell.
View SolutionIn an experiment with a potentiometer, the null point is
obtained at a distance of 60cm along the wire from the
common terminal with a leclanche cell. When a shunt
resistance of 1Ω is connected across the cell, the null
point shifts to a distance of 30cm from the common
terminal. what is the internal resistance of the cell?
View SolutionIn a potentiometer experiment the balancing length with a cell is 560 cm. When an external resistance of 10Ω is connected in parallel to the cell, the balancing length changes by 60 cm. Find the internal resistance of the cell.
View SolutionThe potential difference betwee11 the terminals of a cell in an open circuit is 2.2 V. When a resistor of 5Ω is connected across the terminals of the cell, the potential difference between the terminals of the cell is found to be 1.8 V. The internal resistance of the cell is
View SolutionA cell has an emf 1.5V. When connected across an external resistance of 2Ω, the terminal potential difference falls to 1.0V. The internal resistance of the cell is:
View Solution
