A U-tube is partially filled with water. Oil which does not mix with water is next poured into one side, until water rises by 25 cm on the other side. If the density of oil 0.8g/cm3. The oil level will stand higher than the water by
6.25cm
12.50cm
31.75cm
25cm
The correct Answer is:B
To solve the problem, we need to analyze the pressure balance in the U-tube when oil is poured into one side, causing the water level to rise on the other side.
1. Understand the Setup:
- We have a U-tube partially filled with water.
- When oil is poured into one side, the water level on the other side rises by 25 cm.
2. Identify the Variables:
- Let the density of oil, ρo=0.8g/cm3.
- The density of water, ρw=1g/cm3.
- The rise in water level, hw=25cm.
3. Pressure Balance:
- At the same horizontal level in the U-tube, the pressure must be equal.
- Let’s denote the height of the oil column as h.
4. Write the Pressure Equation:
- The pressure at the bottom of the oil column on the left side (point 1) is given by:
P1=P0+ρogh
- The pressure at the bottom of the water column on the right side (point 2) is given by:
P2=P0+ρwg(hw+h)
- Here, P0 is the atmospheric pressure, which cancels out in the equation.
5. Set the Pressures Equal:
- Since P1=P2:
ρogh=ρwg(hw+h)
6. Cancel g from Both Sides:
- This simplifies to:
ρoh=ρw(hw+h)
7. Substitute Known Values:
- Substitute ρo=0.8g/cm3 and ρw=1g/cm3:
0.8h=1(25+h)
8. Rearranging the Equation:
- Distributing the right side:
0.8h=25+h
- Rearranging gives:
0.8h−h=25
−0.2h=25
h=−250.2=−125cm
- Since height cannot be negative, we take the absolute value:
h=12.5cm
9. Conclusion:
- The oil level will stand higher than the water by 12.5cm.
- We have a U-tube partially filled with water.
- When oil is poured into one side, the water level on the other side rises by 25 cm.
- Let the density of oil, ρo=0.8g/cm3.
- The density of water, ρw=1g/cm3.
- The rise in water level, hw=25cm.
- At the same horizontal level in the U-tube, the pressure must be equal.
- Let’s denote the height of the oil column as h.
- The pressure at the bottom of the oil column on the left side (point 1) is given by:
P1=P0+ρogh
- The pressure at the bottom of the water column on the right side (point 2) is given by:
P2=P0+ρwg(hw+h)
- Here, P0 is the atmospheric pressure, which cancels out in the equation.
- Since P1=P2:
ρogh=ρwg(hw+h)
- This simplifies to:
ρoh=ρw(hw+h)
- Substitute ρo=0.8g/cm3 and ρw=1g/cm3:
0.8h=1(25+h)
- Distributing the right side:
0.8h=25+h
- Rearranging gives:
0.8h−h=25
−0.2h=25
h=−250.2=−125cm
- Since height cannot be negative, we take the absolute value:
h=12.5cm
- The oil level will stand higher than the water by 12.5cm.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Level 2 More Than One Correct
10 Videos
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Comprehension Based
7 Videos
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question
2 Videos
- EXPERIMENTSBOOK - DC PANDEYCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A U-tube is partially filled with mercury. Some water is poured in its one arm and a liquid in the other arm so that the level of mercury in both arms is same. What is the ratio of lengths of water and liquid in the two arms of the U-tube? Density of water = 1000 kg m−3 and density of liquid = 900 kg m−3
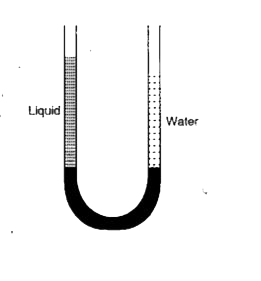
View SolutionA drop of olive oil of radius 0.25 mm spreads into a circular film of radius 5 cm on the water surface. Estimate the molecular size of the olive oil
View Solution
Knowledge Check
A U-tube is partially filled with water. Oil which does not mix with water is next poured into one side untill water rises by 25 cm on the other side. If the density of oil be 0.8, the oil level will stand higher then the water level by
A6.25cmB12.50 cmC31.75 cmD62.50 cmA U-tube is partially filled with water. Oil which does not mix with water is next poured into one side untill water rises by 25 cm on the other side. If the density of oil be 0.8, the oil level will stand higher then the water level by
A6.25 cmB12.50 cmC31.25 cmD20 cmA U-tube with both ends open to the atmosphere is partially filled with water. Oil, which is immiscible with water. Is poured into one side until it stands at a distance of 10mm above the water level on the other side. Meanwhile the water rises by 65mm from its original level (see diagram). The density of the oil is:
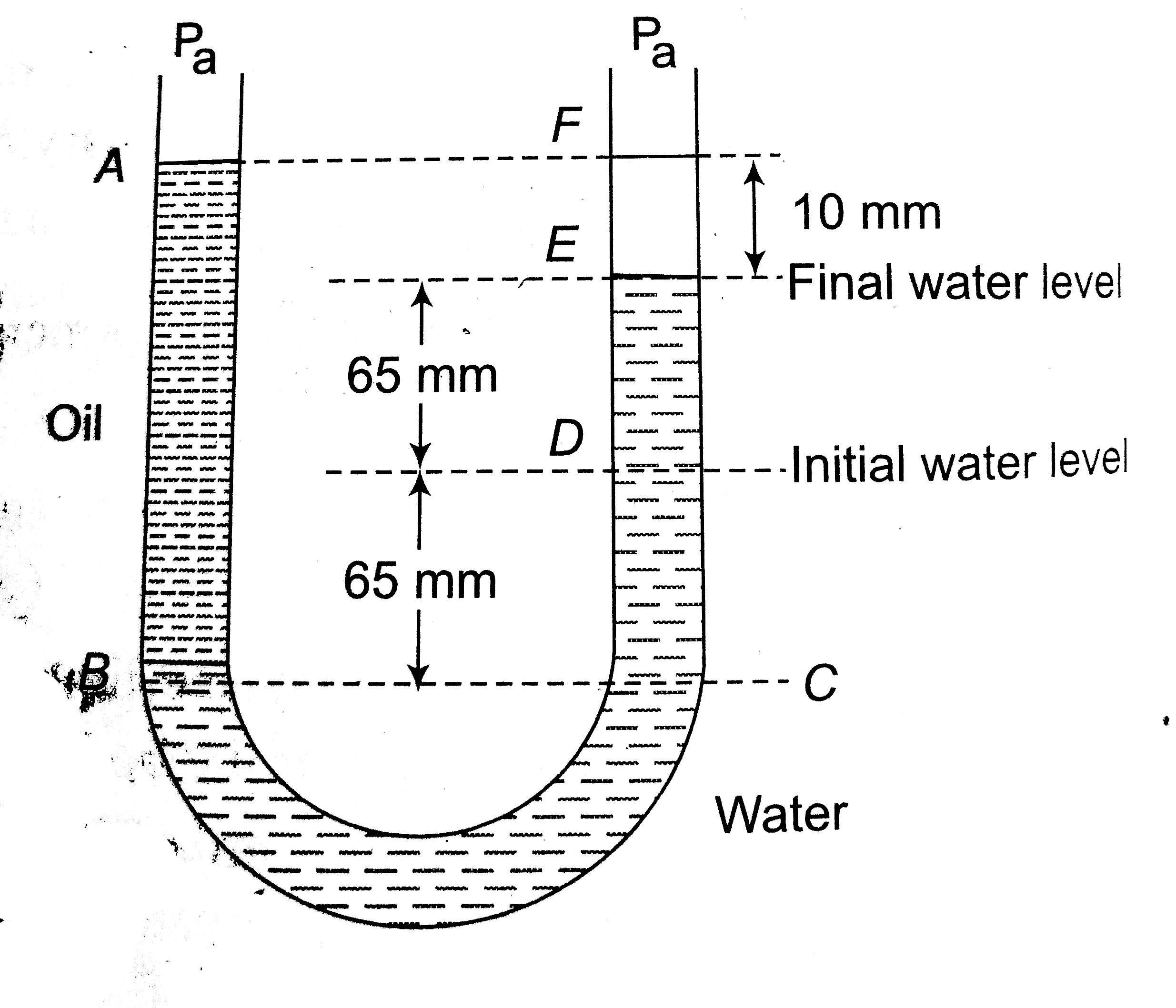 A425kgm−3B800kgm−3C928kgm−3D650kgm−3
A425kgm−3B800kgm−3C928kgm−3D650kgm−3
A U-tube with both ends open to the atmosphere is partially filled with water. Oil, which is immiscible with water. Is poured into one side until it stands at a distance of 10mm above the water level on the other side. Meanwhile the water rises by 65mm from its original level (see diagram). The density of the oil is:

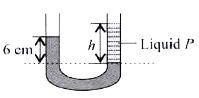
View SolutionThe diagram shows a U-tube used to determine the density of liquid P. When liquid P is poured into one arm, the water level in the other arm rises. If the densities of water and liquid P are 1000kg m−3 and 750kg m−3 respectively, then what is the value of h ?
View SolutionA cubical block of wood 10 cm on a side floats at the interface between oil and water with its lower surface horizontal and 4 cm below the interface. The density of oil is 0.6gcm−3. The mass of block is
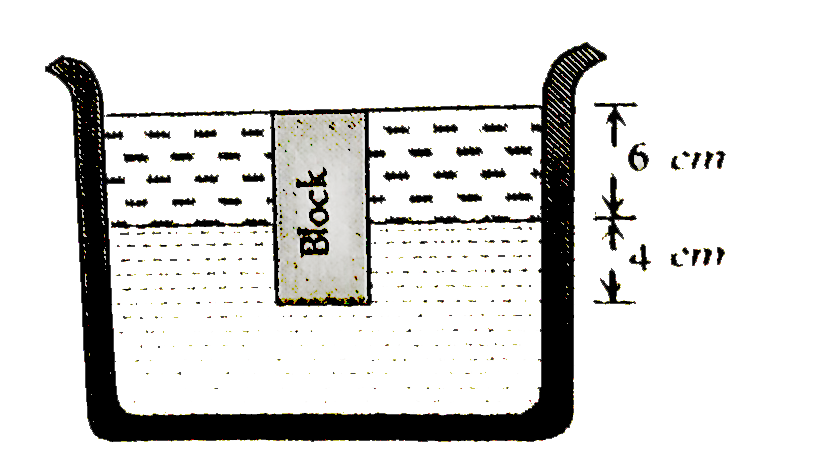
View SolutionA cubical block of wood 10cm on a side floats at the interface between oil and water, as in fig. with its lower face 2cm below the interface. The intensity of the oli is 0.6gcm−3. The mass of the block is 1kg

View SolutionA tank 5 m high is half filled with water and then is filled to the top with oil of density 0.85 g The pressure at the bottom of the tank, due to these liquids is
View Solution
