A system of wedge and block as shown in figure, is released with the spring in its natural length. All surfaces are frictionless. Maximum elongation in the spring will be
 .
.
2mgsinθK
mgsinθK
4mgsinθK
mgsinθ2K
The correct Answer is:A
Decrease in gravitational potential energy of block
= increase in spring potential energy
∴mg(xmsinθ)=12Kx2m
∴Xm=2mgsinθK
Topper's Solved these Questions
- WORK, ENERGY & POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 1 subjective
27 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 objective
30 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 1 Assertion and Reason
12 Videos
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
The block of mass m is released when the spring was in its natrual length. Spring constant is k. Find the maximum elongation of the spring.

View SolutionTwo blocks of mass m and 2m are connected to a spring and wrapped around a smooth pulley as shown in the figure. Blocks are released together with spring in natural length. Calculate the maximum elongation (in meter) of the spring. Given mgK=3 meter.
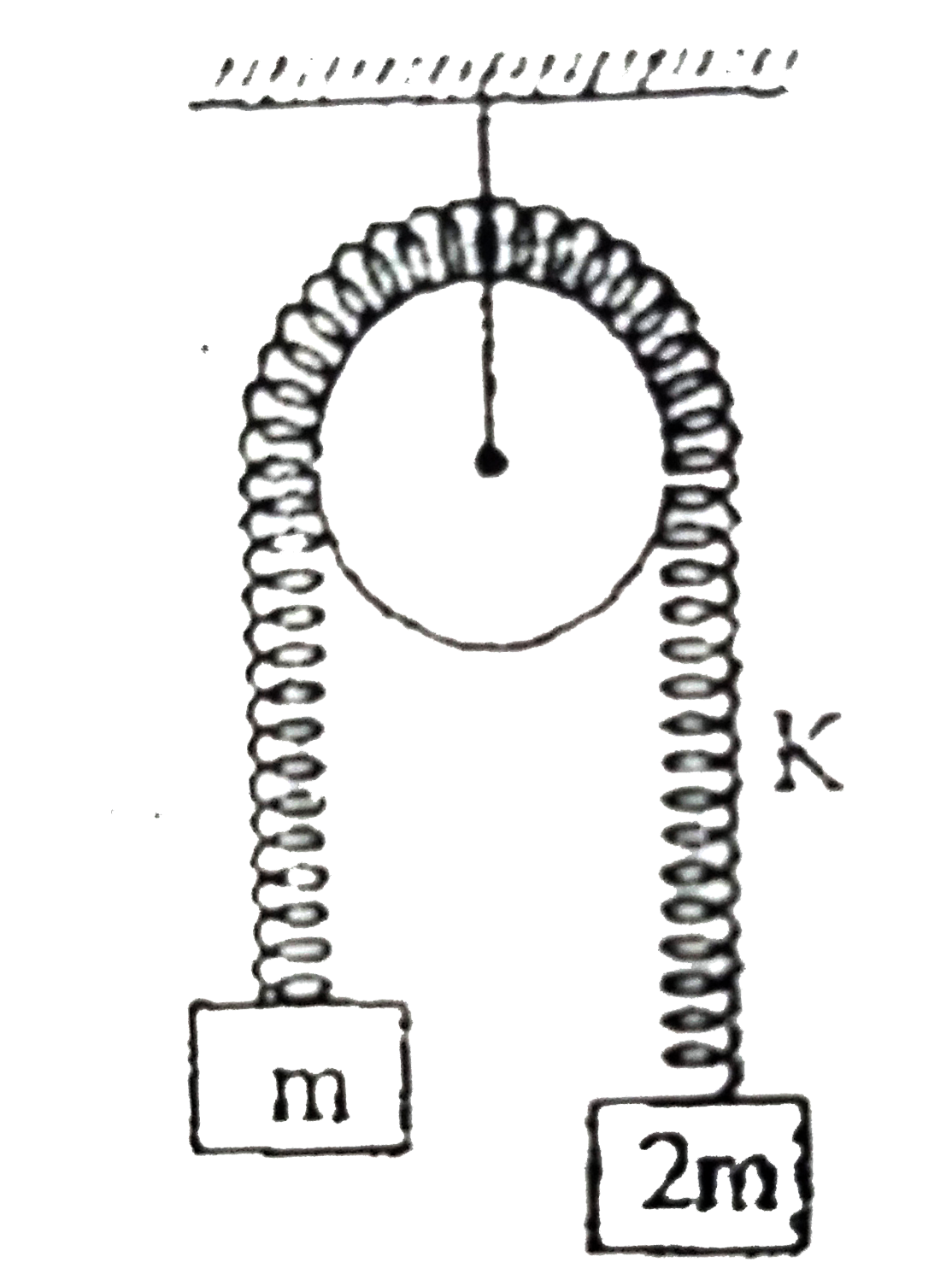
View Solution
Knowledge Check
The system of the wedge and the block connected by a massless spring as shown in the figure is released with the spring in its natural length. Friction is absent. Maximum elongation in the spring will be
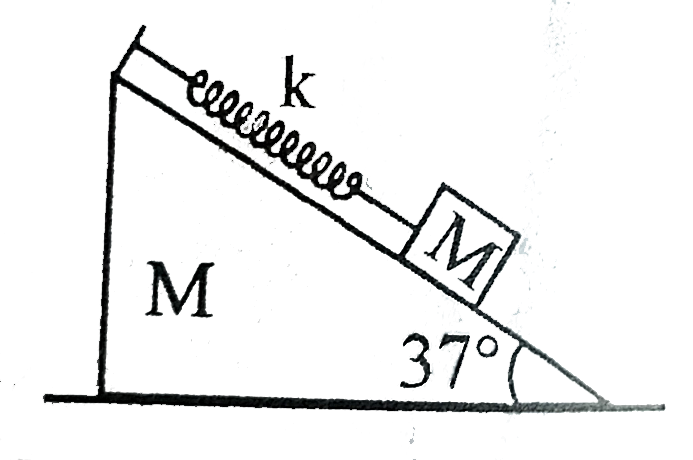 A3Mg5kB6Mg5kC4Mg5kD8Mg5k
A3Mg5kB6Mg5kC4Mg5kD8Mg5kThe system of block and wedges all of same mass shown in the figure is released from rest. All surfaces are smooth. Then:
 AThe speed of block A when it falls 50cm is 2√2m/sBThe speed of block B when A falls 50cm is 4m/sCThe speed of block B when A falls 50cm is √10m/sDThe speed of block C when A falls 50cm is √2m/s
AThe speed of block A when it falls 50cm is 2√2m/sBThe speed of block B when A falls 50cm is 4m/sCThe speed of block B when A falls 50cm is √10m/sDThe speed of block C when A falls 50cm is √2m/sAll surfaces shown in figure are smooth. System is released with the spring instretched. In equilibrium, compression in the spring will be
 A2mgkB(M+m)g√2kCmg√2kDmgk
A2mgkB(M+m)g√2kCmg√2kDmgk
The block shown in figure is released from rest and initially the spring is at its natural length. Write down the energy conservation equation. When the spring is compressed by l1?
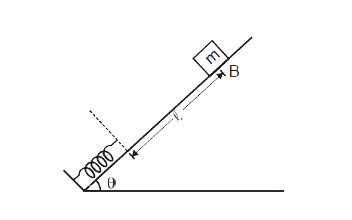
View SolutionBlock of mass 2m is given v0 towards the right. If L is the natural length of spring constant k, find the maximum elongation of the spring.

View SolutionAll surfaces shown in figure are smooth. System is released with the spring unstretched. In equilibrium, compression in the spring will be

View SolutionInitially mass m is held such that spring is in relaxed condition. If mass m is suddenly released, maximum elongation in spring will be
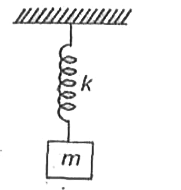
View SolutionA block of mass m is placed on a smooth block of mass M=m with the help of a spring as shown in the figure. A velocity v0 is given to upper block when spring is in natural length. Find maximum compression in the spring.
View Solution