In the given system, when the ball of mass m is released, it will swing down the dotted arc.
a. How fast will it reach the lowest point in its swing? A nail is located at a distance d below the point of suspension.
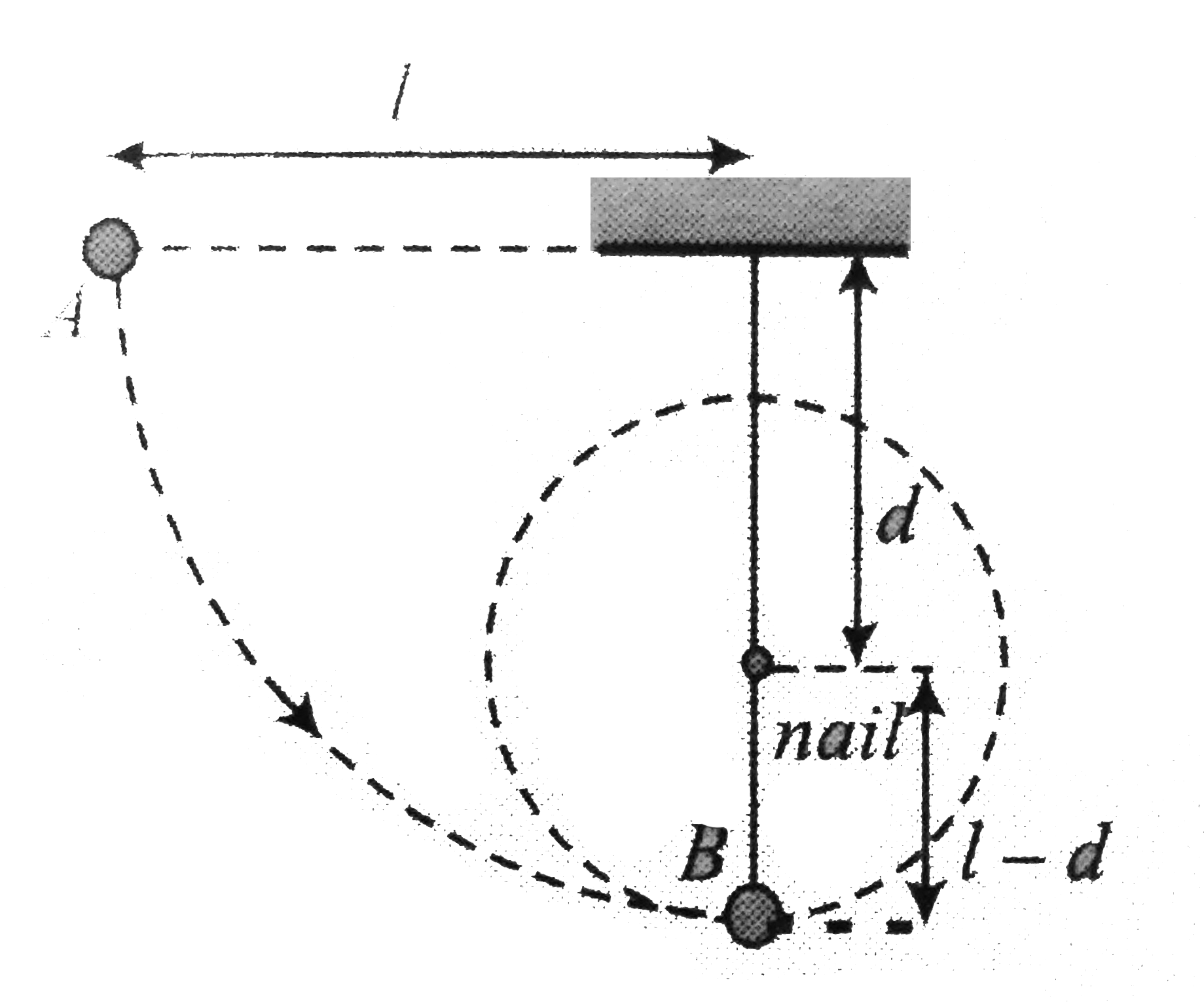
b. Show that d must at 0.6l, if the ball is to swing completely around a circle centered along the nail.
c. If d=0.6l, find the change in tension in the string just after it touches the nail.
a. Radius of the circle at nail =l−d. To complete the circle centered at nail, the speed at the bottom must be at least =√5g(l−d)
From A to B conserving mechanical energy ΔK+ΔU=0:
Loss in GPE=Gain in KE
mgl=12mv2⇒v=√2gl
b. To complete the circle: √2gl=√5g(l−d)
⇒5l−5d=2limpliesd=35l=0.6l
c. Just before touching the nail, the ball is moving in a circle of radius l.
⇒Tbefore−mg=mv2l
=mg+mv2l=mg+2mg=3mg

Just after touching the nail, the ball is moving in a circle of radius (l−d).
Tafter−mg=mv2(l−d)
=mg+mv2l−d=mg+m(2gl)0.4l
⇒Tension=6mg
Hence, the tension in the string changes from 3mg to 6mg as it touches the nail.
Related Playlists
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Solved Examples15videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.125videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
Versatile anthers which are able to swing freely are found in
View Solutionwhen the bob of a simple pendulum swings, the work done by tension in the string is :
View SolutionA small ball of mass m is released from rest from the position shown . All contact surface are smooth . The speed of the ball when it reaches its lowest position is
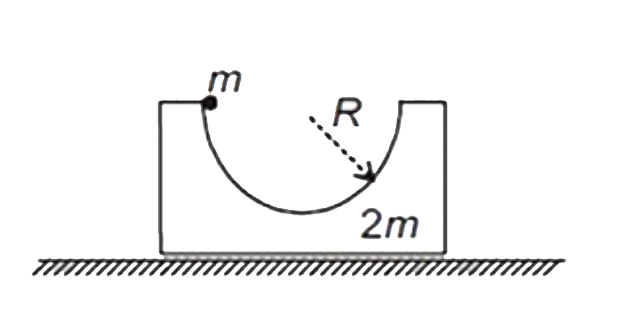 View Solution
View SolutionA small ball of mass m is released from rest from the position shown . All contact surface are smooth . The speed of the ball when it reaches its lowest position is
 View Solution
View SolutionA ball of mass m is fastened to a string. The ball swings in a vertical circle of radius R with the other end of the string held fixed. Neglecting the air resistance, the difference between the string's tension at the bottom of the circle and at the quarter of the cirlce is
View SolutionA ball of mass m is released from A inside a smooth wedge of mass m as shown in figure. What is the speed of the wedge when the ball reaches point B?
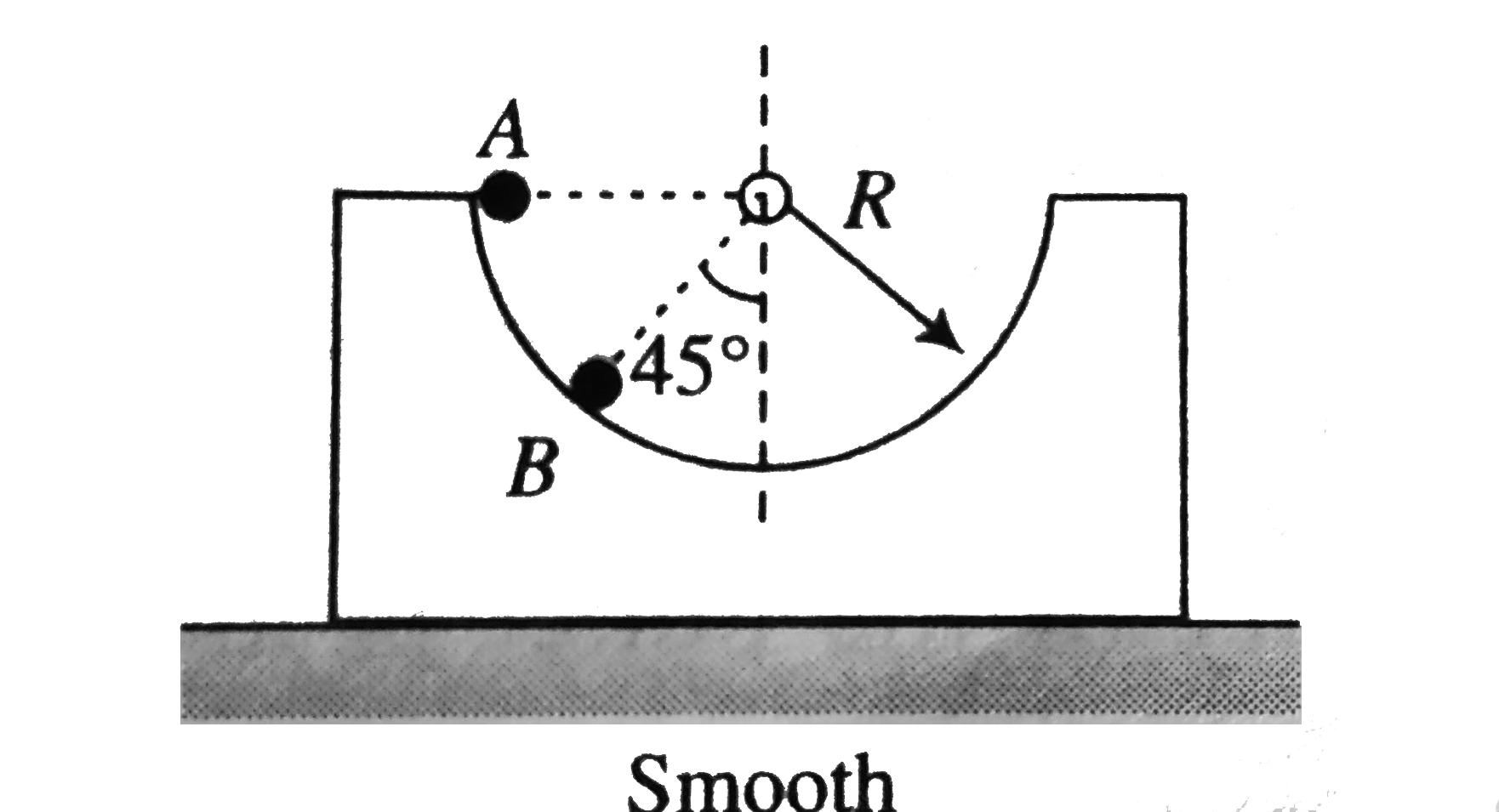 View Solution
View SolutionA ball is attached to a horizontal cord of length l whose other end is fixed. (a) If the ball is released, what will be its speed at the lowest point of its path? (b) A peg is located a distance h directly below the point of attachment of the cord. If h=0.75l, what will be the speed of the ball when it reaches the top of its circular path about the peg?
View SolutionIf a circle passes through the point (0,0),(a,0)and(0,b) , then find its center.
View SolutionIf a circle passes through the point (0,0),(a,0)and(0,b) , then find its center.
View SolutionA simple pendulum of length L and mass m has a spring of force constant k connected to it at a distance h below its point of suspension. Find the frequency of vibrations of the system for small values of amplitude.
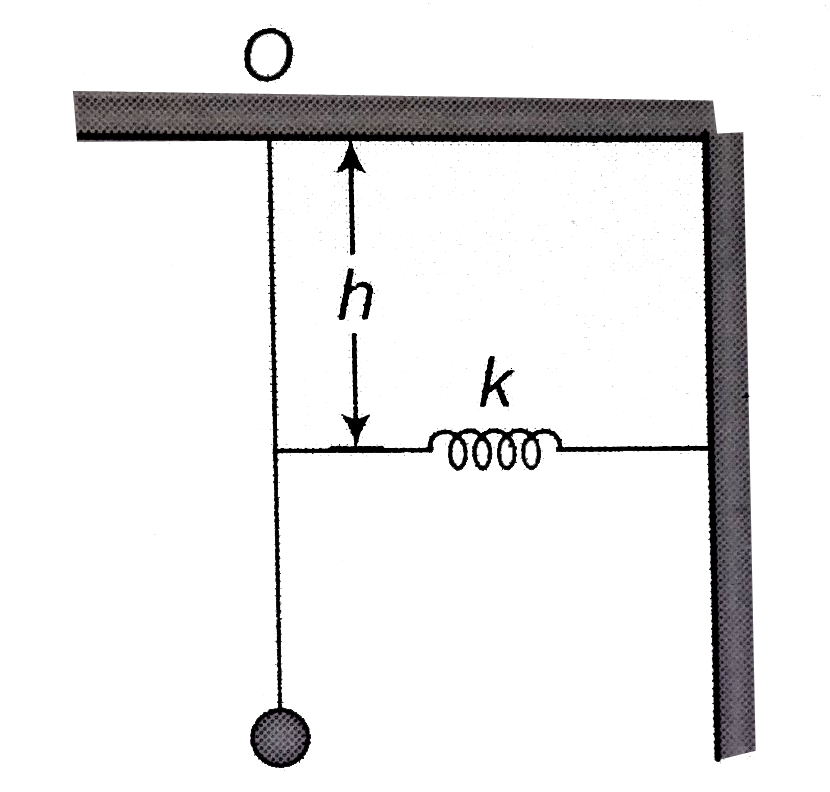 View Solution
View Solution


