Column I gives a listof possible set of parameters measured in some experiments. The variations of the parameters in the form of graphs are shown in Column II. Match the set of parameters given in Column I with the graphs even in Column II. Indicate your answer by darkening the appropriate bubbles of the 4×4 matrix given in the ORS.
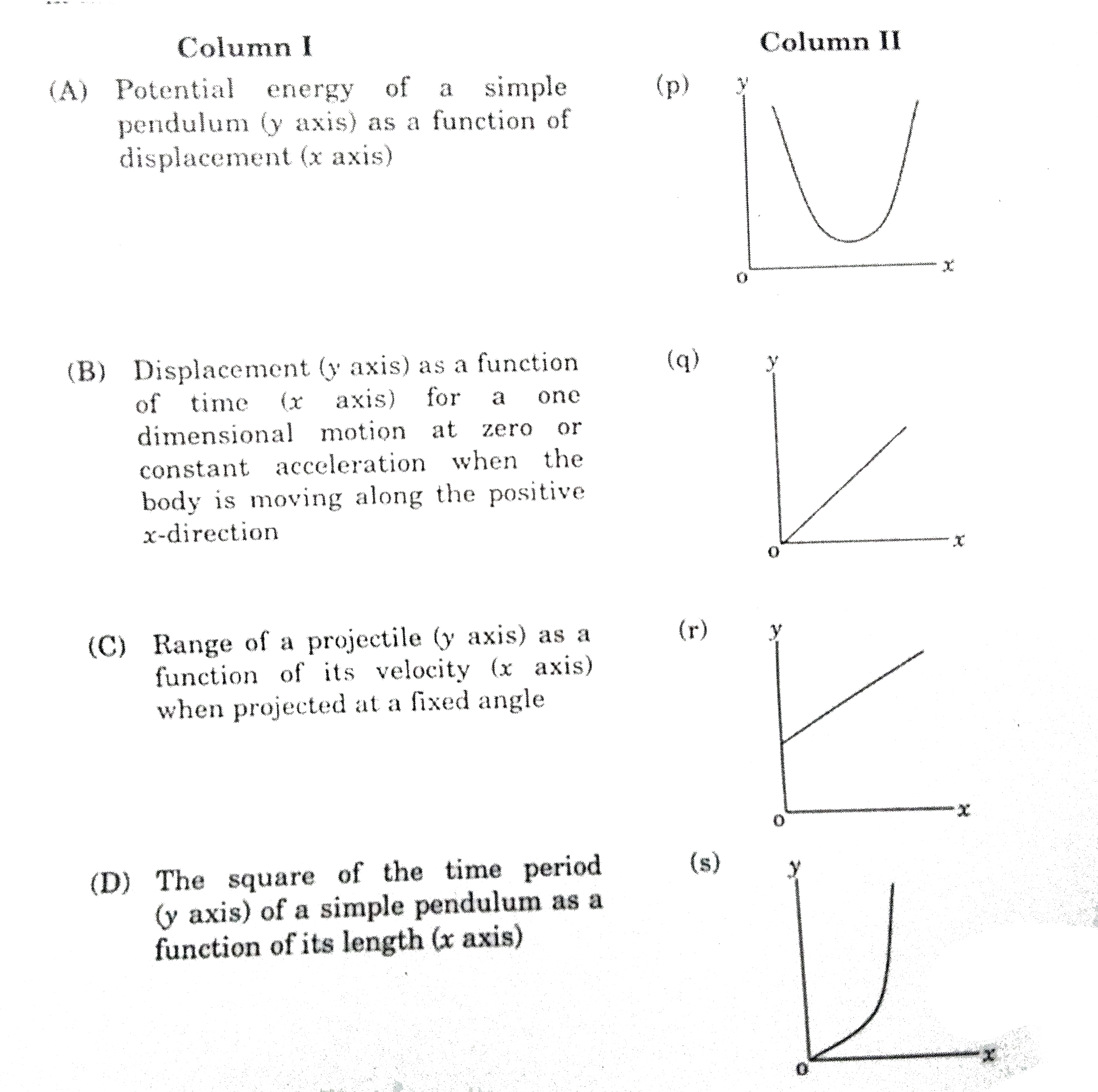
The correct Answer is:A-P,S;B-Q,S;C-S;D-Q
A-P,S,B-Q,S,C-S,D-Q
(A) Potential energy is minimum at mean position. (B) For a=constant, s=ut+12at)(2) -option(S)
( C) R=v2sin2θg therefore R∝v2-option (S)
T=2π√lg because T2∝l-option(Q)
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 6-previous year question56videos
SIMPLE HARMONIC MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (True/False Type)3videos
ROTATIONAL MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F10videos
SYSTEM OF A PARTICLES & ROTATIONAL MOTION
Similar Questions
Match the properties given in Column I with the metals given in Column II.
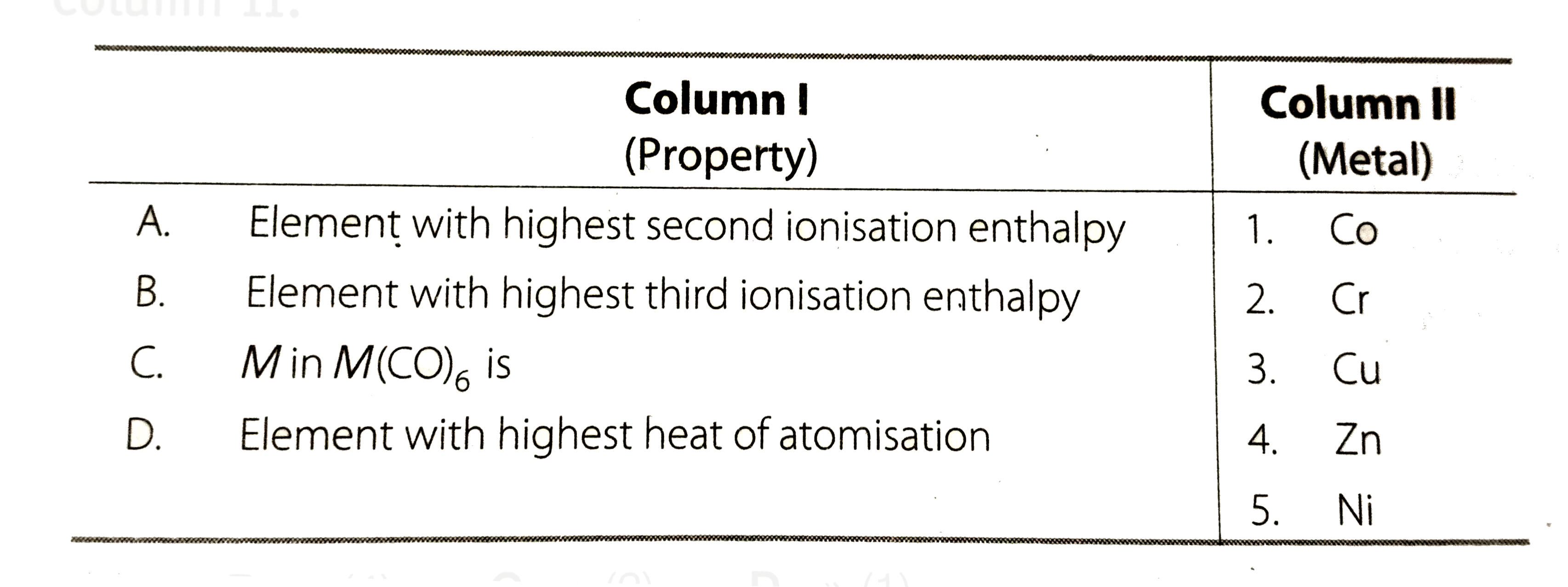 View Solution
View SolutionMatch the classes of drugs given in Column I with their action given in Column II.
 View Solution
View SolutionMatch the type of packing given in column I with the iterms given in column II.
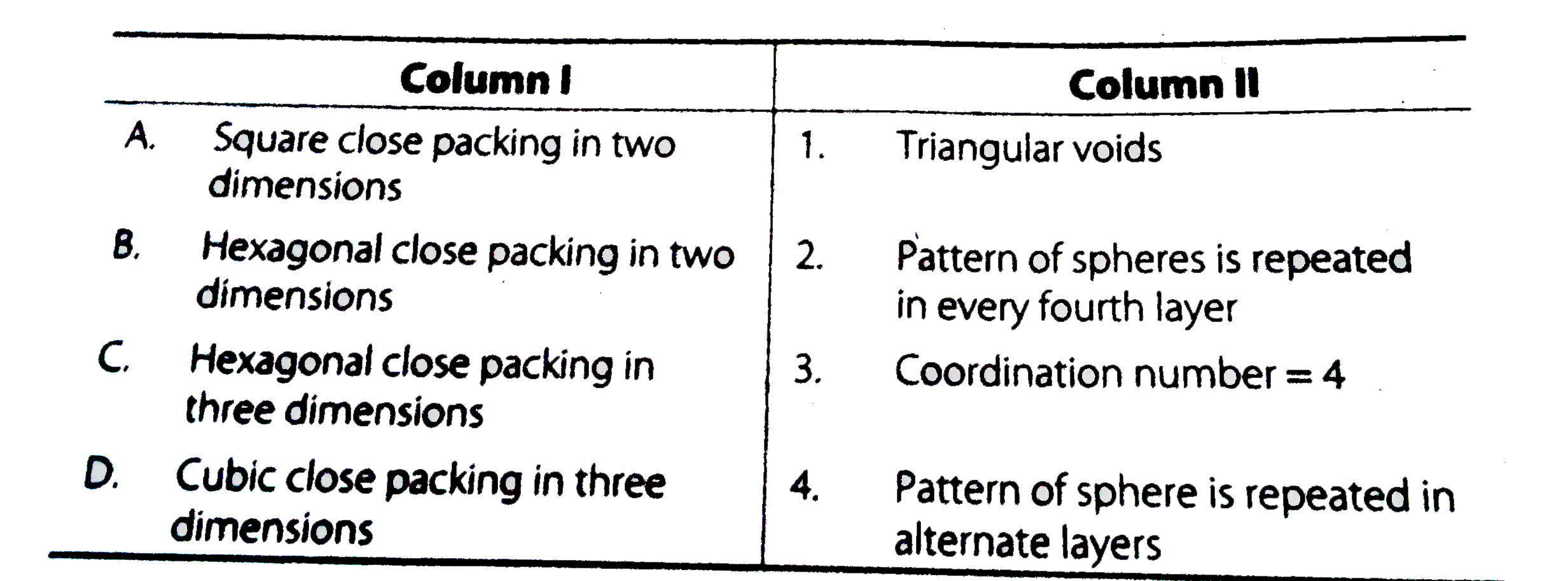 View Solution
View SolutionMatch the properties given in Column I with the metals given in Column II
 View Solution
View SolutionMatch the type of packing given in column I with the iterms given in column II.
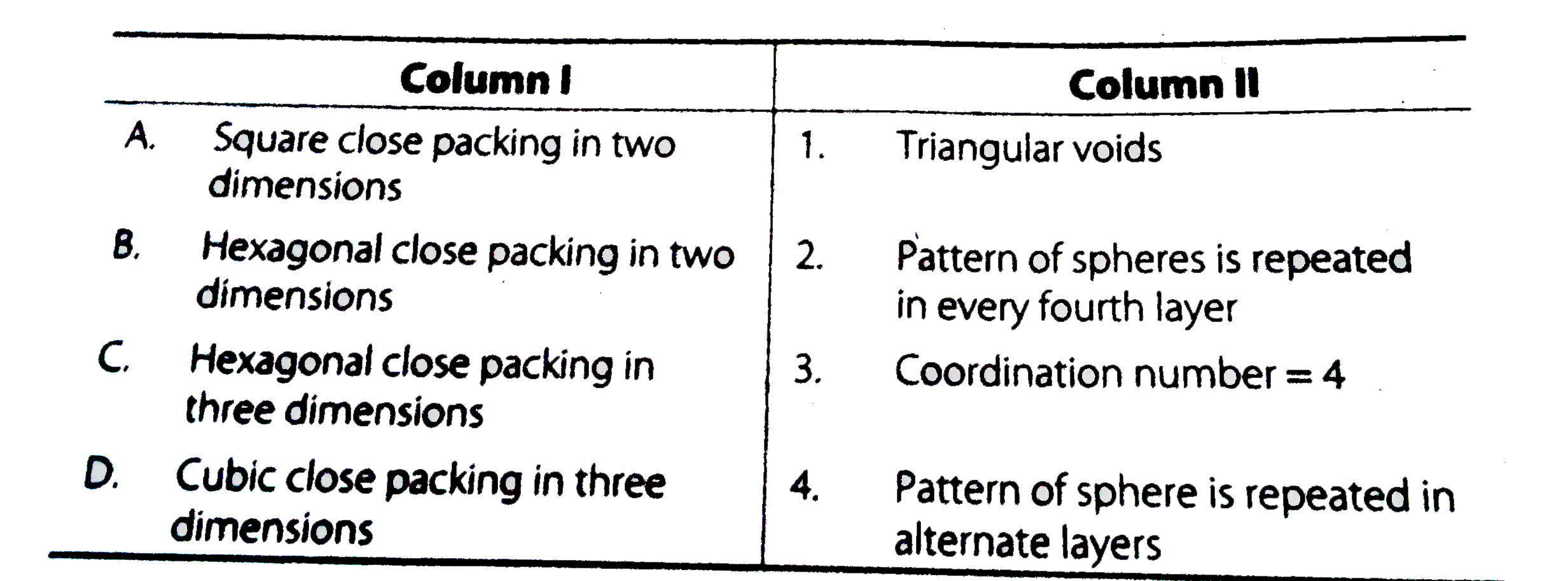 View Solution
View SolutionMatch the reactions given in Column I with the statements given in Column II
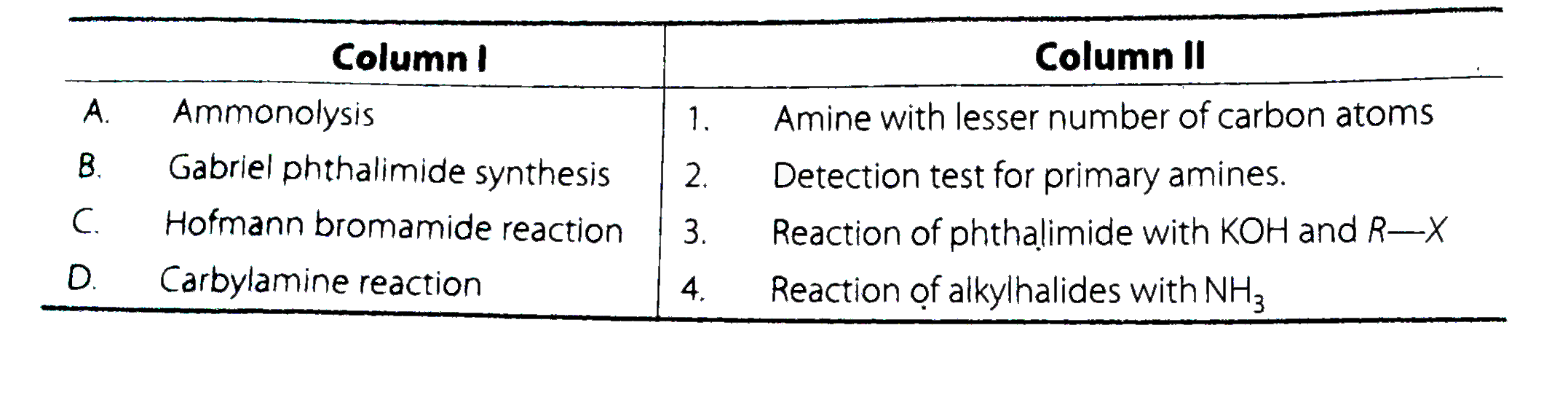 View Solution
View SolutionMatch the reactions given in Column I with the statements given in Column II
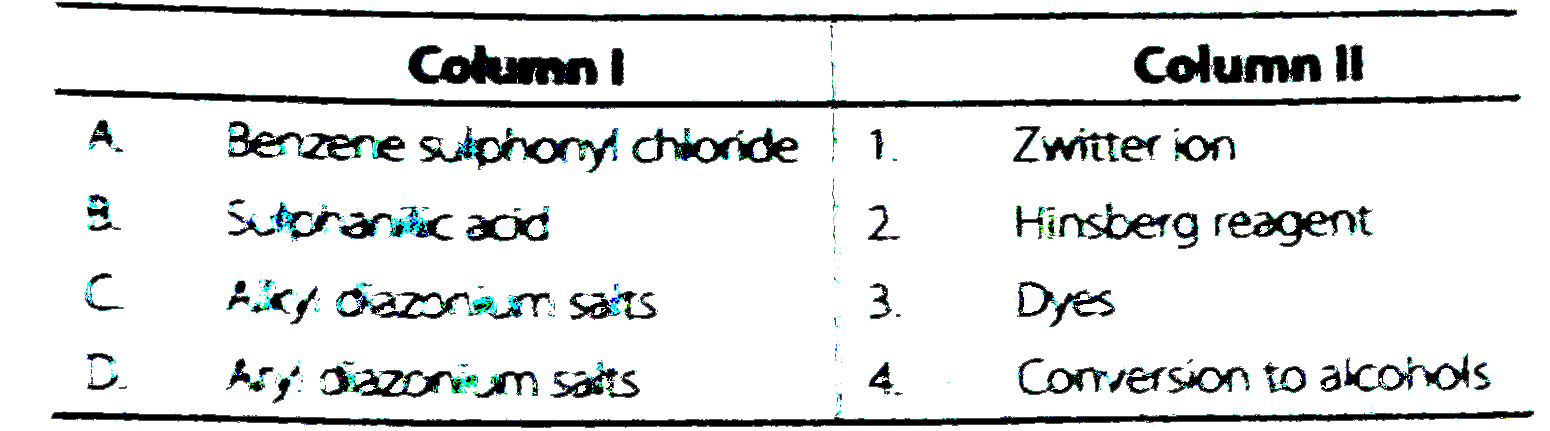 View Solution
View SolutionMatch the terms given in Column I with the compound given in Column II.
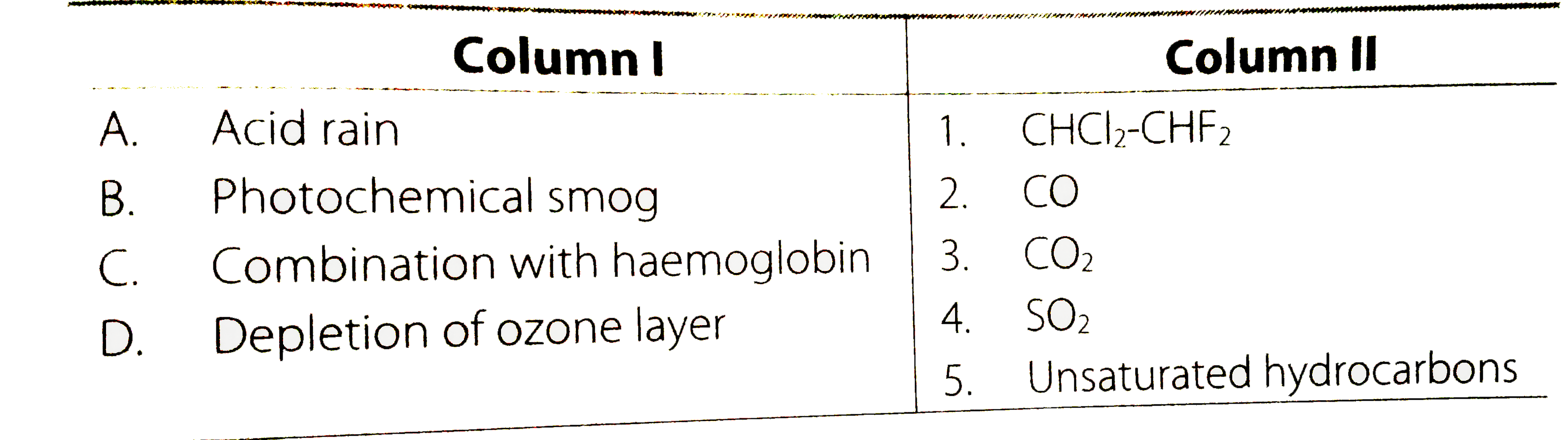 View Solution
View SolutionMatch the reactions given in Column I with the names given in Column II.
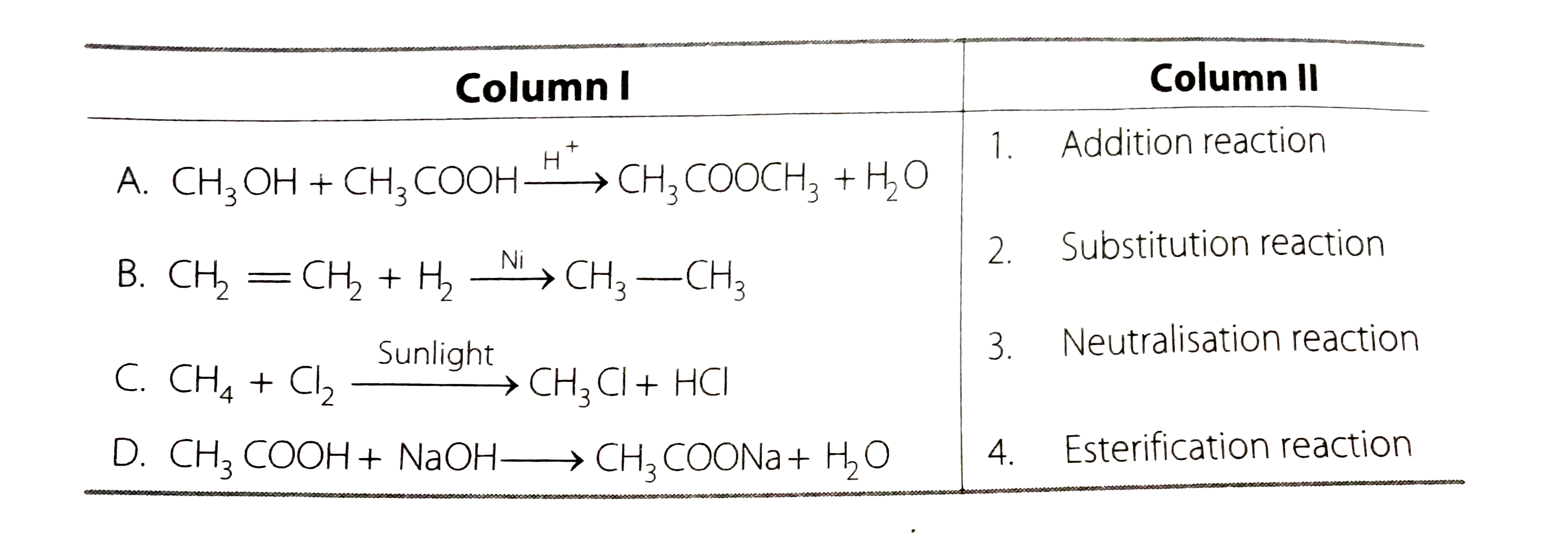 View Solution
View SolutionMatch the terms given in column I with the units given in column II.
 View Solution
View Solution
