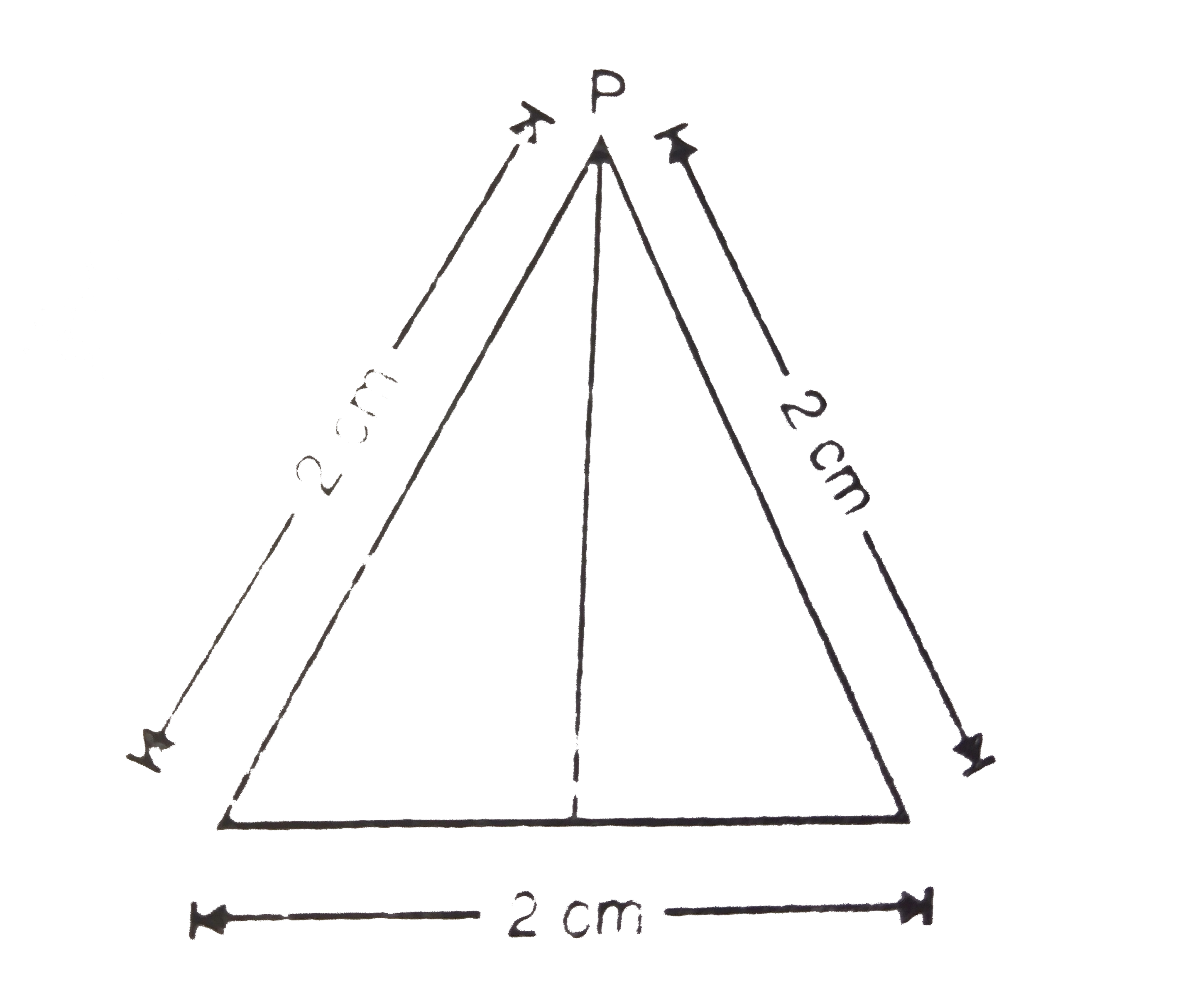
Two parallel wires carry equal currents of 10A along the same direction and are separated by a distance of 2.0 cm. Find the magnetic field at a point which is 2.0 cm away from each of these wires.
Required magnetic field at P,=
⎷(2×10−7×102×10−2)2+(2×10−7×102×10−2)2+(2×10−7×102×10−2)cos60∘
.= sqrt((10 ^()-4)^2 +(10^(-4))^2 + 2(10^(-4))(10^(-4))xx (1)/(2)<br>. =√3×10−4T
.=1.732xx10^(-4)T.
Related Playlists
- BOOK - HC VERMACHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Objective 27videos
MAGNETIC FIELD DUE TO CURRENT
- BOOK - HC VERMACHAPTER - MAGNETIC FIELDEXERCISE - Exercises61videos
MAGNETIC FIELD
- BOOK - HC VERMACHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Exercises9videos
MAGNETIC PROPERTIES OF MATTER
Similar Questions
Two long parallel wires carry equal current I flowing in the same direction are at a distance 2d apart. The magnetic field B at a point lying on the perpendicular line joining the wires and at a distance x from the midpoint is
View SolutionTwo long parallel wires carry equal current I flowing in the same direction are at a distance 2d apart. The magnetic field B at a point lying on the perpendicular line joining the wires and at a distance x from the midpoint is
View SolutionTwo long and parallel straight wire A and B carrying currents of 8.0 A and 5.0 A in the same direction are separated by a distance of 4.0 cm. Estimate the force on a 10 cm section of wire A.
View SolutionTwo infinitely long straight parallel wires carry equal currents 'I' in the same direction and are 2 m apart. Magnetic induction at a point which is at same normal distance (√2)m from each wire has magnitude
View SolutionTwo long and parallel straight wires A and B carrying currents of 8⋅0A and 5⋅0A in the same direction are separated by a distance of 4⋅0cm. Estimate the force on a 10cm section of wire A.
View SolutionTwo long and parallel straight wires A and B carrying currents of 0.8 A and 5.0 A in the same direction are separated by distance of 0.4 cm. What is the force on a 10 cm section of wire A ?
View SolutionEach of two long parallel wires carries a constant current i along the same direction . The wires are separated by a distance 2I. The magnitude of resultant magnetic induction in the symmetric plane of this system located between the wires at a distance R from each wire will be
View SolutionTwo long and parallel straight wires A and B are carrying currents of 4A and 7A in the same directions are separated by a distance of 5cm. The force acting on a 8cm section of wire A is
View SolutionTwo infinitely long wires each carrying current I along the same direction are made into the geometry as shown in the figure. The magnetic field at the point P is
 View Solution
View SolutionTwo long parallel copper wires carry currents of 5A each in opposite directions. If the wires are separated by a distance of 0.5m , then the force between the two wires is
View Solution


