Area of the region bounded by y2≤4x,x+y≤1,x≥0,y≥0 is a√2+b then value of a−b is
A
4
B
6
C
8
D
12
Video Solution
Text Solution
The correct Answer is:B
|
Answer
Step by step video, text & image solution for Area of the region bounded by y^(2)le4x,x+yle1,xge0,yge0 is asqrt(2)+b then value of a-b is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
The region in the xy plane given by y−x≤1,2x−6y≤3,x≥0,y≥0 is
AboundedBnot convexCunbounded convexDbounded and convexShaded, region of the constraints 4x+3y≤12,−x+y≤2,x≥0,y≥0 is
A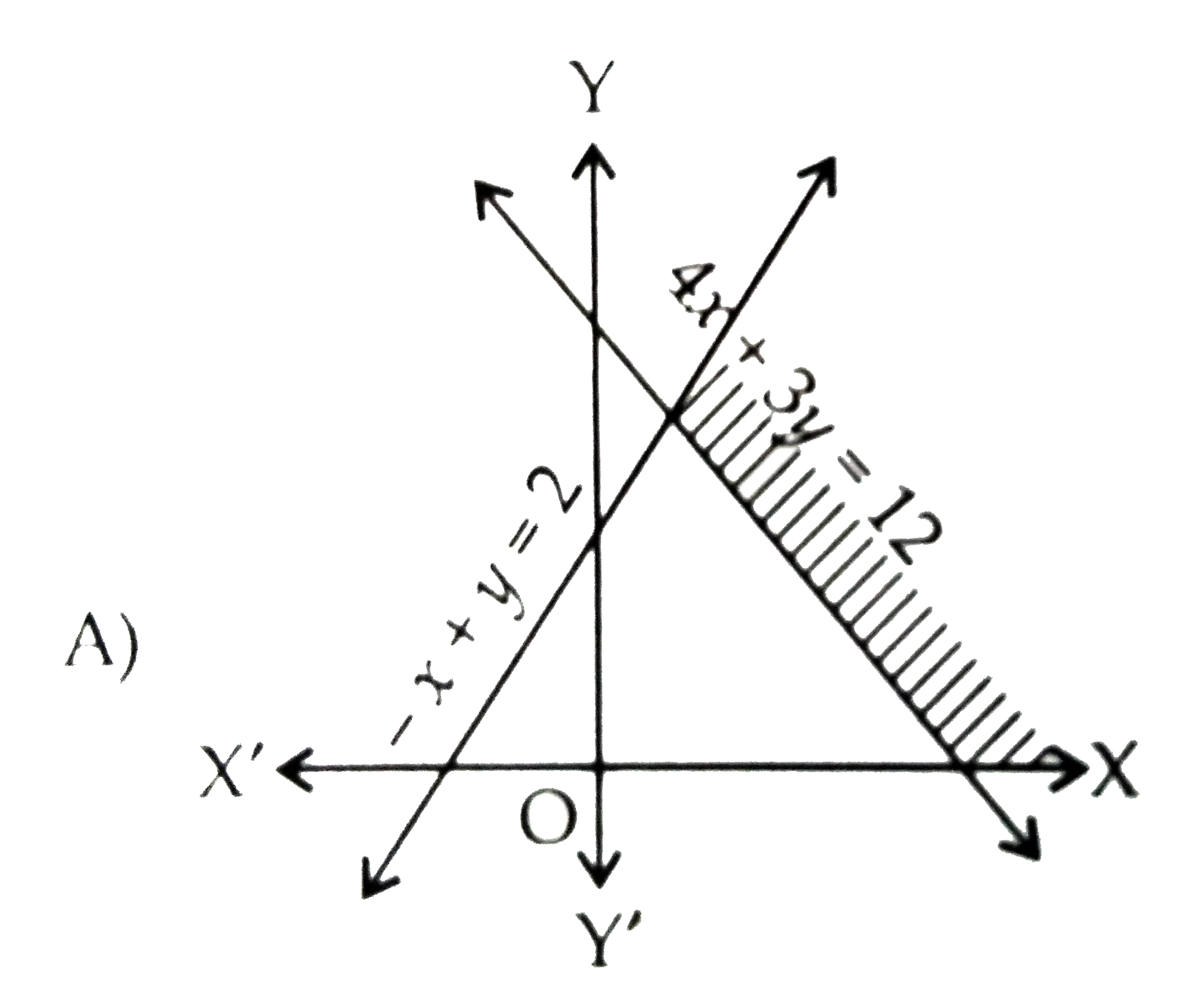 B
B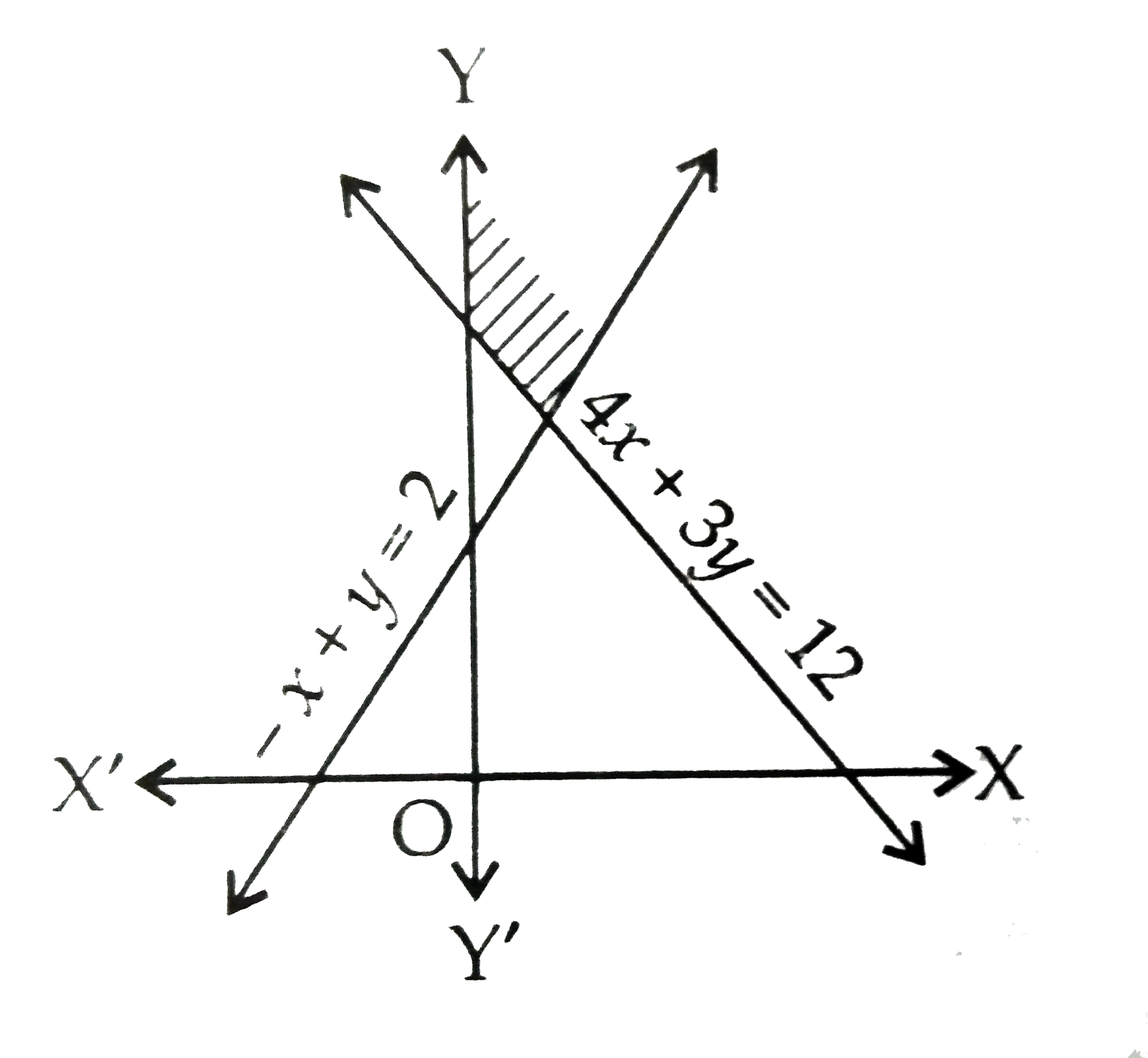 C
C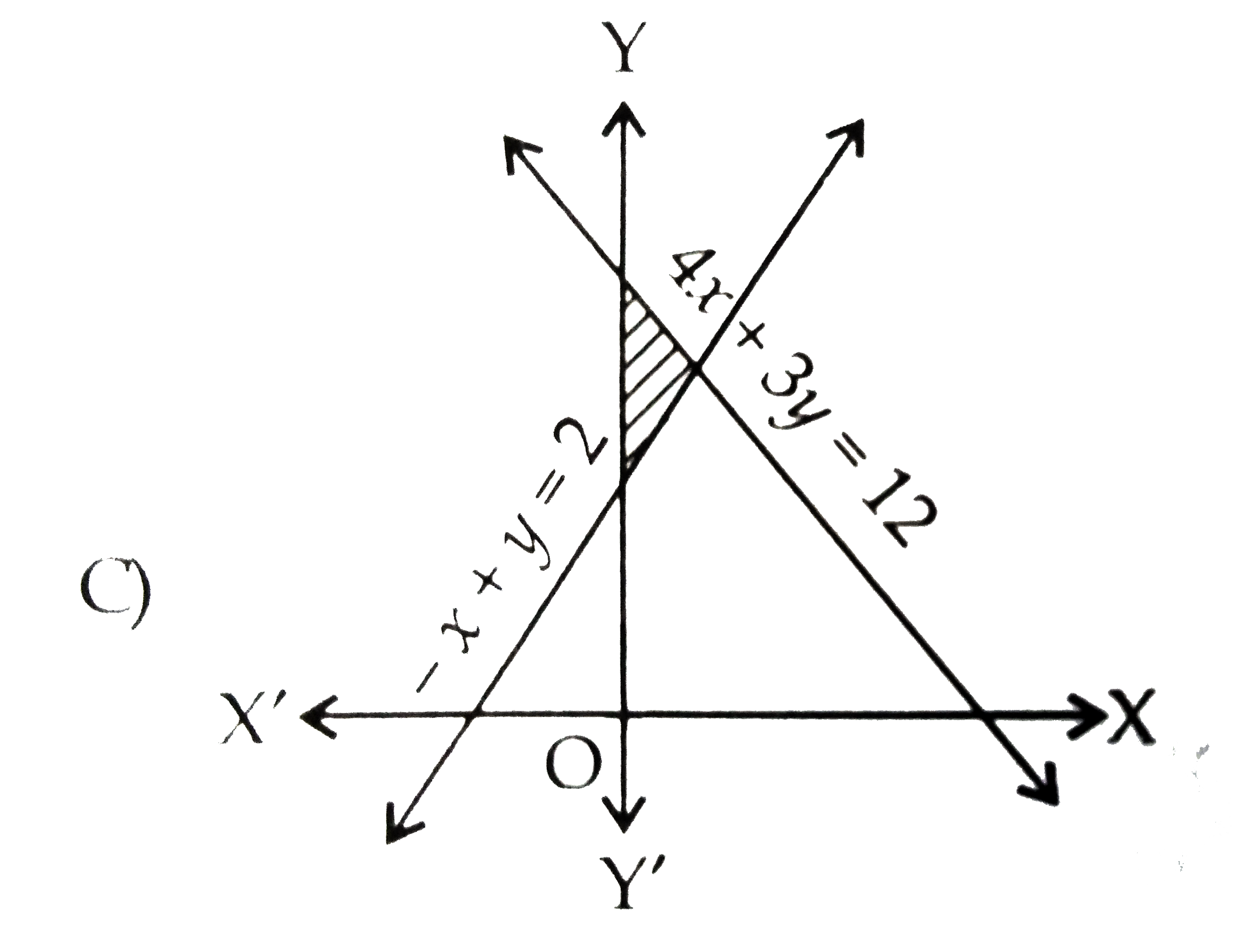 D
D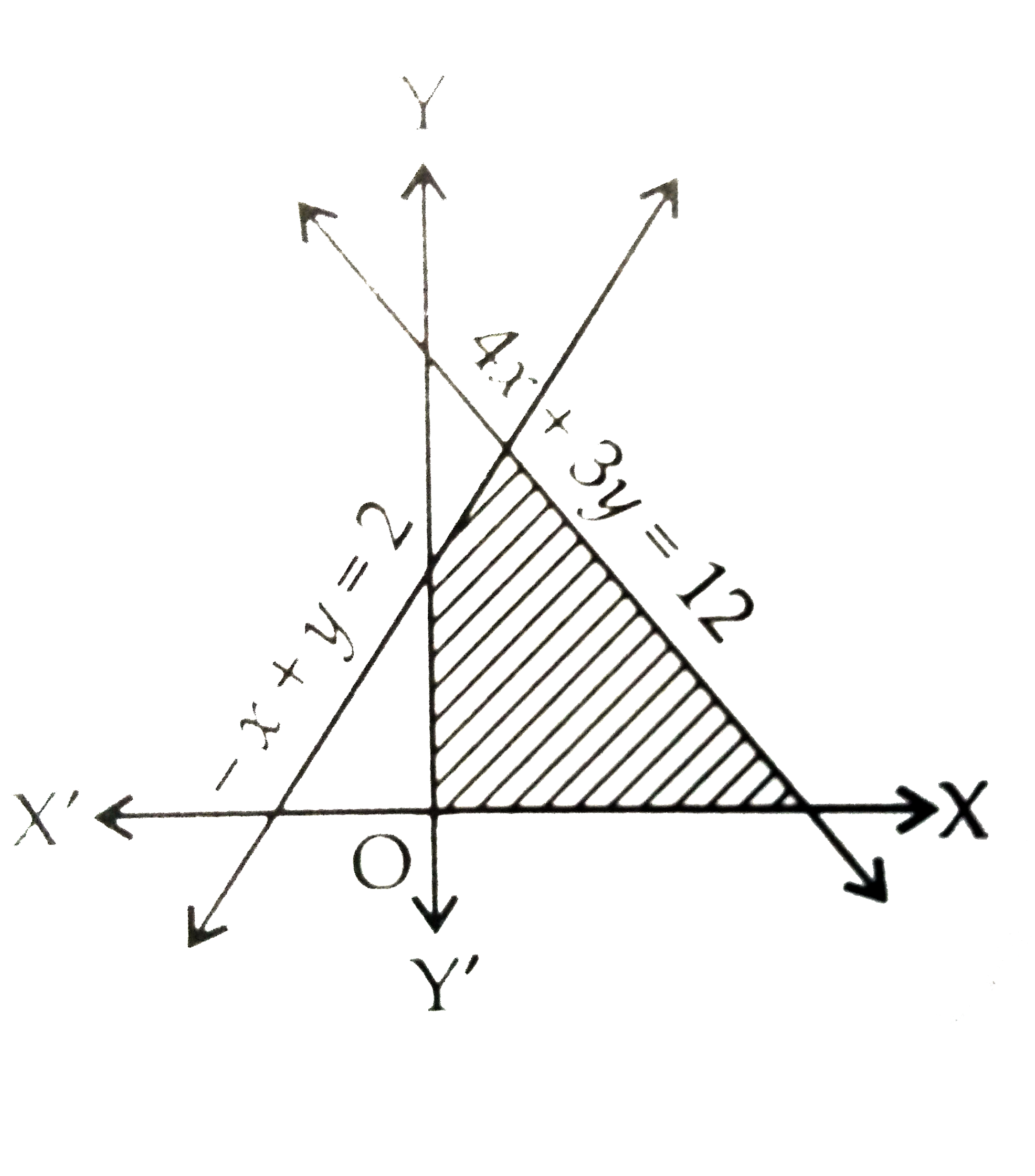
The vertex of the linear inequalities 2x+3y≤6,x+4y≤4,x≥0,y≥0 is
A(1,0)B(1,1)C125,25D25,125
The common region represented by inequalities y≤2,x+y≤3,−2x+y≤1,x≥0,y≥0 is
View SolutionThe constraints −x+y≤1,−x+3y≤9,x≥0,y≥0 defines
View SolutionThe lines 5x+4y≥20,x≤6,y≤4,x≥0,y≥0 form
View SolutionArea of the region bounded by the curve {(x,y):x2a2+y2b2≤1≤xa+yb} is
View SolutionThe region represented by the inequations 2x+3y≤18,x+y≥10,x≥0,y≤0 is
View Solution
