Select and write the corrcet answer :
The elastic potential energy per unit volume in a copper wire $(Y = 1.2 \times 10^{11} P a)$ stretched by $0.5 %$ of its length is

$1.5 \times 10^{10} J / m^{3}$

$1.5 \times 10^{8} J / m^{3}$

$1.5 \times 10^{7} J / m^{3}$

$1.5 \times 10^{6} J / m^{3}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, C, D

Updated on:21/07/2023

Two wires of same material and length but diameters in the ratio $1 : 2$ are streached by the same force, the elastic potential energy per unit volume for the two wires then strechted by the same force will be in the ratio
A $16 : 1$
B $1 : 1$
C $1 : 4$
D $4 : 1$
Figure shows the graph of elastic potential energy $(U)$ stored versus extension, for a steel wire $(Y = 2 \times 10^{11} P a)$ of volume $200 c c$ . If area of cross- section $A$ and original length L, then

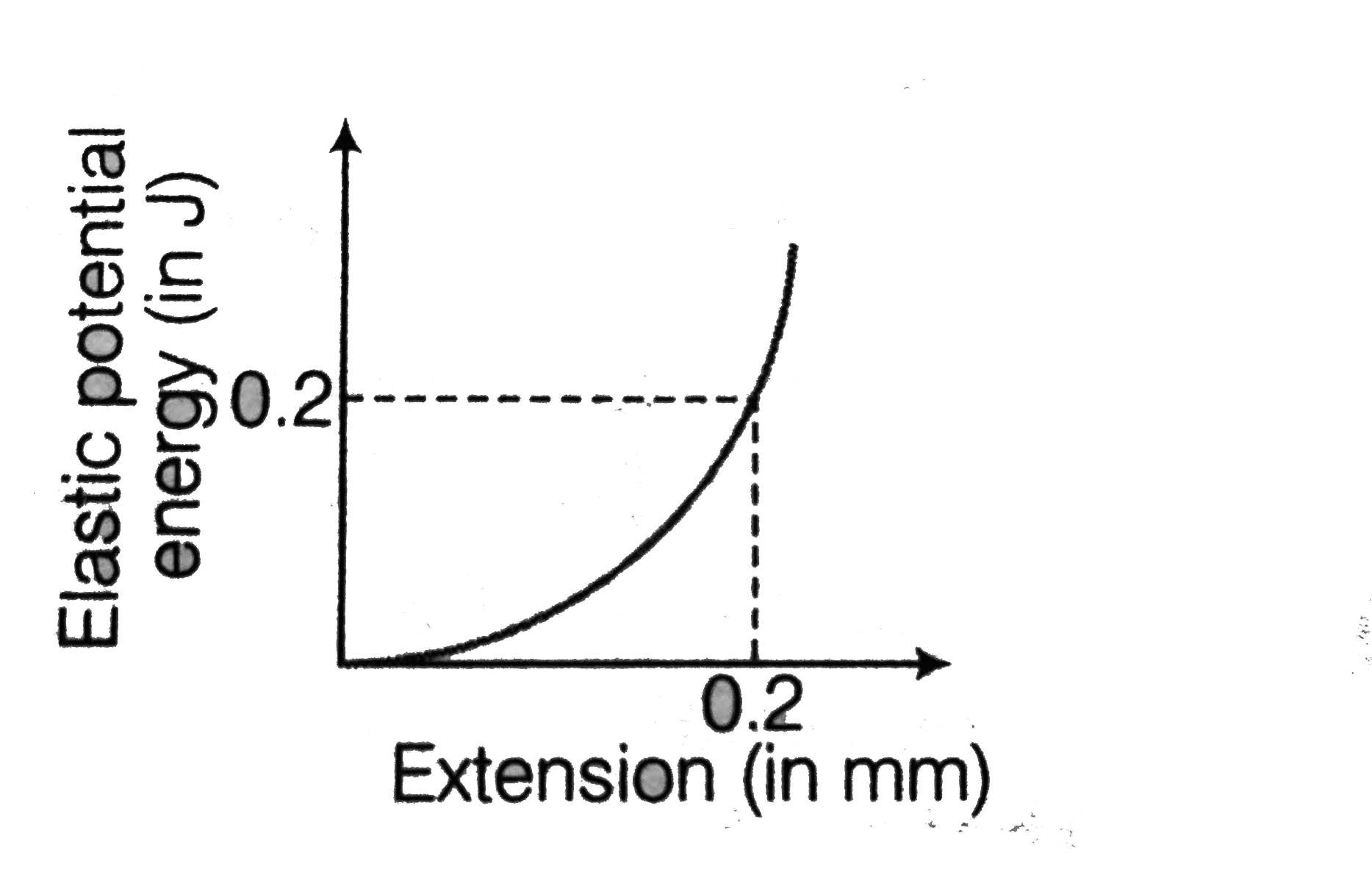A $4 m$
B $2 m$
C $4 c m$
D $2 c m$
Figure shows the graph of elastic potential energy $(U)$ stored versus extension, for a steel wire $(Y = 2 \times 10^{11} P a)$ of volume $200 c c$ . If area of cross- section $A$ and original length L, then

A $A = 10^{- 4} m^{2}$
B $A = 10^{- 3} m^{2}$
C $L = 1.5 m$
D $L = 2 m$