Three non - zero vectors ¯a,¯b,¯c are complanar if and only if there exist scalars x,y,z, not all zero simultaneously such that x¯a+y¯b+z¯c=¯0.
Let ¯a,¯b,¯c be coplanar vectors. Then any one of them, say ¯a, will be the linear combination of ¯band¯c.
∴ there exist scalars αandβ such that
¯a=α¯b+β¯c
∴(−1)¯a+α¯b+β¯c=¯0,i.e.,x¯a+y¯b+z¯c=¯0
where x=−1,y=α,z=β which are not all zero simultaneously.
Conversely : Let there exist scalars x,y,z not all zero such that
x¯a+y¯b+z¯c=¯0 . . . (1)
Let x≠0, then divide (1) by s, we get
i.e.,¯a+(yx)¯b+(zx)¯c=¯0
∴¯a=(−yx)¯b+(−zx)¯c
i.e.,¯a=α¯b+β¯c, where α=−yxandβ=−zx are scalars.
∴¯a is the linear combination of ¯band¯c.
Hence, ¯a,¯b,¯c are coplanar.
Topper's Solved these Questions
- VECTORSBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:VECTORSExercise:Examples for Practics
Explore 28 Videos
- VECTORSBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:VECTORSExercise:Multiple choice question
Explore 10 Videos
- VECTORSBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:VECTORSExercise:Multiple choice question
Explore 10 Videos
- TRIGONOMETRIC FUNCTIONS Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:TRIGONOMETRIC FUNCTIONS Exercise:Multiple Choice Questions
Explore 12 Videos
Similar Questions
If ¯a,¯b,¯c are non-coplanar vectors and x, y, z are scalars such that ¯a=x(¯bׯc)+y(¯cׯa)+z(¯aׯb) then x =
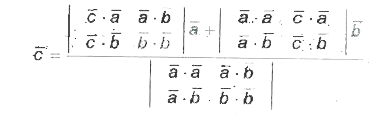
View Solution¯a,¯b,¯c are three coplanar vectors, If ¯a is not parallel to ¯b, Show that
View SolutionIf →a is perpendicular to →b and p is non zero scalar such that p¯r+(¯r.¯b)¯a=¯c
View SolutionThree vectors ¯¯¯A,¯¯¯Band¯¯¯C add up to zero. Find which is false.
View Solutionparallel to the vector ¯b is ¯r=¯a+λ¯b , where λ is a scalar.
View SolutionFor non -zero vectors a and b if ∣∣¯a+¯b∣∣<∣∣¯a−¯b∣∣ |then ¯a and ¯b are
View SolutionThe vectors ¯a,¯b&¯c each two of which are non collinear. If ¯a+¯b is collinear with ¯c,¯b+¯c is collinear with ¯a&|¯a|=∣∣¯b∣∣=|¯c|=√2 .Then the value of ∣∣¯a.¯b+¯b.¯c+¯c.¯a∣∣=
View SolutionFor any three non-zero vectors ¯a,¯b,¯c,∣∣¯aׯb.¯c∣∣=|¯a|∣∣¯b∣∣|¯c| hold if and only if
View SolutionGiven four non zero vectors ¯a,¯b,¯cand¯d. The vectors ¯a,¯band¯c are coplanar but not collinear pair by pairand vector ¯d is not coplanar with vectors ¯a,¯band¯candˆ¯a¯b=ˆ¯b¯c=π3,(¯d¯b)=β ,If (¯d¯c)=cos−1(mcosβ+ncosα) then m−n is :
View SolutionIf ¯a,¯b,¯c are non coplanar vectors and λ is a real number then [λ(¯a+¯b)λ2¯bλ¯c]=[¯a¯b+¯c¯b] for
View Solution