If ¯a and ¯b any two non-collinear vectors lying in the same plane, then prove that any vector ¯r coplanar with them can be uniquely expressed as ¯r=t1¯a+t2¯b, where t1andt2 are scalars.
Take any points O in the plane of ¯a,¯band¯r. Represents the vectors ¯a,¯band¯r by ¯¯¯¯¯¯OA,¯¯¯¯¯¯OBand¯¯¯¯¯¯OR. Take the points P on ¯a and Q ¯b such that OPRQ is a parallelogram.
Now ¯¯¯¯¯¯OPand¯¯¯¯¯¯OA are collinear vectors.
∴ there exists a non - zero scalar t1 such that
¯¯¯¯¯¯OP=ta⋅¯¯¯¯¯¯OA=t1⋅¯a.
Also ¯¯¯¯¯¯OQand¯¯¯¯¯¯OB are collinear vectors.
∴ there exixts a non-zero scalar t2 such that ¯¯¯¯¯¯OQ=t2⋅¯¯¯¯¯¯OB=t2⋅¯b.
Now, by parallelogram law of addition of vectors,
¯¯¯¯¯¯OR=¯¯¯¯¯¯OP+¯¯¯¯¯¯OQ ∴¯r=t1¯a+t2¯b
Thus ¯r expressed as linear combination t1¯a+t2¯b
Uniqueness:
Let, if possible, ¯r=t′1¯a+t′2¯b, where t′1,t′2 are non-zero scalars. Then
t1¯a+t2¯b=t′1¯a+t′2¯b
∴(t1−t′1)¯a=−(t2−t′2)¯b . . . . (1)
WE want to show that t1=t′1andt2=t′2.
Suppose t1≠t′1,i.e.,t1−t′1≠0andt2≠t′2≠0.
Then dividing both sides of (1) by t1−t′1, we get,
¯a=−(t2−t′2t1−t′1)¯b
This shows that the vector ¯a is a non-zero scalar multiple of ¯b.
∴¯aand¯b are collinear vectors.
This is a contradiction, since ¯a,¯b are given to be non-collinear.
∴t1=t′1
Similarly, we can show tath t2=t′2
This shows that ¯r is uniquwly expressed as a linear combination t1¯a+t2¯b.

Topper's Solved these Questions
- VECTORSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - VECTORSEXERCISE - Examples for Practics
28 Videos
- VECTORSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - VECTORSEXERCISE - Multiple choice question
10 Videos
- VECTORSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - VECTORSEXERCISE - Multiple choice question
10 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Multiple Choice Questions
12 Videos
Similar Questions
Knowledge Check
A vector coplanar with the non-collinear vectors ¯aand¯b is
A¯aׯbB¯a+¯bC¯a.¯bD¯a×3¯b
¯a & ¯b are two non- collinear vectors then the value of ⎧⎨⎩¯a|¯a|2−¯b∣∣¯b∣∣2⎫⎬⎭2 is equal to
View Solution¯a and ¯b are non- collinear vectors. If ¯c=(x−2)¯a+¯b and ¯d=(2x+1)¯a−¯b are collinear , then find the value of x
View SolutionIf ¯¯¯Aand ¯¯¯Bare two vectors then A new vector parallel to ¯¯¯Ahaving the magnitude of ¯¯¯Bis
View Solution¯a and ¯b are non-collinear vectors. If ¯c=(x−2)¯a+¯b and ¯d=(2x+1)¯a−¯b are collinear, then find the value of x.
View Solution¯a,¯b,¯c are three coplanar vectors, If ¯a is not parallel to ¯b, Show that
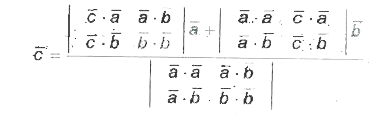
View SolutionIf ¯aand¯b are unit vectors then the vectors (¯a+¯b)×(¯aׯb) is parallel to the vector
View SolutionIf ¯a,¯b,¯c are non-coplanar unit vectors each including the angle of measure 30∘ with the other, then find the volume of tetrahedron whose co-terminal edges are ¯a,¯b,¯c.
View Solution
