The area bounded in the first quadrant by the normal at(1,2)on the curve ,y2=4x,x−axis &the curve is given by (A) 103 (B) 73 (C) 43 (D) 92
A
10/3
B
7/3
C
4/3
D
None of these
Video Solution
Text Solution
The correct Answer is:A
Equation of normal at (1, 2 ) is x+y=3, which meets X -axis at N(3,0)
∴QN=2,PQ=2
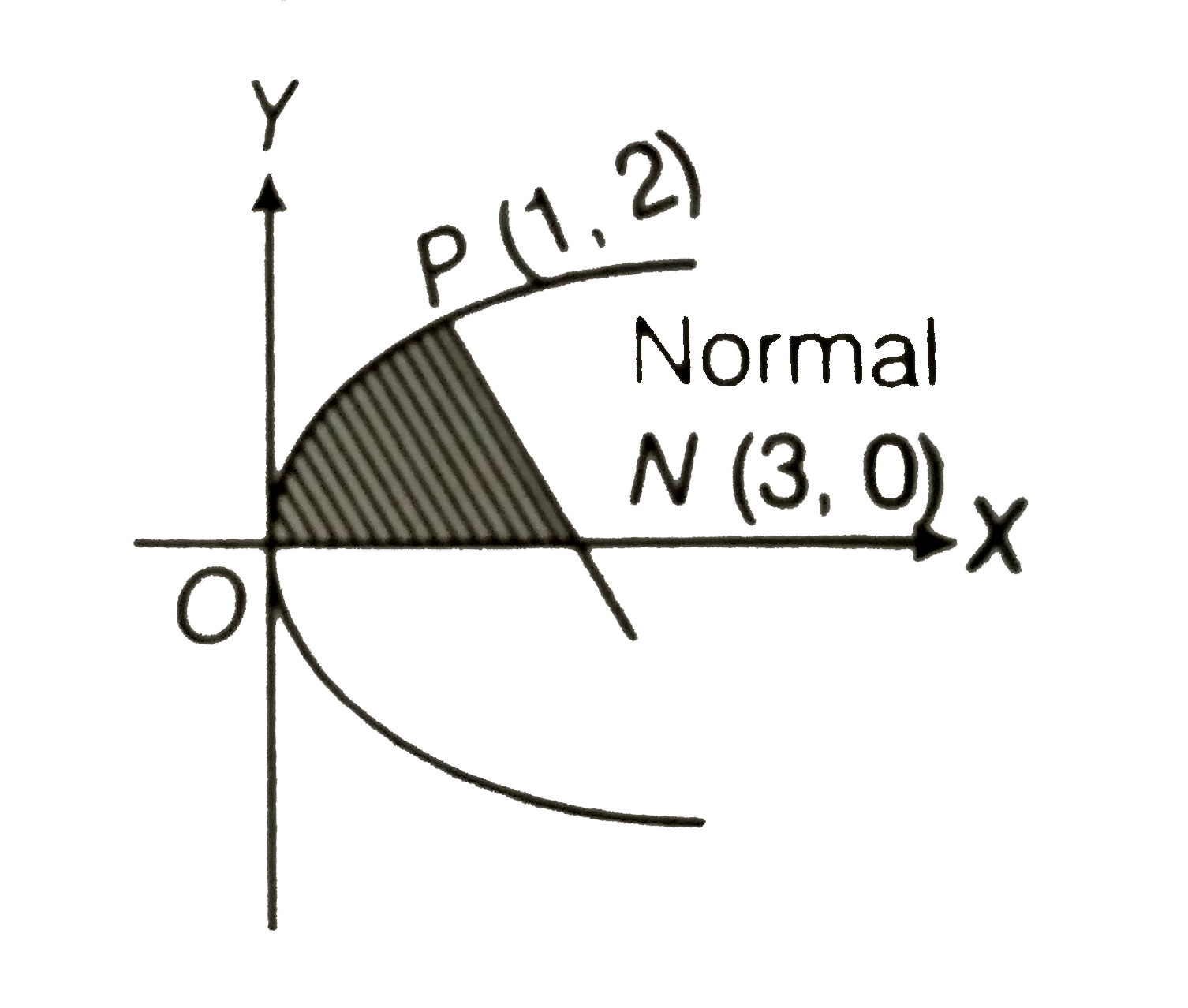
∴ Required area
= Area of ΔPQO + Area of ΔPQN
=∫10√(4x)dx+12⋅2⋅2=103
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
Find the area lying in the first quadrant and bounded by the curve y=x3 and the line y=4x.
A2B3C4D5What is the area bounded by the curve y=4x−x2−3 and the x-axis?
A4/3B3/4C7D3/2The area lying in the first quadrant and bounded by the circle x2+y2=4 and the line x=√3y is :
Aπ3Bπ2CπDπ5
Find the area lying in the first quadrant and bounded by the curve y=x3 and the line y=4x.
View SolutionThe area bounded by the x-axis and the curve y=4x−x2−3 is
View SolutionFind the area lying in the first quadrant, bounded by the curves y2−x+2=0 and y=|x−2|.
View SolutionFind the area of the region lying in the first quadrant bounded by the curve y2=4x, X axis and the lines x=1,x=4.
View SolutionThe area bounded by the curves y2=x3and|y|=2x is
View Solution
