The electrical analog of a spring constant k is
L
R
C
1C
The correct Answer is:D
Step by step video, text & image solution for The electrical analog of a spring constant k is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - AAKASH INSTITUTECHAPTER - ALTERNATING CURRENT EXERCISE - Assignment (Section - B) (Objective Type Questions (One option is correct))12videos
ALTERNATING CURRENT
- BOOK - AAKASH INSTITUTECHAPTER - ALTERNATING CURRENT EXERCISE - Assignment (Section - C ) (Objective Type Questions) ( More than one option are correct)2videos
ALTERNATING CURRENT
- BOOK - AAKASH INSTITUTECHAPTER - ALTERNATING CURRENT EXERCISE - Try Yourself15videos
ALTERNATING CURRENT
- BOOK - AAKASH INSTITUTECHAPTER - ATOMSEXERCISE - ASSIGNMENT SECTION J (Aakash Challengers )4videos
ATOMS
Similar Questions
What is the mechanical equivalent of spring constant k in LC oscillating circuit?
View SolutionWhat is the mechanical equivalent of spring constant K, in I.C oscillating circuit ?
View SolutionThe frequency f of vibrations of a mass m suspended from a spring of spring constant k is given by f=Cmxky , where C is a dimensionnless constant. The values of xandy are, respectively,
View SolutionOne-forth length of a spring of force constant K is cut away. The force constant of the remaining spring will be
View SolutionA light spring of spring constant k is cut into two equal halves. Each half is connected in parallel then net spring constant of the combination is :
View SolutionA spring of certain length and having spring constant k is cut into two pieces of length in a ratio 1:2. The spring constants of the two pieces are in a ratio :
View SolutionA solid sphere rolls without slipping and presses a spring of spring constant k as shown in figure. Then, the compression in the spring will be
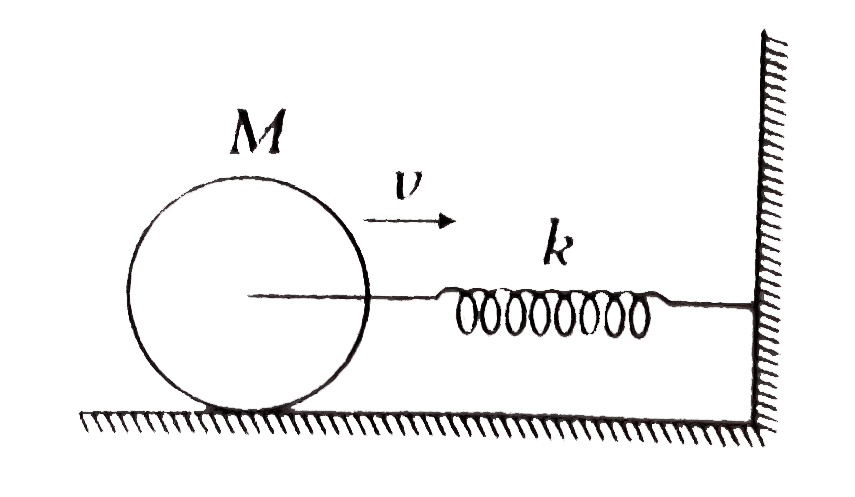 View Solution
View SolutionA spring of length 'l' has spring constant 'k' is cut into two parts of length l1 and l2. If their respective spring constahnt are K1 and k2, then K1K2 is:
View Solution
The period of oscillation of a mass M suspended from a spring of spring constant K is T. the time period of oscillation of combined blocks isView SolutionA massless spring of length l and spring constant k is placed vertically on a table. A ball of mass m is just kept on top of the spring. The maximum velocity of the ball is
View Solution


