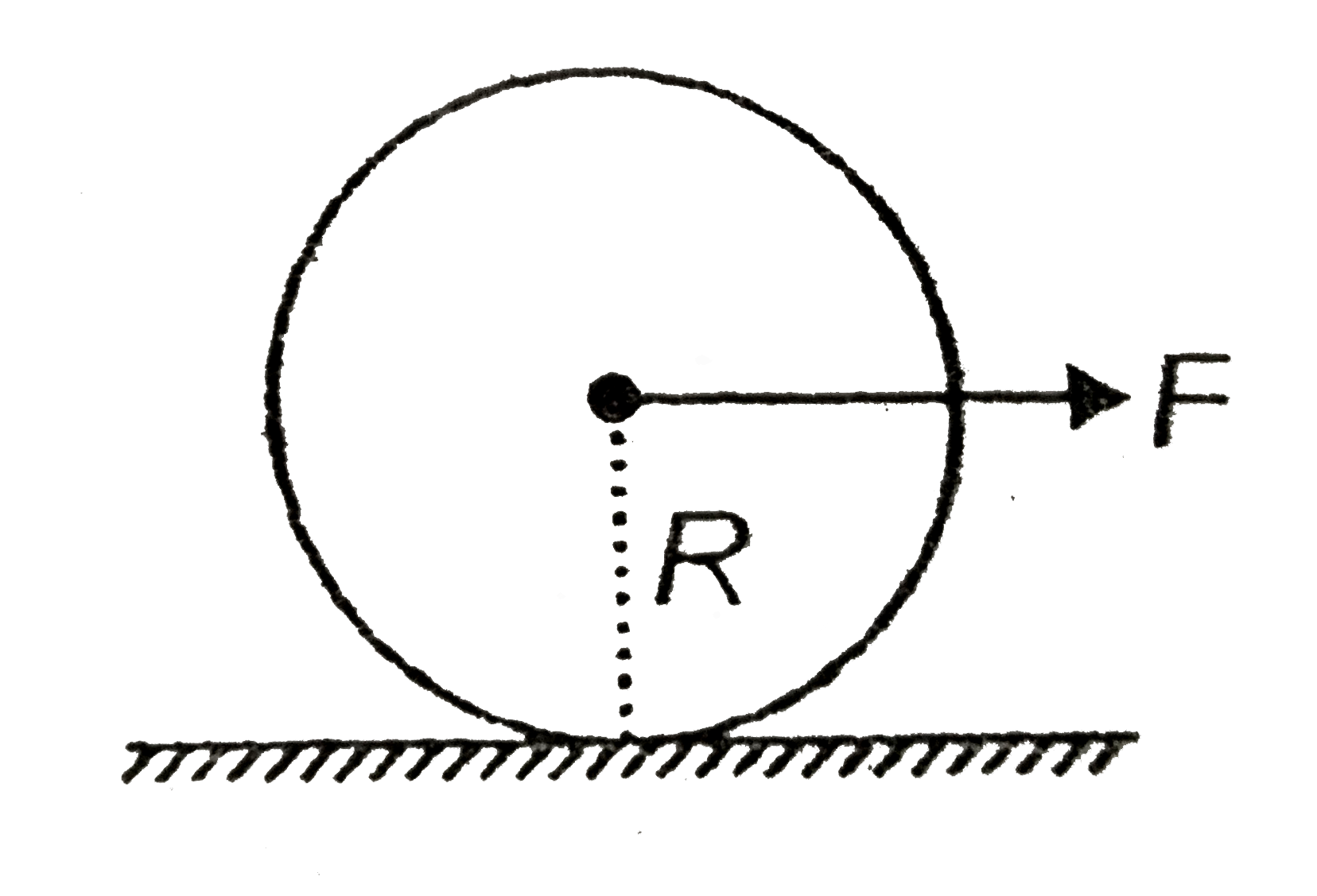
A force $F$ is applied on a disc at it's centre. Find acceleration of center of mass in the case of pure rolling and also find minimum coefficient of fricition required for pure rolling.

Video Solution

Text Solution

Verified by Experts

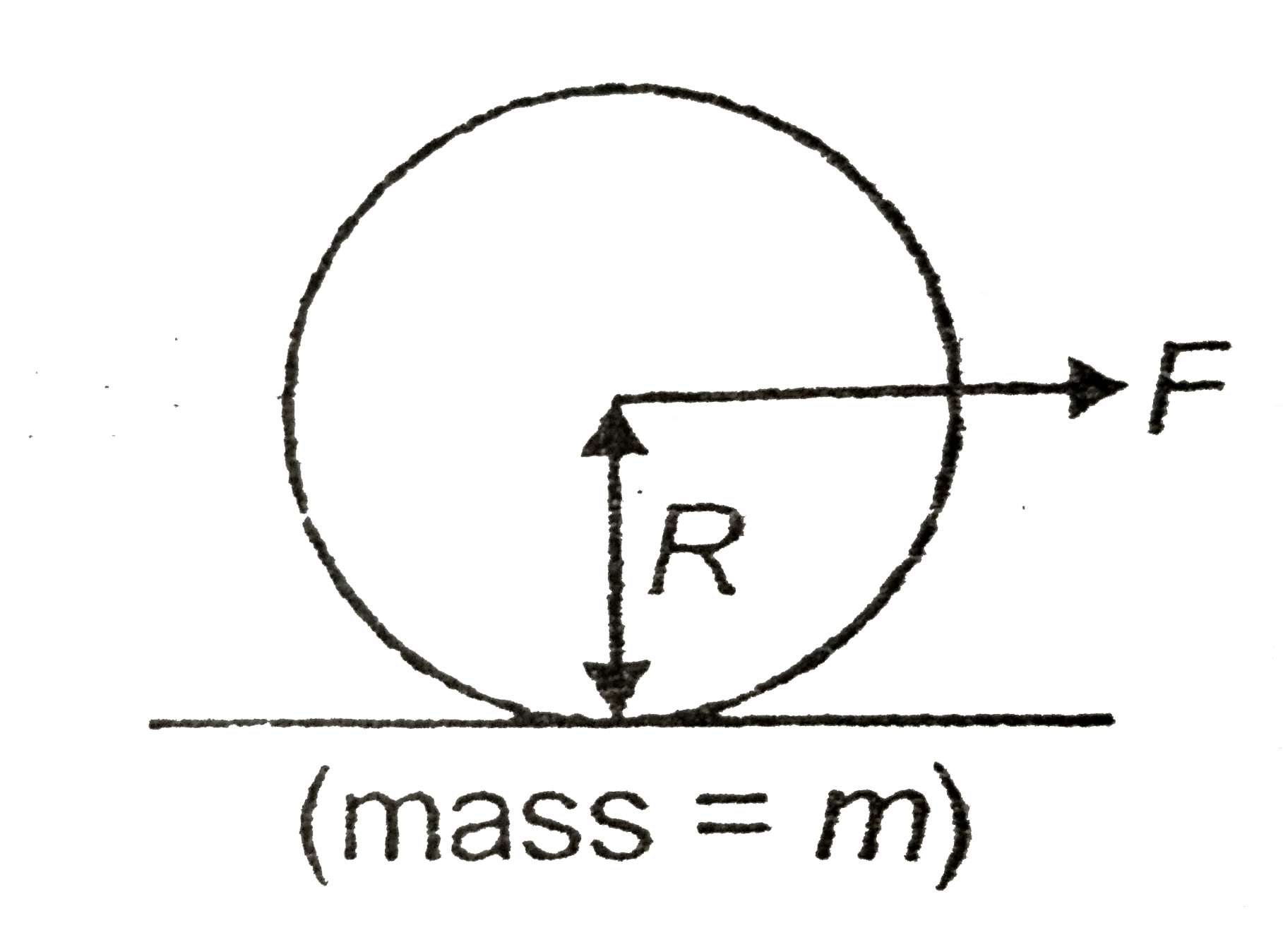
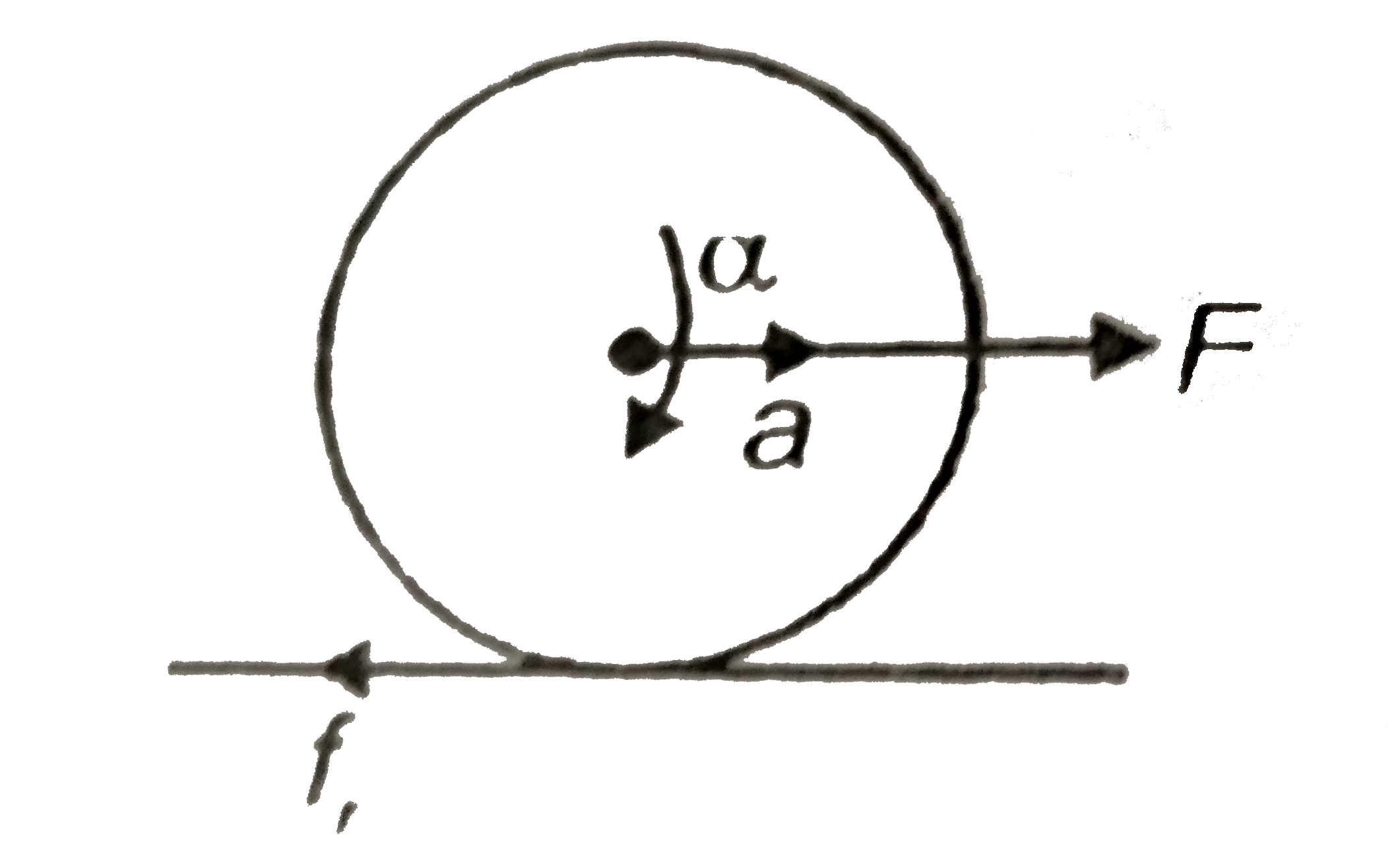
Let the friction force acting on disc be $f_{r}$ , acceleration of centre of mass is a and angular acceleration is $α$ .
We have three unknowns
$(i)$ a
$(i i) α$
$(i i i) f_{r}$
We require $3$ equations to solve them
Translatory Motion
$F - f_{r} = m a$ ...... $(i)$
Rotatory Motion
$r \cdot f_{r} = \frac{m r^{2}}{2} \cdot α$ ........ $(i i)$
Condition of no slipping
$a = r α$ .......... $(i i i)$
By solving $(i i)$ & $(i i i)$
$f_{r} = \frac{m a}{2}$ , $F = \frac{3 m a}{2} \Rightarrow a = \frac{2 F}{3 m}$ , $f_{r} = \frac{m}{2} \times \frac{2 F}{3 m}$ , $f_{r} = \frac{F}{3}$
Now, $f_{r} \leq μ N$
$\Rightarrow \frac{F}{3} \leq μ m g$
$\Rightarrow μ \geq \frac{F}{3 m g}$

|

Updated on:21/07/2023

Class 12 PHYSICS SYSTEM OF PARTICLES AND ROTATIONAL MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A force F is applied horizontally on a cylinder in the line of centre as shown in the figure. The cylinder is on a rough surface of coefficient of fricition $μ$ . The direction of the friction force acting on the cylinder will be

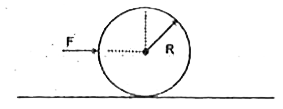Ain the direction of F
Bzero
Cin opposite direction of F
Dcan not be ascertained
Question 2 - Select One

A horizontal force $F$ is applied at the centre of solid sphere placed over a plank. The minimum coefficient of friction between plank and sphere required for pure rolling is $μ_{1}$ when plank is kept at rest ad $μ_{2}$ when plank can move, then $μ_{2} < μ_{1}$
Reason: Work done by frictional force on the sphere in both cases is zero.
AIf both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
BIf both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
CIf assertion is true, but the reaction is false.
DIf assertion is false but the reason is true.
Question 3 - Select One
A force F is applied at the centre of a disc of mass M. The minimum value of coefficient of friction of the surface for rolling is
A $\frac{F}{2 M g}$
B $\frac{F}{3 M g}$
C $\frac{2 F}{5 M g}$
D $\frac{2 F}{7 M g}$

AAKASH INSTITUTE-SYSTEM OF PARTICLES AND ROTATIONAL MOTION-Try Yourself

Home
Ask Doubt
Profile