A particle of mass $m$ is projected with a speed $v$ at an angle $θ$ with the horizontal. Find the angular momentum of the particle about an axis passing through point of projection and perpendicular to the plane of motion of the particle.
$(a)$ When the particle is at maximum height and
$(b)$ When the particle is just about to collide with the horizontal surface

Video Solution

Text Solution

Verified by Experts

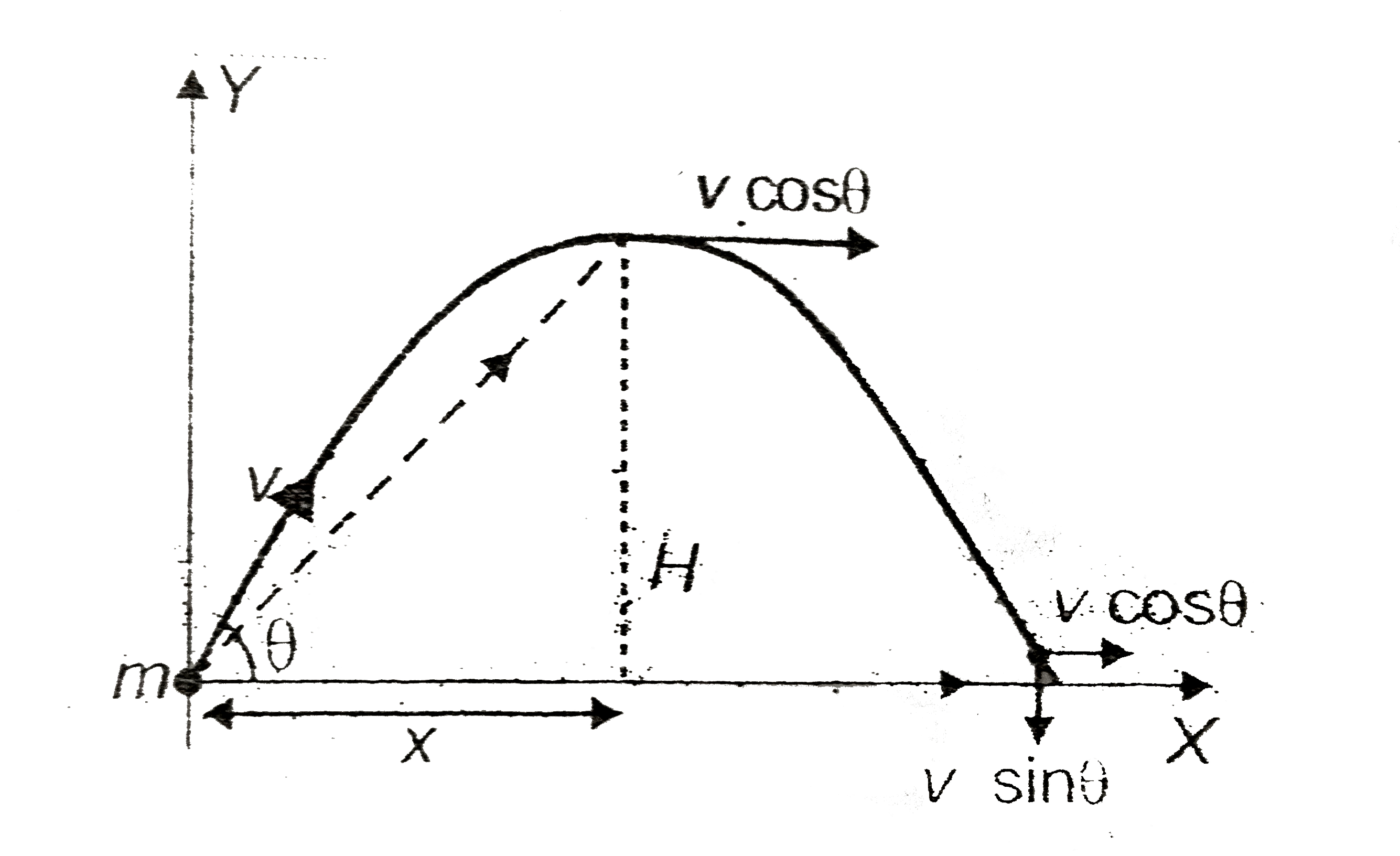
$(a)$ Position vector of the particle when it is at maximum height $H$ is
$\to r = x ˆ i + H ˆ j$
Linear momentum of the particle at maximum height
$\to p = m v cos θ ˆ i$
therefore $\to L = \to r \times \to p$
$= (x ˆ i + H ˆ j) \times m v cos θ ˆ i$
$= m v H cos θ (- ˆ k)$
$= m v cos θ \frac{v^{2} {sin}^{2} θ}{2 g} (- ˆ k)$
$= \frac{m v^{3} {sin}^{2} θ cos θ}{2 g} (- ˆ k)$
$(b)$ Position vector of the particle just before hitting ground s
$\to r = R ˆ i$
$= \frac{v^{2} sin 2 θ}{g} ˆ i$
Linear momentum of the particle is
$p = m v cos θ ˆ i - m v sin θ ˆ j$
Therefore $\to L = \to r \times \to p$
$= R ˆ i \times (m v cos θ ˆ i - m v sin θ ˆ j)$
$= m v R sin θ (- ˆ k)$
$= \frac{m v sin θ v^{2} sin 2 θ}{g} (- ˆ k)$
$\Rightarrow \to L = \frac{m v^{3} sin 2 θ sin θ}{g} (- ˆ k)$

Updated on:21/07/2023

A particle of mass m is projected with a velocity $μ$ at an angle of $θ$ with horizontal.The angular momentum of the particle about the highest point of its trajectory is equal to :
A $\frac{{mu}^{3} {sin}^{2} θ cos θ}{3 g}$
B $\frac{3 {mu}^{3} {sin}^{2} θ cos θ}{3 g}$
C $\frac{{mu}^{3} {sin}^{2} θ cos θ}{2 g}$
D $\frac{2 {mu}^{3} sin θ {cos}^{2} θ}{3 g}$
A projectile is projected with a velocity $v_{0}$ at an angle $θ$ with the horizontal as shown in figure. The angular momentum of particle about the origin:

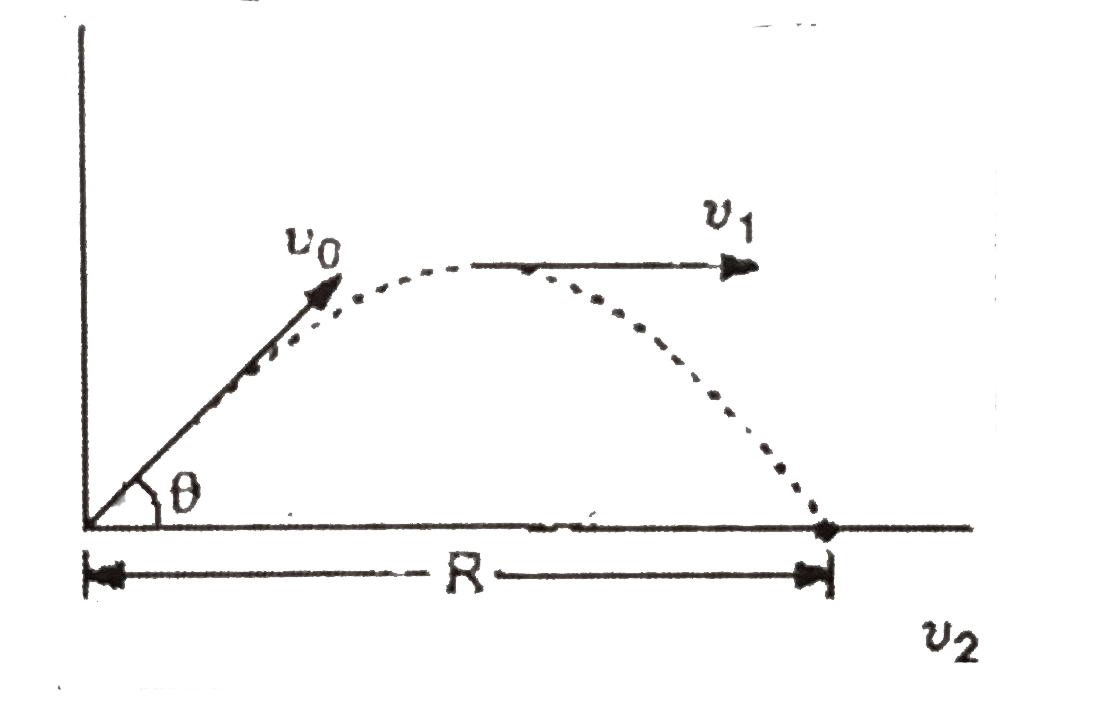Ais zero when particle is at the origin
Bis $\frac{- m v^{3} {sin}^{2} θ cos θ}{2 g} ˆ k$ when particle is at the highest point of trajectory
Cis $\frac{- 2 m v^{2} {sin}^{2} θ cos θ}{g} ˆ k$ when particle is just about to hit ground
Ddownward force of gravity exerts a torque in $- z$ direction.
A particle of mass m is projected with an initial velocity U at an angle $θ$ to the horizontal. The torque of gravity on projectile at maximum height about the point of projection is
A $\frac{m U^{2} Sin 2 θ}{2}$
B $m U^{2} Sin 2 θ$
C $\frac{m U^{2} Sin θ}{2}$
D $\frac{1}{2} m U^{2} {(Sin 2 θ)}^{2}$