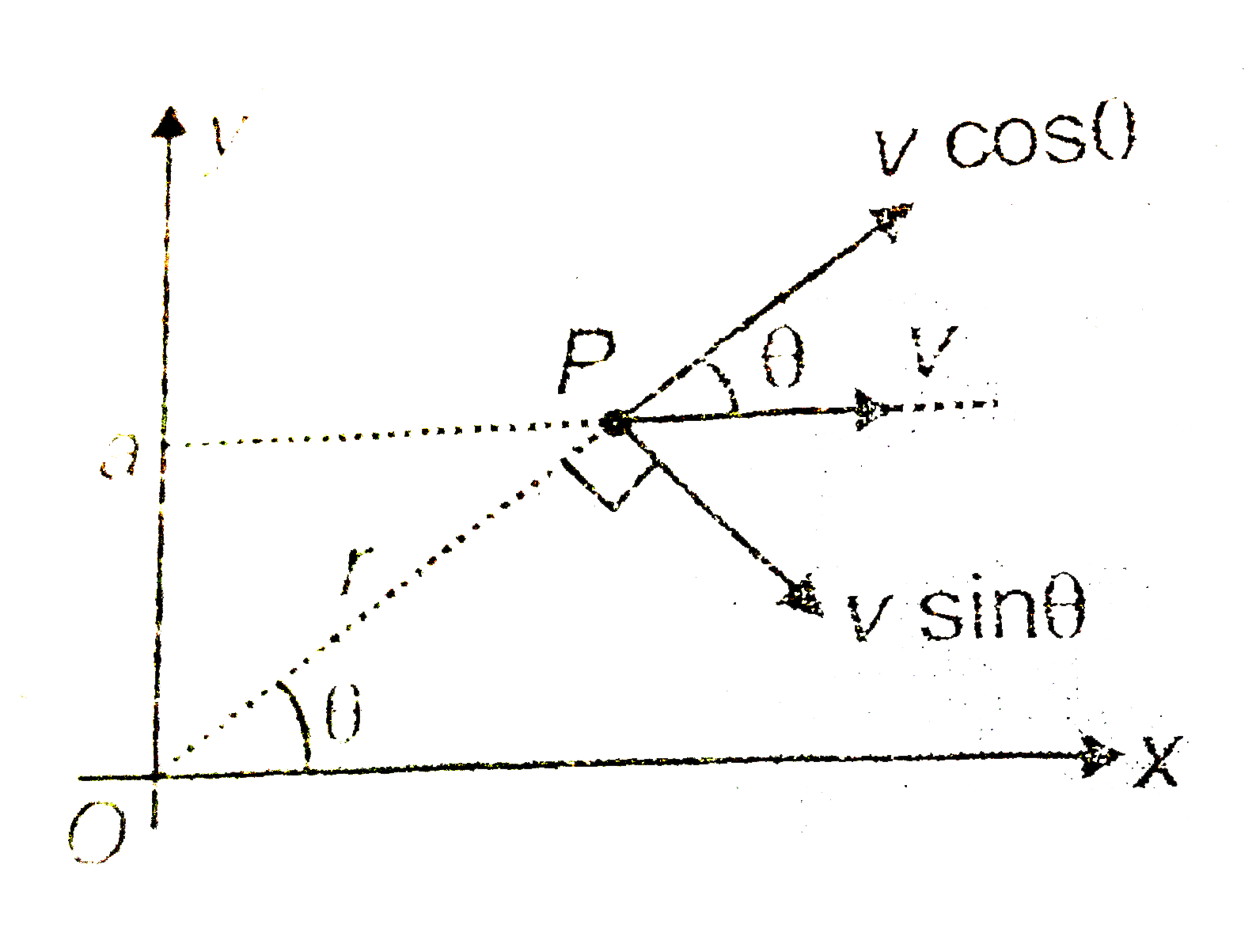
A particle is moving with constant speed v along the line y = a in positive x -direction. Find magnitude of its angular velocity about orgine when its position makes an angle θ with x-axis.
Consider the particle is at point P as shown in the figure. We will resolve its velocity into two components vcosθ, which is radial and outward from point O and vsinθ which is perpendicular component.
Now ω=v⊥r=vsinθr
where ar=sinθ
⇒r=asinθ
∴ω=vsin2θa
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBook:AAKASH INSTITUTEChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTIONExercise:Illustration
Explore 4 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBook:AAKASH INSTITUTEChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTIONExercise:Assignment (Section - A) Objective Type Questions (One option is correct)
Explore 62 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBook:AAKASH INSTITUTEChapter:SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSExercise:Assignment (Section-D (Assertion and reason))
Explore 5 Videos
- TEST 1Book:AAKASH INSTITUTEChapter:TEST 1Exercise:EXERCISE
Explore 9 Videos
Similar Questions
A particle is moving with constant speed v along x - axis in positive direction. Find the angular velocity of the particle about the point (0, b), when position of the particle is (a, 0).
View SolutionA particle is moving with a constant speed in a circular path. Find the ratio of average velocity to its instantaneous velocity when the particle rotates an angle θ=(π2) .
View Solution
Knowledge Check
A particle of mass m is moving with constant speed v on the line y=b in positive x-direction. Find its angular momentum about origin, when position coordinates of the particle are (a, b).
AmvbBmvb/2Cmvb/4DnoneA particle is moving with constant speed v in xy plane as shown in figure. The magnitude of its angular velocity about point O is
 Av√a2+b2BvbCvb(a2+b2)Dva
Av√a2+b2BvbCvb(a2+b2)DvaA particle of mass m is moving with constant velocity v parallel to the x-axis as shown in the figure. Its angular momentum about origin O is
 AmvbBmvaCmv√a2+b2Dmv(a+b)
AmvbBmvaCmv√a2+b2Dmv(a+b)
A particle of m=2kg is moving with constant speed 15m/s along a curved path y=x^(2) . Find its angular momentum about origin 0 when its velocity vector is at 53^(@) with x -axis (in kgm^(2)/s)
View SolutionA particle is going parallel to x - axis with constant speed V at a distance 'a' from the axis . Find its angular velocity about an axis passing the origin O , at the instant when radial vector of the particle makes angle θ with the x - axis
View SolutionA particle is moving along a circle with a uniform speed v. Find (a) change in the magnitude of velocity and (b) the magnitude of change in the velocity when it has rotated an angle θ.(0<θ<90∘)
View SolutionA particle is moving in the xy-plane with a constant velocity along a line parallel to the X-axis away from the origin. The magnitude of its angular momentum about the origin.
View SolutionA particle of mass m is moving on the XY-plane along a straight line y = d, with constant velocity v. Its angular momentum about the origin
View Solution