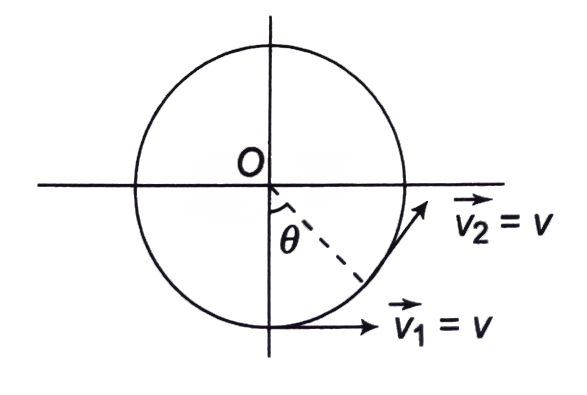
A particle is moving along a circle with a uniform speed v. Find (a) change in the magnitude of velocity and (b) the magnitude of change in the velocity when it has rotated an angle θ.(0<θ<90∘)
(a) Since the particle is moving with a uniform speed , the magnitude of velocity |→v|=v , remains same , hence the change in the magnitude of velocity , i.e. Δ|→v|=0.
(b) The magnitude of change in the velocity |Δ→v|:
OR
We can represent
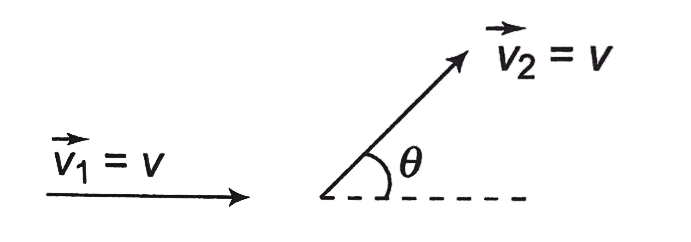
Δv=→v2−→v1=→v2+(−→v1)
Δv=√v2+v2+2v.vcos(180−θ)
=√2v2−2v2cosθ [sincecos(180−θ)=−cosθ]
=√2v√1−cosθ
=√2v
⎷(1−(1−2sin2(θ)2)
=2vsin(θ2)
Change in the velocity =|Δ→v|=Δv=2vsin(θ2).
If θ=90∘,Δv=√2v (as in the previous problem).

Topper's Solved these Questions
Similar Questions
A body is moving uniformly on a circle with speed v. Find the magnitude of change in its velcotiy whem, it has turend an angle θ.
View SolutionA particle is moving along a circular path with a constant speed 10ms−1.What is the magnitude of the change in velocity of the particle ,when it moves through an angle of 60∘ around the center of the circle ?
View Solution
Knowledge Check
A particle is moving with a constant speed v in a circle. What is the magnitude of average velocity after half rotation?
A2vBv(vπ)Cv2Dv2πA motor car is moving in a circular path with uniform speed v. Suddenly the car rotates through an angle θ. Then, the magnitude of change in its velocity is
A2vcos(θ2)B2vsin(θ2)C2vtan(θ2)D2vsec(θ2)A particle is moving along a circular path with uniform speed. Through what angle does its angular velocity change when it completes half of the circular path ?
A0∘B45∘C180∘D360∘
A particle is moving along a circular path with uniform speed. Through what angle does its angular velocity change when it completes half of the circular path ?
View SolutionA paricle is moving along a circular path with uniform speed. Through what angle does its angular velocity change when it completes half of the circular path?
View SolutionA particle is moving on a circular path with constant speed v then the change in its velocity after it has desceibed an angle of 60∘ will be
View SolutionA particle is moving on a circular path with a constant speed 'v'. Its change of velocity as it moves from A to B is:

View SolutionWhen a particle is moving along a circular path with uniforme speed , the physical quantity which remains constant both in magnitude and direction is
View Solution