A uniform chain of mass 4 kg and legth 2 m overhags a smooth table with its one third part lying on the table Find the speed of chain as it c ompletely slips of the table. (Take )
The correct Answer is:A
To solve the problem of finding the speed of the chain as it completely slips off the table, we can use the principles of energy conservation. Here is the step-by-step solution:
Step 1: Understand the setup
The chain has a total length of 2 m and a mass of 4 kg. One-third of the chain (which is 23 m) is hanging off the table, while the remaining one-third (which is 23 m) is on the table.
Step 2: Calculate the mass of the hanging part
Since the chain is uniform, the mass per unit length is given by:
Mass per unit length=Total massTotal length=4kg2m=2kg/m
The length of the hanging part is 23 m, so the mass of the hanging part is:
Mass of hanging part=Mass per unit length×Length of hanging part=2kg/m×23m=43kg
Step 3: Calculate the potential energy of the hanging part
The center of mass of the hanging part is at a distance of 13 m from the table edge (since it is hanging down). The potential energy (PE) of the hanging part when it is at rest is given by:
PE=mgh=(43kg)×(10m/s2)×(13m)=409J
Step 4: Apply conservation of energy
When the chain slips off the table, the potential energy will convert into kinetic energy (KE). The total mass of the chain is 4 kg, and the kinetic energy when the chain has completely slipped off the table is given by:
KE=12mv2
Setting the potential energy equal to the kinetic energy gives:
409J=12×4kg×v2
Step 5: Solve for the speed v
Rearranging the equation to solve for v2:
409=2v2⟹v2=4018=209
Taking the square root gives:
v=√209=√203=2√53m/s
Final Answer
The speed of the chain as it completely slips off the table is:
v≈1.49m/s
---
The chain has a total length of 2 m and a mass of 4 kg. One-third of the chain (which is 23 m) is hanging off the table, while the remaining one-third (which is 23 m) is on the table.
Since the chain is uniform, the mass per unit length is given by:
Mass per unit length=Total massTotal length=4kg2m=2kg/m
The length of the hanging part is 23 m, so the mass of the hanging part is:
Mass of hanging part=Mass per unit length×Length of hanging part=2kg/m×23m=43kg
The center of mass of the hanging part is at a distance of 13 m from the table edge (since it is hanging down). The potential energy (PE) of the hanging part when it is at rest is given by:
PE=mgh=(43kg)×(10m/s2)×(13m)=409J
When the chain slips off the table, the potential energy will convert into kinetic energy (KE). The total mass of the chain is 4 kg, and the kinetic energy when the chain has completely slipped off the table is given by:
KE=12mv2
Setting the potential energy equal to the kinetic energy gives:
409J=12×4kg×v2
Rearranging the equation to solve for v2:
409=2v2⟹v2=4018=209
Taking the square root gives:
v=√209=√203=2√53m/s
The speed of the chain as it completely slips off the table is:
v≈1.49m/s
Topper's Solved these Questions
- WORK, ENERGY AND POWERBook:AAKASH INSTITUTEChapter:WORK, ENERGY AND POWERExercise:SECTION-C (OBJECTIVE TYPE QUESTIONS) (MORE THAN ONE OPTIONS ARE CORRECT)
Explore 16 Videos
- WORK, ENERGY AND POWERBook:AAKASH INSTITUTEChapter:WORK, ENERGY AND POWERExercise:SECTION-D (LINKED COMPREHENSION TYPE QUESTIONS) (COMPREHENSION-I)
Explore 3 Videos
- WORK, ENERGY AND POWERBook:AAKASH INSTITUTEChapter:WORK, ENERGY AND POWERExercise:SECTION-A (OBJECTIVE TYPE QUESTIONS (ONE OPTIONISCORRECT)
Explore 60 Videos
- WAVESBook:AAKASH INSTITUTEChapter:WAVESExercise:ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
Explore 12 Videos
Similar Questions
A uniform chain of length l and mass m overhangs a smooth table with its tow third pat lying on the tabl. Find the kinetic energy of the chain s it completely slips off the table.
View SolutionA uniform chain of mass m and length l overhangs a table with its two third part on the table. Fine the work to be done by a person to put the hanging part back on the table.
View Solution
Knowledge Check
A chain of mass m and length l is placed on a table with one-sixth of it hanging freely from the table edge. The amount of work. done to pull the chain on the table is
Amgl/4Bmgl/6Cmgl/72Dmgl/36A chain of mass m and length l is placed on a table with one-sixth of it hanging freely from the table edge. The amount of work. done to pull the chain on the table is
Amgl/4Bmgl/6Cmgl/72Dmgl/36
A unifrom chain of mass M and length L lies on a table with nth part of it hanging off the table. Find the work required to slowly pull the hanging part up to the table.
View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

What is the speed of the chain, when it just loses contact with the table?
View SolutionA uniform chain of length L and mass M overhangs a horizontal table with its two third part on the table. The friction coefficient between the table and the chain is µ. The work done by the friction during the period the chain slips off the table is [−2kμMgL]. Find the value of k.
View SolutionA uniform chain of mass m and length l is placed on a smooth table so that one−third length hanges freely as shown in the figure. Now the chain is released, with what velocity chain slips off the table?
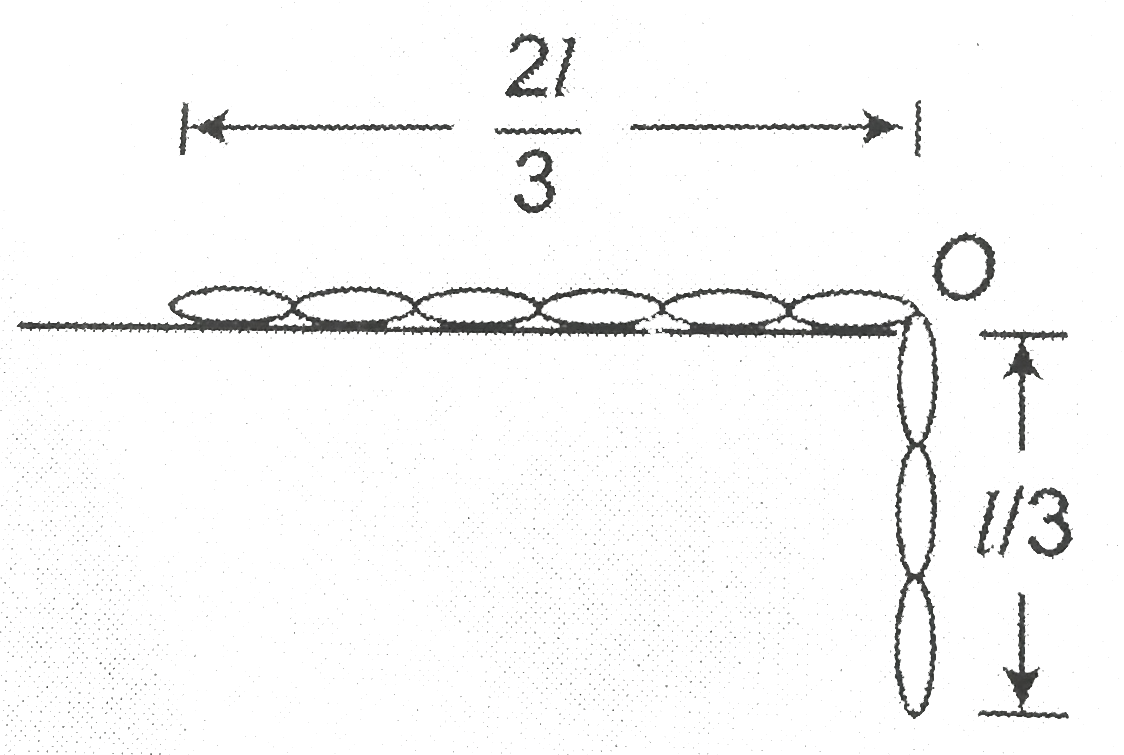
View SolutionA uniform chain of mass m and length l is kept on a horizontal table with half of its length hanging from the edge of the table.Work done in pulling the chain on to the table so that only 1/5 th of its length now hangs from the edge is K(mgl200) .Find the value of K.
View SolutionA chain of mass m and length l lies on a horizontal table. The chain is allowed to slide down gently from the side of the table. Find the speed of the chain at the instant when last link of the chain slides from the table. Neglect friction everywhere.
(a) the force from thelicopter and
(b) the gravitational force on her
(c ) What are speed ot the kinetic energy and.
(d) the speed of the astronaut just before she reaches the helicopter?
View Solution
