Figure shows two discs of same mass m. They are rigidy attached to a spring of stiffness k. The system is in equlibrium. From this equilibrium position, the upper disc is pressed down slowly by a distance x and released. Find the minimum vlue of x, if the lower disc is just lifted off the ground.
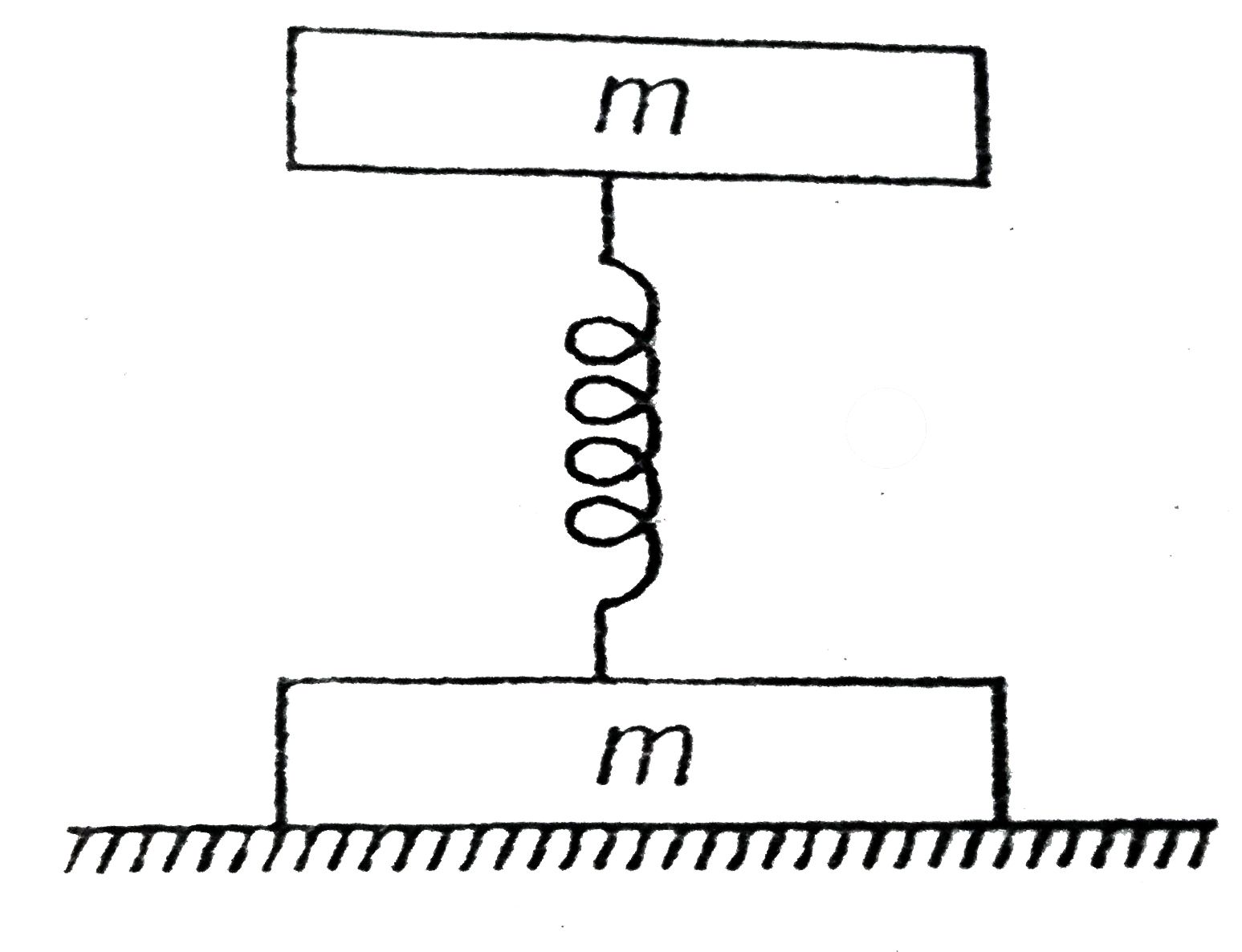
Let us first find out the minimum elongation (x') needed to lift the lower discc from the ground . THe free body digram of the lower disct at this instant will be
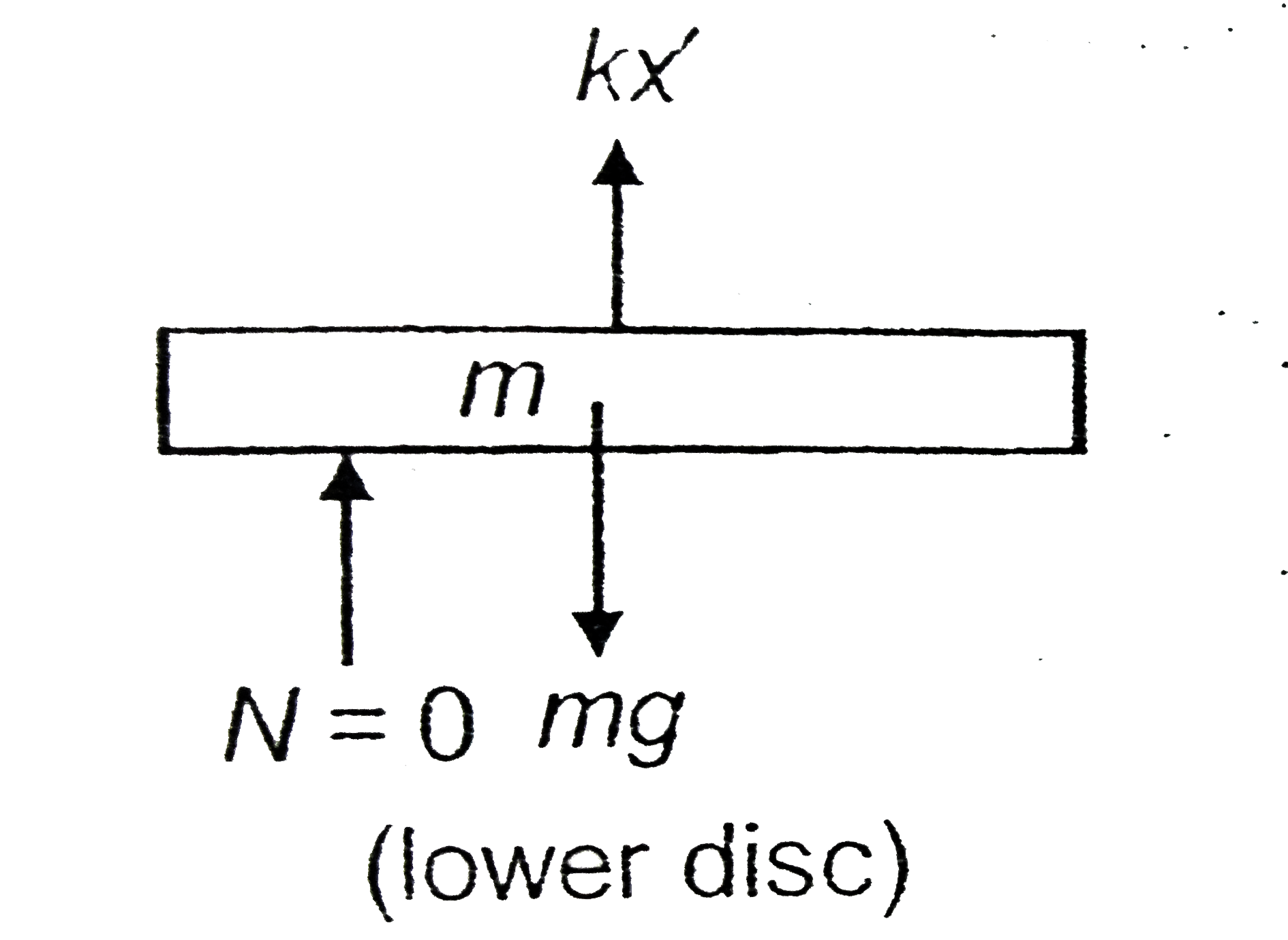
The system given in the question is in equlibrium thus for the equlibrium of upper disc initial compression (x0) in spring is
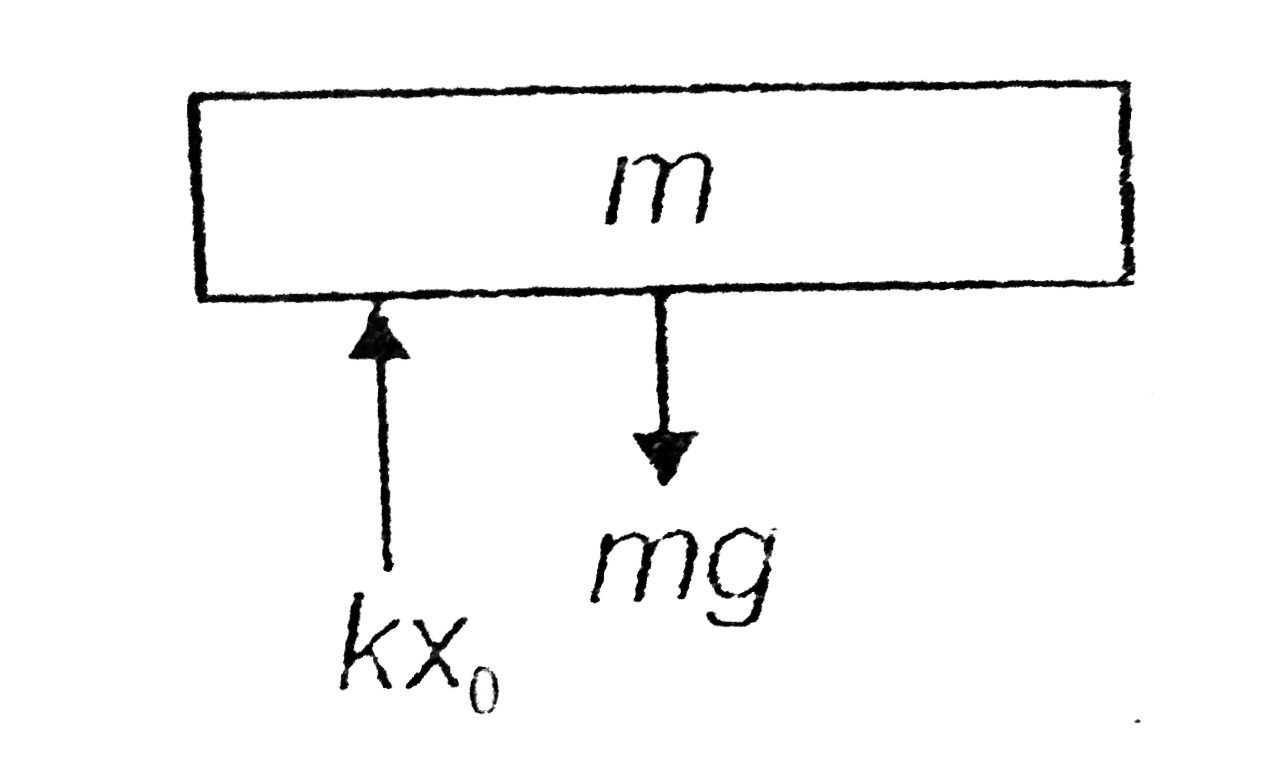
now from this equlibrium state spring is further compressed by an amount x and released. Now let us apply energy conservation from this compressed state and final state. Let us take the reference for gravitation potential energy at unstreatched position then
12k(x+x0)2=−mg(x+x0)=12kx2+mgx'
Now, x0=mgk
x=mgk
on solving, we get
x=2mgk
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - IILUSTRATION
2 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - TRY YOURSELF
95 Videos
- WAVESBOOK - AAKASH INSTITUTECHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
A sand bag of mass m is hanging from a light spring of stiffness k. Find the elongation of the spring. If we pull the sand bag down by an additional distance x and release it, find its acceleration and maximum velocity of block.
View SolutionIn the figure shown, a block A of mass m is rigidy attached to a light spring of stiffness k and suspended from a fixed support. Another block B of same mass is just placed on it and blocks are in equilibrium. Suddenly the block B is removed. Choose the correct options (s) afterward.
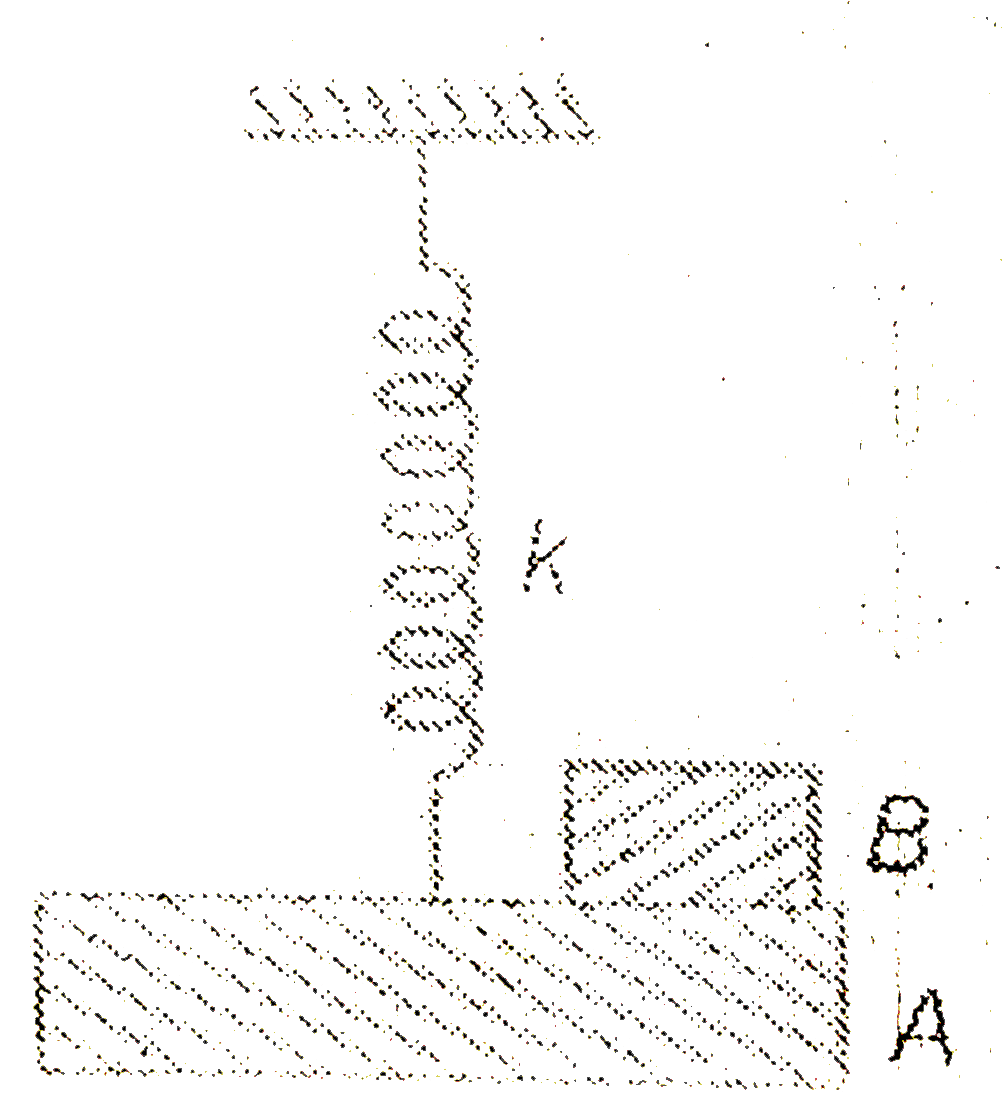
View Solution
Knowledge Check
A bob of mass 2m hanges by a string attached to the block of mass m of a spring blocks syetem. The whole arrangement is in a state of equilibrium. The bob of mass 2m is pulled down slowely by a distance x0 and released.
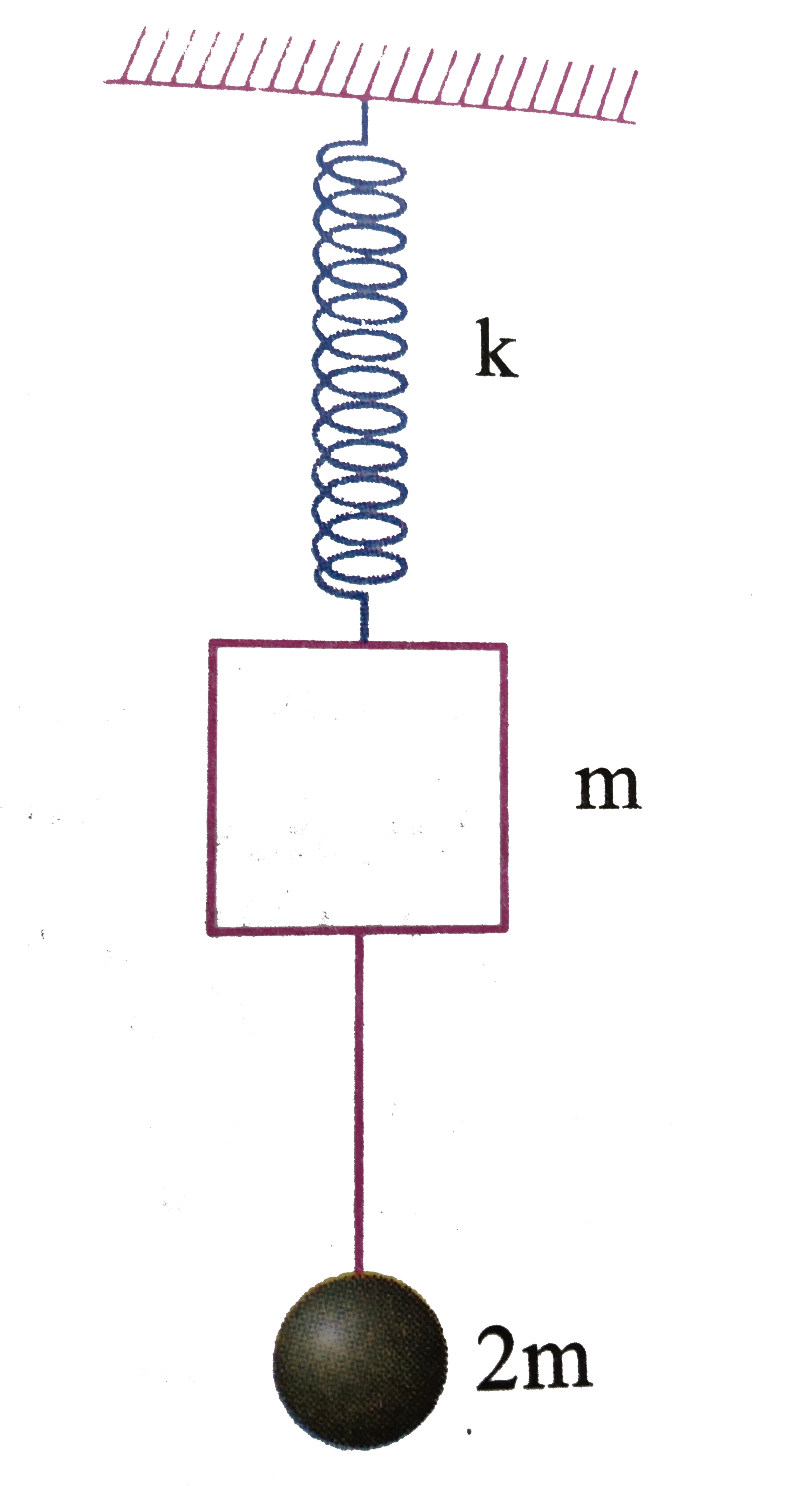 AFor x0=3mgk, maximum tension in string is 4mgBFor x0>3mgk, minimum tension in string is mgCFrequency of oscillation of system is 12π√k3m, for all non-zero values of x0DThe motion will remain simple harmonic for x0≥3mgk
AFor x0=3mgk, maximum tension in string is 4mgBFor x0>3mgk, minimum tension in string is mgCFrequency of oscillation of system is 12π√k3m, for all non-zero values of x0DThe motion will remain simple harmonic for x0≥3mgkA disc of mass 3 m and a dise of mass m are connected by a massless sping of stiffness k. The heavier is disc placed on the ground with the spring vertical and lighter disc on top from its equilibrium position the upper disc is pushed down by a distance δ and released. Then.
Aif δ>3mgk the lower desc will bounce up.Bif δ=2mgk maximum normal reaction fron on lower disc = 6mg .Cif δ=2mgk maximum normal reaction from ground on lower disc = 4 mg.Dif δ>4mgk, the lower disc will bounce upPassage VIII A disc of mass m and radius R is attached with a spring of force contant k at its center as shown in figure. At x-0, spring is unstretched. The disc is moved to x=A and then released. There is no slipping between disc and ground. Let f be the force of friction on the disc from the ground.

f versus t (time) graph will be asA B
B C
C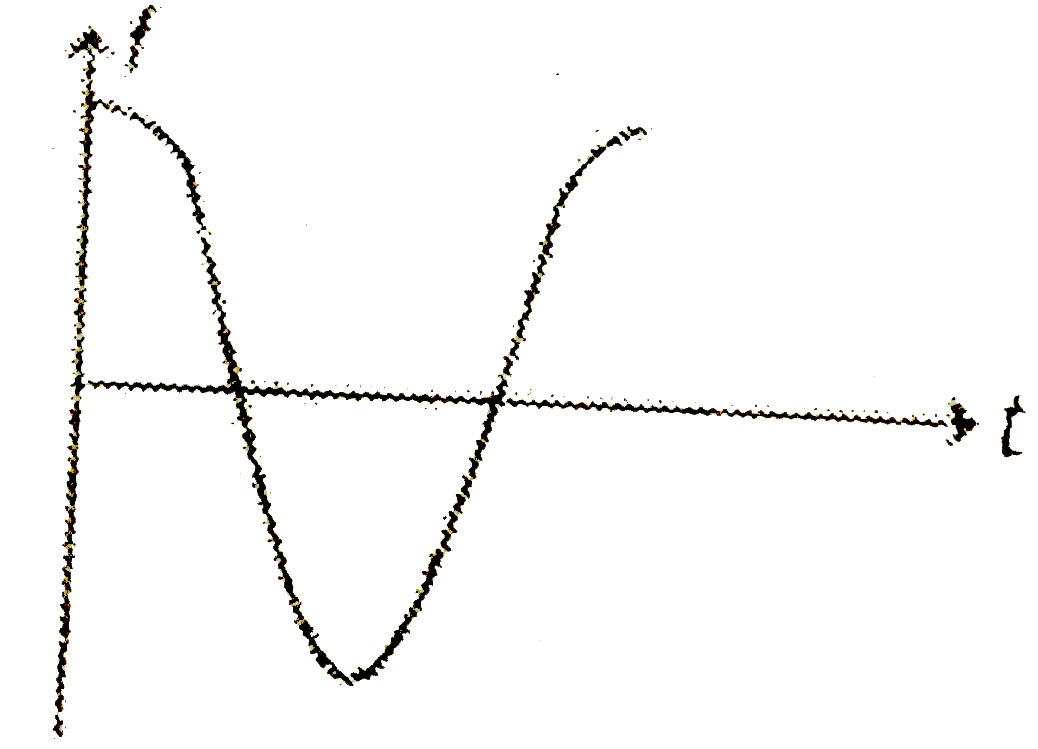 D
D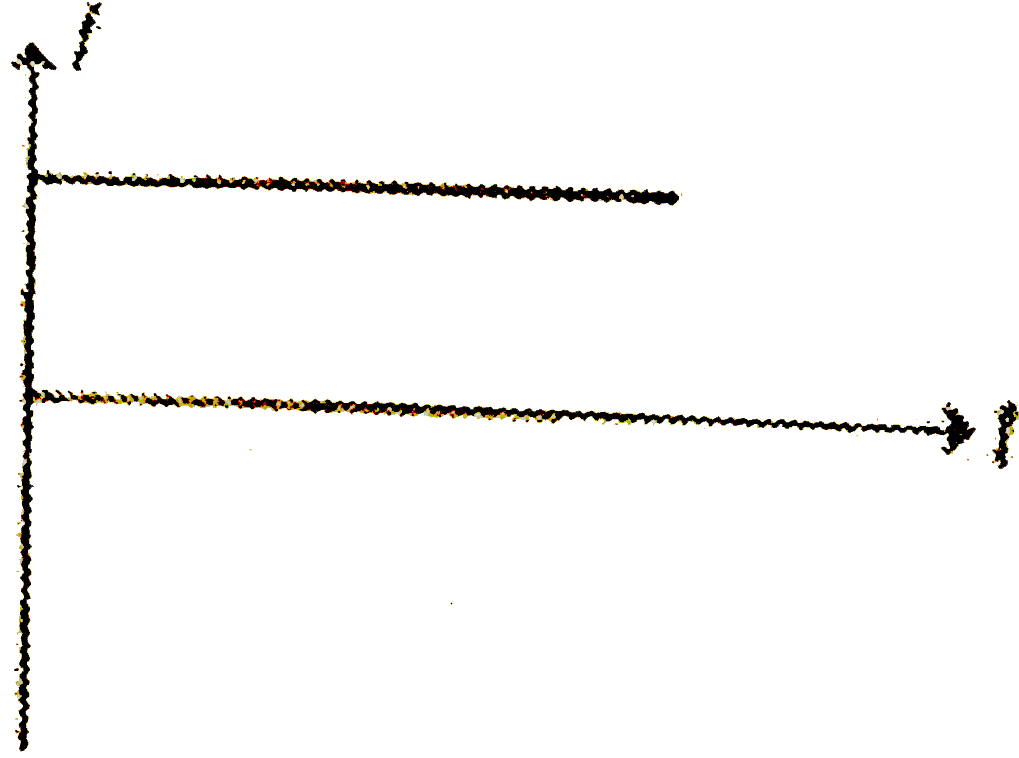
Two bars of masses m1 and m2 connected by a weightless spring of stiffness ϰ (figure) rest on a smooth horizontal plane.

Bar 2 is shifted a small distance x to the left and then released. Find the velocity of the centre of inertia of the system after bar 1 breaks off the wall.
View SolutionA block whose mass m is 680g is fastened to a spring whose spring constant k is 65N/m. The block is pulled a distance x=11cm from its equilibrium position at x=0 on a frictionless surface and released from rest at t= 0.
What is the amplitude of the oscillation?
View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.
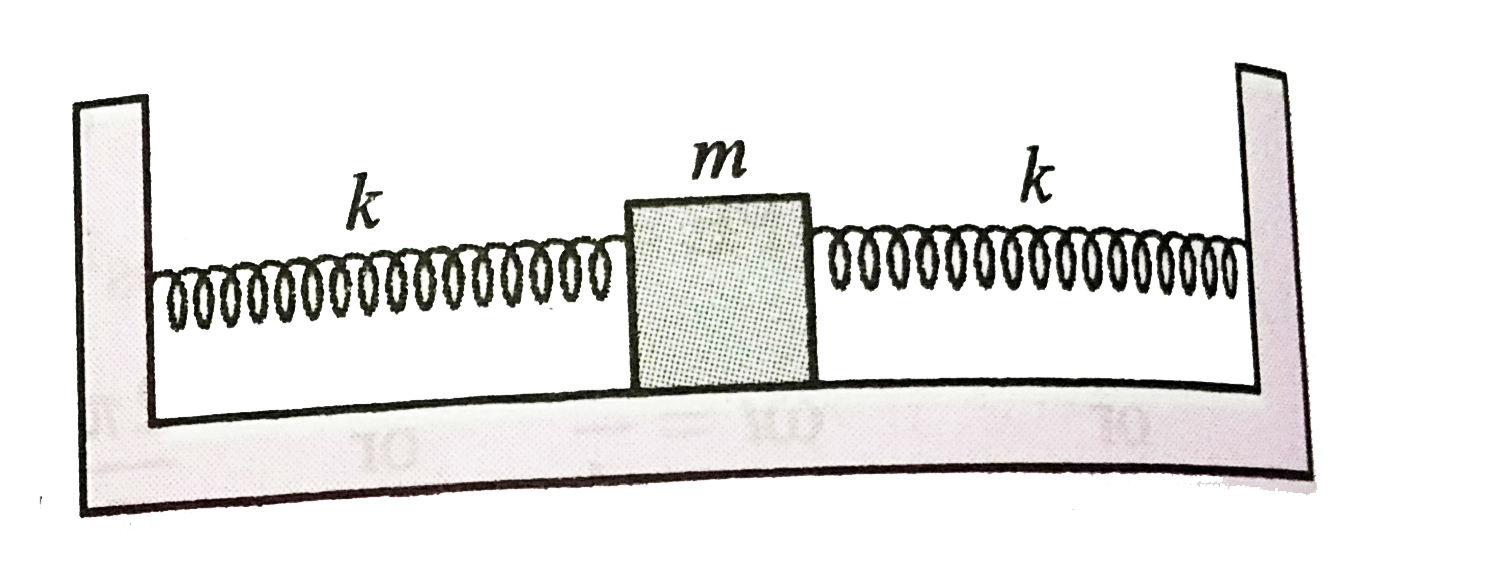
View SolutionTwo blocks of masses m1 and m2, connected by a weightless spring of stiffness k rest on a smooth horizontal plane as shown in Fig. Block 2 is shifted a small distance x to the left and then released. Find the velocity of centre of mass of the system after block 1 breaks off the wall.

View SolutionIn the figure shown the spring constant is K the mass of the upper disc is m and that of the lower disc is 3m the upper block is depressed down from its equilibrium position by a distance δ=5mg/k and released at t=0 find the velocity of m when normal reaction on 3m is mg

View Solution