At what distance is the radial probability maximum for 1s orbital ? What is this distance called ?
To find the distance at which the radial probability is maximum for the 1s orbital, we can follow these steps:
1. Understand the 1s Orbital: The 1s orbital is the simplest atomic orbital and is spherically symmetric around the nucleus. The probability density for finding an electron in this orbital varies with distance from the nucleus.
2. Radial Probability Function: The radial probability density is given by the function P(r)=|ψ(r)|2×4πr2, where ψ(r) is the wave function of the electron in the 1s orbital, and r is the distance from the nucleus. This function accounts for the volume element in spherical coordinates.
3. Maximizing the Radial Probability: To find the maximum radial probability, we need to differentiate the radial probability function P(r) with respect to r and set the derivative equal to zero. This will give us the critical points.
4. Finding the Maximum: For the 1s orbital, it can be shown that the radial probability is maximized at a distance of r=0.529 Å (angstroms). This distance is known as the Bohr radius.
5. Conclusion: Therefore, the distance at which the radial probability is maximum for the 1s orbital is 0.529 Å, and this distance is referred to as the Bohr radius.
Final Answer:
The radial probability maximum for the 1s orbital occurs at a distance of 0.529 Å, known as the Bohr radius.
---
The radial probability maximum for the 1s orbital occurs at a distance of 0.529 Å, known as the Bohr radius.
Topper's Solved these Questions
- STRUCTURE OF ATOMBOOK - PRADEEPCHAPTER - STRUCTURE OF ATOMEXERCISE - NCERT Questions And Exercises with Answers
67 Videos
- STRUCTURE OF ATOMBOOK - PRADEEPCHAPTER - STRUCTURE OF ATOMEXERCISE - NCERT Exemplar Problems With Answers, Hints and Solution (I. Multiple Choice Questions)
16 Videos
- STRUCTURE OF ATOMBOOK - PRADEEPCHAPTER - STRUCTURE OF ATOMEXERCISE - Test Your Grip (Fill in the Blanks)
24 Videos
- STATES OF MATTER: SOLID MATTERBOOK - PRADEEPCHAPTER - STATES OF MATTER: SOLID MATTEREXERCISE - COMPETITION FOCUS (ASSERTION-REASON)
17 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - MULTIPLE CHOICE QUESTION ( BASED ON PRACTICAL CHEMISTRY)
3 Videos
Similar Questions
Knowledge Check
The radius of maximum probability for 1s orbital of H atom is
A52.4nmB52.9μmC52.9ÅD52.9±There are two nodes in the radial probability distribution curve for the orbital with
An=2,n=0Bn=3,l=1Cn=4,l=3Dn=3,l=0The maximum radial probability in 1s-orbital occures at a distance when : [r0=Bohr radius]
Ar=r0Br=2r0Cr=r02D2r=r02
Find the distance at which probability of finding electron is maximum for 1s orbital in a He atom. The wave function orbital given as.
ψ1s=4a3/20e2ra0
View SolutionWhat is the maximum probability for an electron to be present within the atomic orbital ?
View SolutionGiven below as Figure 13.8 is the distance-time graph of the motion an object.
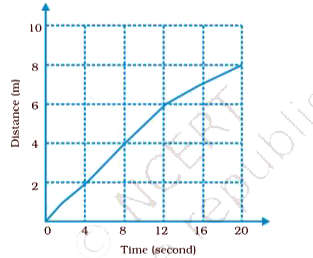
(i) What will be the position of the object at 20s?
(ii) What will be the distance travelled by the object in 12s?
(iii) What is the average speed of the object?
View SolutionWhat is the maximum number of orbitals in any energy level ?
View SolutionFor which of the following orbitals is the radial probaility density R2 the maximum at the nucleus and descrease sharply as the distance form the nucleus increases?
View Solution
