If the equation $∣ ∣ x^{2} + b x + c ∣ ∣ = k$ has four real roots, then

A

$b^{2} - 4 c > 0 and - < k < \frac{4 c - b^{2}}{4}$

B

$b^{2} - 4 c < 0 and - < k < \frac{4 c - b^{2}}{4}$

C

$b^{2} - 4 c > 0 and - k > \frac{4 c - b^{2}}{4}$

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:1

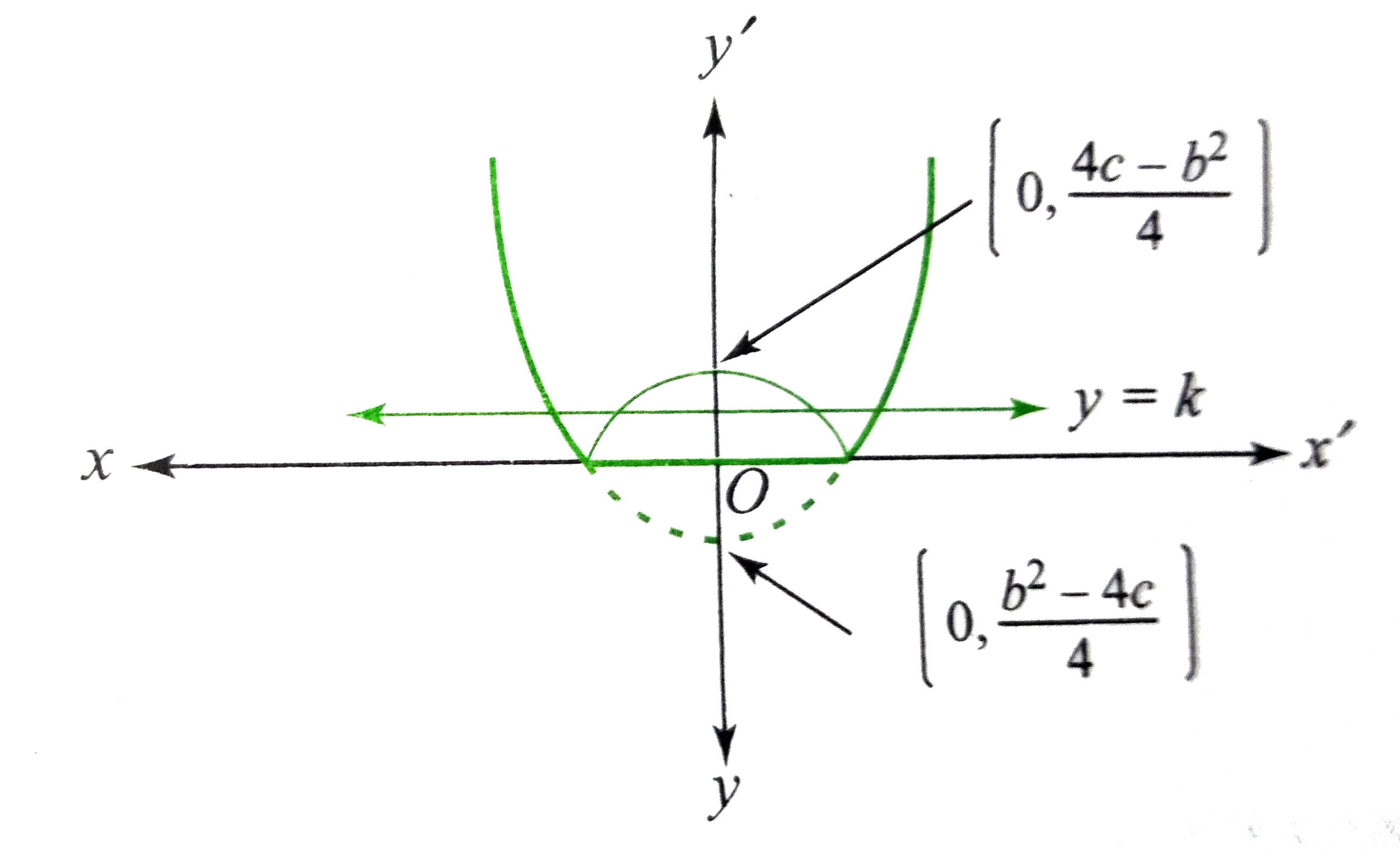
For the equation to have four real roots, the line y = k must
intersect $y = ∣ ∣ x^{2} = b x + c ∣ ∣$ at four point.
$∴ D > 0 and k \in (0, \frac{- D}{4})$

|

Updated on:21/07/2023

Class 12 MATHS QUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Let f(X) be real valued continous funcion on R defined as f(X) = $x^{2} e^{- | x |}$
The values of k for which the equation $x^{2} e^{- | x |}$ =k has four real roots are
A $0 < k < e$
B $0 < k < \frac{8}{e^{2}}$
C $0 < k < \frac{4}{e^{2}}$
Dnone of these
Question 2 - Select One
If a, b $\in$ {1, 2, 3} and the equation $a x^{2} + b x + 1 = 0$ has real roots, then
A $a > b$
B $a \leq b$
Cnumber of possible ordered pairs of (a, b) are 3
D $a < b$
Question 3 - Select One
If the equation $a x^{2} + 2 b x - 3 c = 0$ has no real roots and $\frac{3 c}{4} < a + b$ , then
A $c < 0$
B $c > 0$
C $c \geq 0$
D $c = 0$

CENGAGE-THEORY OF EQUATIONS-Exercise (Single)

Home
Ask Doubt
Profile