A vertical electric field of magnitude 4.9×105 N/C just prevents a water droplet of a mass 0.1 g from falling. The value of charge on the droplet will be : (Given g=9.8m/s2 )
1.6×10−9C
2.0×10−9C
3.2×10−9C
0.5×10−9C
The correct Answer is:B
To find the charge on the water droplet that is just prevented from falling by a vertical electric field, we can follow these steps:
1. Identify the Given Values:
- Electric field strength, E=4.9×105N/C
- Mass of the water droplet, m=0.1g=0.1×10−3kg=1.0×10−4kg
- Acceleration due to gravity, g=9.8m/s2
2. Calculate the Weight of the Droplet:
The weight W of the droplet can be calculated using the formula:
W=m⋅g
Substituting the values:
W=(1.0×10−4kg)⋅(9.8m/s2)=9.8×10−4N
3. Understand the Forces Acting on the Droplet:
The droplet is in equilibrium, meaning the upward electric force Fe due to the electric field must balance the downward weight of the droplet:
Fe=W
4. Relate Electric Force to Charge:
The electric force Fe on a charge q in an electric field E is given by:
Fe=q⋅E
Setting this equal to the weight:
q⋅E=W
5. Solve for Charge q:
Rearranging the equation to find q:
q=WE
Substituting the values we have:
q=9.8×10−4N4.9×105N/C
6. Calculate the Charge:
Performing the calculation:
q=9.8×10−44.9×105=2.0×10−9C
Final Answer:
The value of the charge on the droplet is:
2.0×10−9C
- Electric field strength, E=4.9×105N/C
- Mass of the water droplet, m=0.1g=0.1×10−3kg=1.0×10−4kg
- Acceleration due to gravity, g=9.8m/s2
The weight W of the droplet can be calculated using the formula:
W=m⋅g
Substituting the values:
W=(1.0×10−4kg)⋅(9.8m/s2)=9.8×10−4N
The droplet is in equilibrium, meaning the upward electric force Fe due to the electric field must balance the downward weight of the droplet:
Fe=W
The electric force Fe on a charge q in an electric field E is given by:
Fe=q⋅E
Setting this equal to the weight:
q⋅E=W
Rearranging the equation to find q:
q=WE
Substituting the values we have:
q=9.8×10−4N4.9×105N/C
Performing the calculation:
q=9.8×10−44.9×105=2.0×10−9C
The value of the charge on the droplet is:
2.0×10−9C
Topper's Solved these Questions
- JEE MAINS 2022Book:JEE MAINS PREVIOUS YEARChapter:JEE MAINS 2022Exercise:Physics - Section B
Explore 40 Videos
- JEE MAINS 2022Book:JEE MAINS PREVIOUS YEARChapter:JEE MAINS 2022Exercise:PHYSICS- SECTION A
Explore 19 Videos
- JEE MAINS 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAINS 2021Exercise:Physics (Section B )
Explore 10 Videos
- JEE MAINS 2023 JAN ACTUAL PAPERBook:JEE MAINS PREVIOUS YEARChapter:JEE MAINS 2023 JAN ACTUAL PAPERExercise:Question
Explore 360 Videos
Similar Questions
The bob of a simple pendulum has a mass of 60 g and a positive charge of 6×10−6C. It makes 30 oscillations in 50 s above earth's surface. A vertical electric field pointing upward and of magnitude 5×104N/C is switched on. How much time will it now take to complete 60 oscillations ? (g=10m/s2)
View SolutionA charged particle is suspended in equilibrium in a uniform vertical electric field of intensity 20000 V/m. If mass of the particle is 9.6×10−16 kg the charge on it and excess number of electrons on the particle are respectivly (g=10m/s2)
View Solution
Knowledge Check
A vertical electric field of magnitude 4.00×105NC−1 just prevents a water droplet of mass 1.000x10−4kgfrom. falling, Find the charge on the droplet.
A4.2×10−9CB2.5×10−7CC4.2×10−7CD2.5×10−9CIn the figure shown a ball is suspended from the ceiling of a room in a uniform electric field of magnitude 2×104 V/m . If the mass of the ball is 25 g and the charge on the ball is 3μC . The angle θ is (g=9.8ms−2)
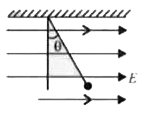 A14∘B22∘C34∘D76∘
A14∘B22∘C34∘D76∘The frictional force of the air on the body of mass 0.25kg, falling with an acceleration of 9.2 m/s2 will be (g=9.8m/s2).
A1.0NB0.55NC0.25ND0.15N
A ball of mass 500 g falls from a height of 4 m Find the K.E. of the ball when it reaches the ground. (Take g =9.8 ms2)
View SolutionFind the energy possessed by an object of mass 10 kg when it is at a height of 6 m above the ground.Given, g=9.8m/s2
View SolutionThe magnitude of electric field intensity due to a point charge is 10 N/C at a distance 1 m from it. Calculate the magnitude of charge.
View SolutionThe electric field strength in N C−1 that is required to just prevent a water drop carrying a change 1.6×10−19 C from falling under gravity is (g = 9.8 m s−2 , the mass of water drop = 0.0016 g )
View SolutionA charge produce an electric field of 1 N/C at a point distant 0.1 m from it. The magnitude of charge is
View Solution