A solid sphere spinning about a horizontal axis with an angular velocity ω is placed on a horizontal surface. Subsequently it rolls without slipping with an angular velocity of :
2ω5
7ω5
2ω7
ω
The correct Answer is:C
Initial sphere is slipping and finally it starts rolling during its motion τ about point of contact is zero. Angular momentum of sphere about point of contact remains conserved

Iω=(I+MR2)ω.
⇒25MR2ω=(25MR2+MR2)ω⇒ω.=2ω7
Similar Questions
If a ring, disc, hollow sphere and solid sphere rolling horizontally without slipping with the same velocity on a surface, then translational kinetic energy is more for
View SolutionA solid sphere is rotating with a constant angular velocity about an axis touching the surface. Its angular momentum will be:
View SolutionA solid sphere and a hollow sphere of equal mass and radius are placed over a rough horizontal surface after rotating it about its mass centre with same angular velocity ω0. Once the pure rolling starts let v1 and v2 be the linear speeds of their centres of mass. Then
View SolutionA solid sphere of mass m= 500gm is rolling without slipping on a horizontal surface. Find kinetic energy of a sphere if velocity of centre of mass is 5 cm/sec
View Solution{:(" Column-I"," Column-II"),("(A) Solid sphere rolling with slipping","(P) Total kinetic energy is conserved on a rough horizontal surface"),("(B) Solid sphere in pure rolling on a rough horizontal ","(Q) Angular momentum about CM is conserved"),("(C) Solid sphere in pure rolling on a smooth horizontal surface","(R) Angular momentum about a point on contact surface is conserved"), ("(D) Solid sphere in pure rolling on a rough incline","(S) Momentum is conserved"):}
View Solution
solid sphere of radius r is gently placed on a rough horizontal ground with an initial angular speed ω0 and no linear velocity. If the coefficient of friction is μ, find the time t when the slipping stops. in addition state the linear velocity v and angular velocity ω at the end of slipping
View SolutionA uniform solid sphere of radius R , rolling without sliding on a horizontal surface with an angular velocity omega_(0) , meets a rough inclined plane of inclination theta = 60^@ . The sphere starts pure rolling up the plane with an angular velocity omega Find the value of omega .
View SolutionA solid cylinder is rolling without slipping with velocity of its centre of mass v and angular velocity about its centre of mass ω on a horizontal frictionless surface as shown in figure. If it collides with a frictionless vertical wall X, then after collision its velocity and angular velocity respectively become
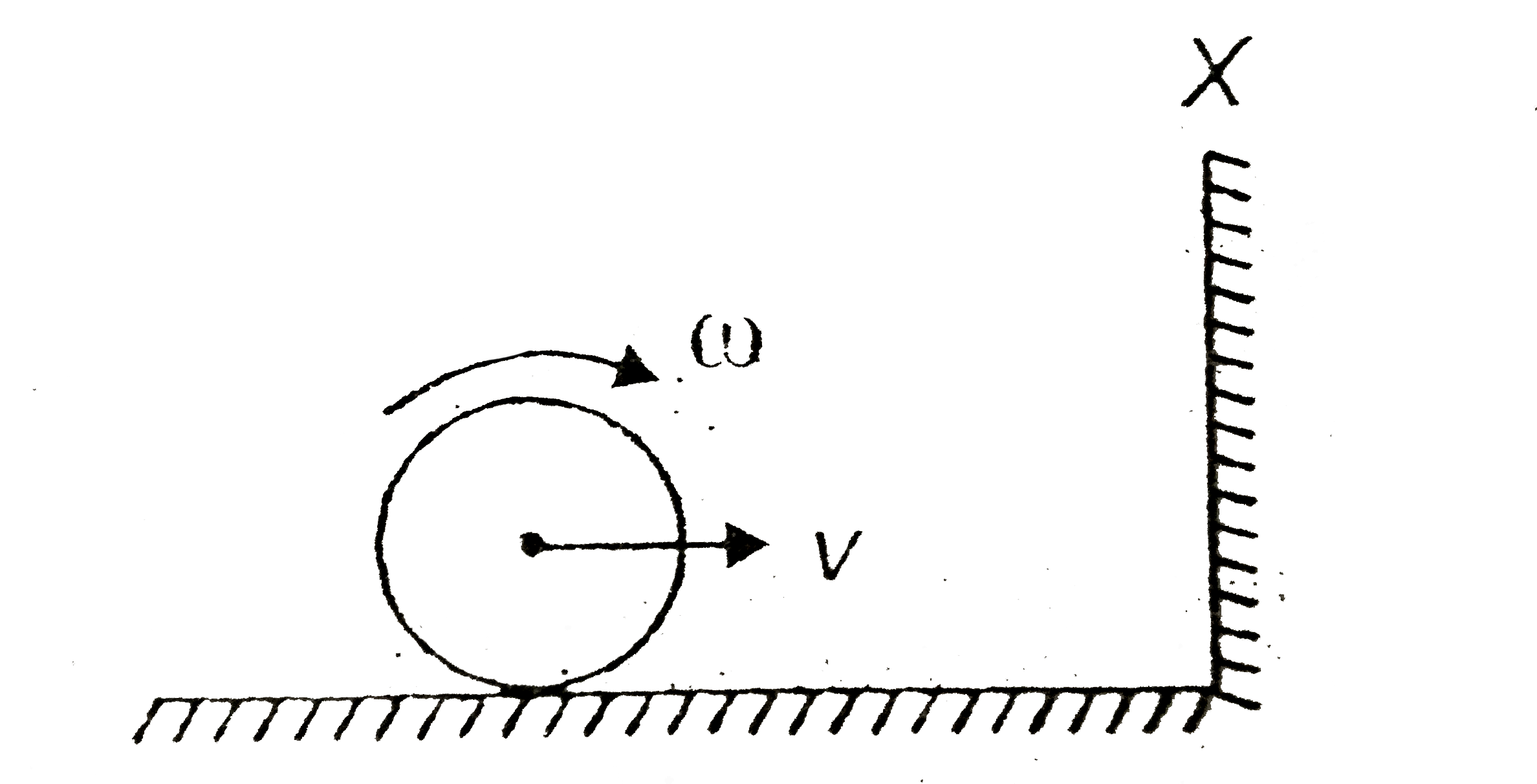
View Solution