The capacitor of capacitance C in the circuit shown in fully charged initially. Resistance is R.–
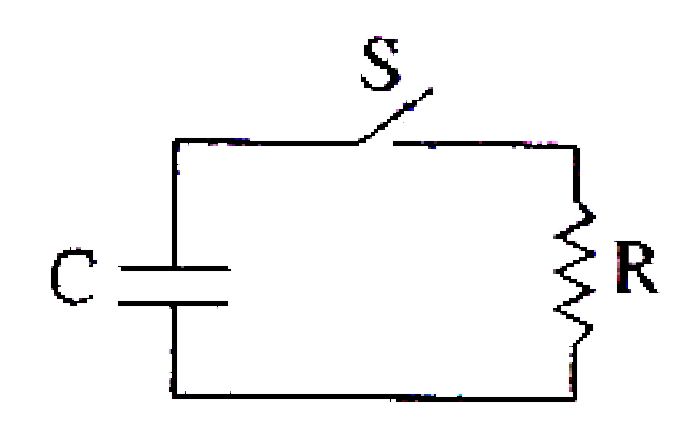
After the switch S is closed, the time taken to reduce the stored energy in the capacitor to half its initial value is
RC2
RCln2
2RCln2
RCln22
The correct Answer is:D
Q=Q0e−t/RC⇒Q0√2=Q0e−t/RC⇒1√2=e−tRC
ln1√2=−tRC⇒tRC=ln√2⇒t=RCln22

Similar Questions
प्रदर्शित चित्र में संधारित्र पर प्रारंभिक आवेशों को दिखाया गया है | स्विच S बंद करने के पश्चात ज्ञात कीजिए -
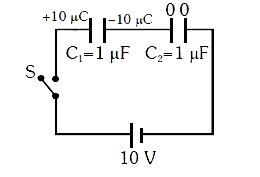
संधारित्र C1 पर आवेश
View Solutionप्रदर्शित चित्र में संधारित्र पर प्रारंभिक आवेशों को दिखाया गया है | स्विच S बंद करने के पश्चात संधारित्र.C2 पर आवेश ज्ञात कीजिए -

View SolutionLet C be the capacitance of a capacitor discharging through a resistor R. Suppose t_1 is the time taken for the energy stored in the capacitor to reduce to half its initial value and t_2 is the time taken for the charge to reduce to one-fourth its initial value. Then the ratio t_1//t_2 will be
View SolutionIn the circuit shown, the capacitor C1 is initially charged with charge q0. The switch S is closed at time t = 0 . The charge on C2 after time t is

View SolutionInitially, both capacitors are uncharged. A long time after the switch S is closed, the potential energy stored in the capacitor of capacitance 2μFis______×10−6J.
View SolutionThe capacitor shown in the figure is initially unchanged, the battery is ideal. The switch S is closed at time t= 0, then the time after which the energy stored in the capacitor becomes one - fourth of the energy stored in it in steady - state is :
View SolutionA charged capacitor of capacitance C is discharging through a resistor of resistance R. At what time the charge on the capacitor is equal to one half of its initial value?
View SolutionIn the circuit shown, the capacitor is initially uncharged. The switch S is closed at t = 0. Time after which voltage across capacitor and resistor are equal is:
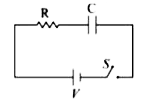
View Solution

