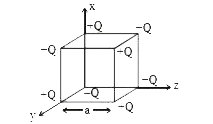
A cube of side 'a' has point charges +Q located at each of its vertices except at the origin where the charge is -Q. The electric field at the centre of cube is :
2Q3√3πε0a2(ˆx+ˆy+ˆz)
−Q3√3πε0a2(ˆx+ˆy+ˆz)
−2Q3√3πε0a2(ˆx+ˆy+ˆz)
Q3√3πε0a2(ˆx+ˆy+ˆz)
The correct Answer is:C
We can replace −Q charge at origin by +Q and −2Q. Now due to +Q charge at every corner of cube. Electric field at center of cube is zero so now net electric field at center is only due to −2Q charge at origin
→E=kq→rr3=1(−2Q)a2(ˆx+ˆy+ˆz)4πε0(a2√3)3
→E=−2Q(ˆx+ˆy+ˆz)3√3πa2ε0
Similar Questions
A cube of side b has a charge q at each of its vertices. Determine the potential and electric field due to this charge array at the centre of the cube.
View SolutionA cube of side b has a charge q at each of its vertices. Determine the potential and electric field due to this charge array at the center of the cube.
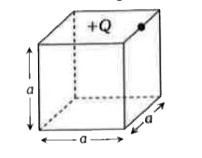
View Solutionचित्र में +Q आवेश घन के एक किनारे पर स्थित है | तब आवेश +Q के कारण घन पर विद्युत अभिवाह का मान है
View SolutionA cube of side b has a charge g at each of its vertices. Determine the potential and electric field due to this charge array at the centre of the cube.
View SolutionA cube of side b has a charge q at each of its vertices. Determine the potential and electric field due to this charge array at the centre of the cube.
View SolutionA cube of side x has a charge q at each of its vertices. Determine the potential due to this charge array at the center of the cube.
View SolutionA cube of side 'a' has point charges +Q located at each of its vertices except at the origin where the charge is -Q. The electric field at the centre of cube is :

View SolutionA cube of side b has a charge q at each of its vertices. Determine the electric field due to this charge array at the center of the cube.
View Solution
