One end of a spring of force constant k is fixed to a vertical wall and the other to a blcok of mass m resting on a smooth horizontal surface. There is another wall at distance x0 from the block. The spring is then compressed by 2x0 and released. The time taken to strike the wall is
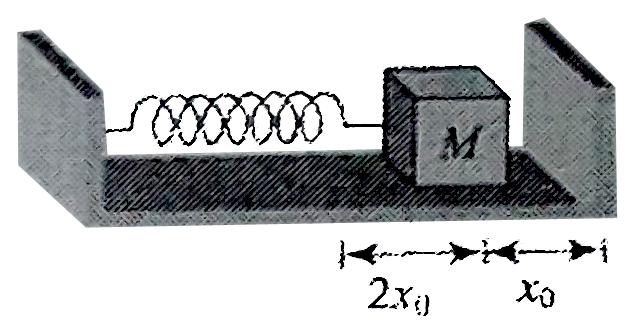
16π√km
√km
2π3√mk
π4√km
The correct Answer is:C

tAC=tAB+tBC
⇒tAC=T4+tBC
Now,
BC=ABsin(2πT)tBC ⇒BCAB=sin(2πT)tBC
⇒12=sin(2πT)tBC [as BCAB=12]
⇒sinπ6=sin(2πT)tBC⇒π6=2πTtBC
⇔tBC=T12
So, tAC=T4+T12=T3⇒tAC=2π3√mk.
Similar Questions
One end of a spring with a k force constant is attached to the wall and at the other end a mass of m mass that is placed on a smooth horizontal plane is attached. From the body x_(0) There is another wall at the distance. Spring 2x_(0) The length is pressed and released. Time taken to hit the body against the wall
View SolutionOne end of a light spring of force constant K is fixed to the wall and other end is tied to a block placed on a smooth horizontal surface. In a displacement, the work done by the spring is 1/2Kx^(2) . The possible cases may be
View SolutionA block of mass .M. moving on the firctionless , horizontal surface collides with the spring of spring constant .k.. The other end of the spring is rigidly fixed to a vertical wall. If the spring is compressed by L, the maximum momentum of the block after collision is :
View SolutionA block of mass 'M' moving on the firctionless horizontal surface collides with the spring of spring constant 'k'. The other end of the spring is rigidly fixed to a vertical wall. If the spring is comressed by L, the maximum momentum of the block after collision is :
View SolutionA block of mass 'M' moving on the firctionless horizontal surface collides with the spring of spring constant 'k'. The other end of the spring is rigidly fixed to a vertical wall. If the spring is comressed by L, the maximum momentum of the block after collision is :
View SolutionA block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is mu then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
View SolutionIn the figure shown, a spring mass system is placed on a horizontal smooth surface in between two vertical rigid walls W1 and W2. One end of spring is fixed with wall W1 and other end is attached with mass m which is free to move. Initially, spring is tension free and having natural length l0. Mass m is compressed through a distance a and released. Taking the collision between wall W2 and mass m as elastic and K as spring constant, the average force exerted by mass m on wall W2 in one oscillation of block is
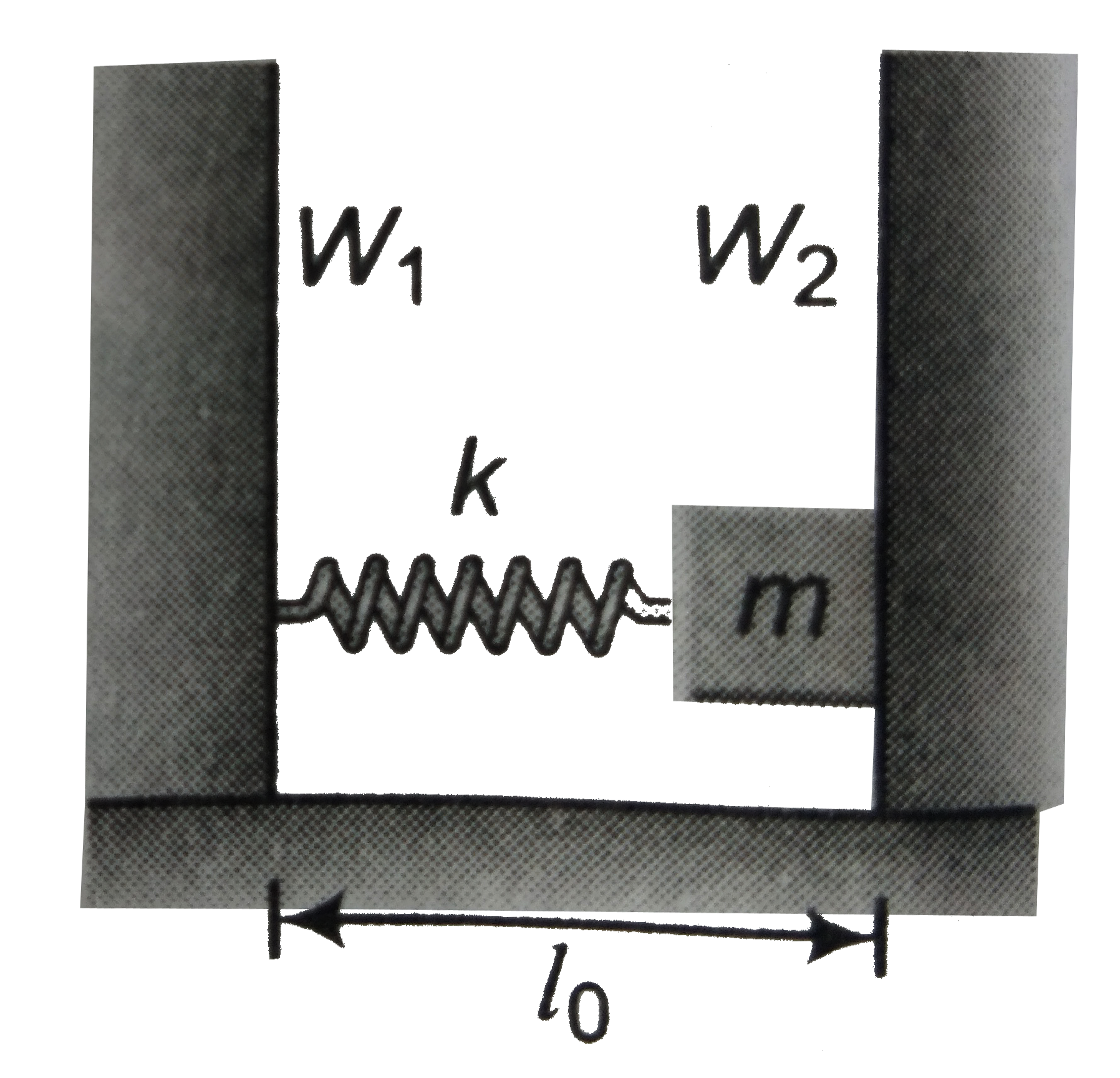
View Solution
One end of a spring of force constant K is fixed to a vertical wall and the other to a body of mass m resting on a smooth horizontal surface. There is another wall at a distance x0 from the body. The spring is then compressed by 3x0 and released. The time taken to strike the wall from the instant of release is (given sin−1(13)=(π9))
View Solution
