Four identical particles of equal masses 1kg made to move along the circumference of a circle of radius 1 m under the action of their own mutual gravitational attraction. The speed of each particle will be :

A

$\sqrt{\frac{G}{2} (2 \sqrt{2} - 1)}$

B

$\sqrt{G (1 + 2 \sqrt{2})}$

C

$\sqrt{\frac{G}{2} (1 + 2 \sqrt{2})}$

D

$\frac{\sqrt{(1 + 2 \sqrt{2}) G}}{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

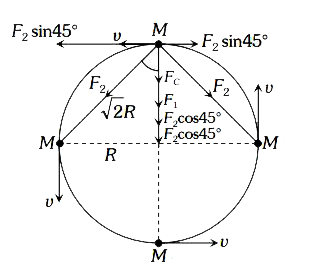implies By resolving force $F_{2}$ , we get
$\Rightarrow F_{1} + F_{2} {cos 45}^{\circ} + F_{2} {cos 45}^{\circ}$
$\Rightarrow F_{1} + 2 F_{2} {cos 45}^{\circ} = F_{c}$
$F_{c}$ = centripetal force = $\frac{M v^{2}}{R}$
$\Rightarrow \frac{G M^{2}}{{(2 R)}^{2}} + ⎡ ⎢ ⎢ ⎢ ⎣ \frac{2 G M^{2}}{{(\sqrt{2} R)}^{2}} {cos 45}^{\circ} ⎤ ⎥ ⎥ ⎥ ⎦ = \frac{M v^{2}}{R}$
$\Rightarrow \frac{G M^{2}}{4 R^{2}} + \frac{2 G M^{2}}{2 \sqrt{2} R^{2}} = \frac{M v^{2}}{R}$
$\Rightarrow \frac{G M}{4 R} + \frac{G M}{\sqrt{2} . R} = v^{2} \Rightarrow v = \sqrt{\frac{G M}{4 R} + \frac{G M}{\sqrt{2} . R}}$
$\Rightarrow v = \sqrt{\frac{G M}{R} [\frac{1 + 2 \sqrt{2}}{4}]}$
$\Rightarrow v = \frac{1}{2} \sqrt{\frac{G M}{R} (1 + 2 \sqrt{2})}$
(Given : mass = 1kg, radius = 1m)
$\Rightarrow v = \frac{1}{2} \sqrt{\frac{G M}{R} (1 + 2 \sqrt{2})}$

Updated on:21/07/2023

|

Class 11 PHYSICS GRAVITATION

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile