A straight of length L extends from x=a to x=L+a. the gravitational force it exerts on a point mass 'm' at x=0, if the mass per unit length of the rod is is given by :
The correct Answer is:C
Similar Questions
A rod of length L is placed along the X-axis between x=0andx=L. The linear density (mass/length) ρ of the rod varies with the distance x from the origin as ρ=a+bx. a.) Find the SI units of a and b b.) Find the mass of the rod in terms of a,b, and L.
View SolutionA straight of length L extends from x=a to x=L+a. the gravitational force it exerts on a point mass 'm' at x=0, if the mass per unit length of the rod is A+Bx^(2), is given by :
View SolutionA straight rod of length L extends from x= a to x= L+a. The linear mass density of the rod varies with x- coordinate is lamda=A + bx^(2) . The gravitational force experienced by a point mass m at x= 0 is
View SolutionA straight rod of length L has one of its end at the origin and the other at X=L . If the mass per unit length of the rod is given by Ax where A is constant, where is its centre of mass?
View SolutionA straight rod of length L has one of its end at the origin and the other at X=L . If the mass per unit length of the rod is given by Ax where A is constant, where is its centre of mass?
View SolutionA straight rod of length L has one of its end at the origin and the other at X=L. If the mass per unit length of the rod is given by Ax where A is constant, where is its centre of mass?
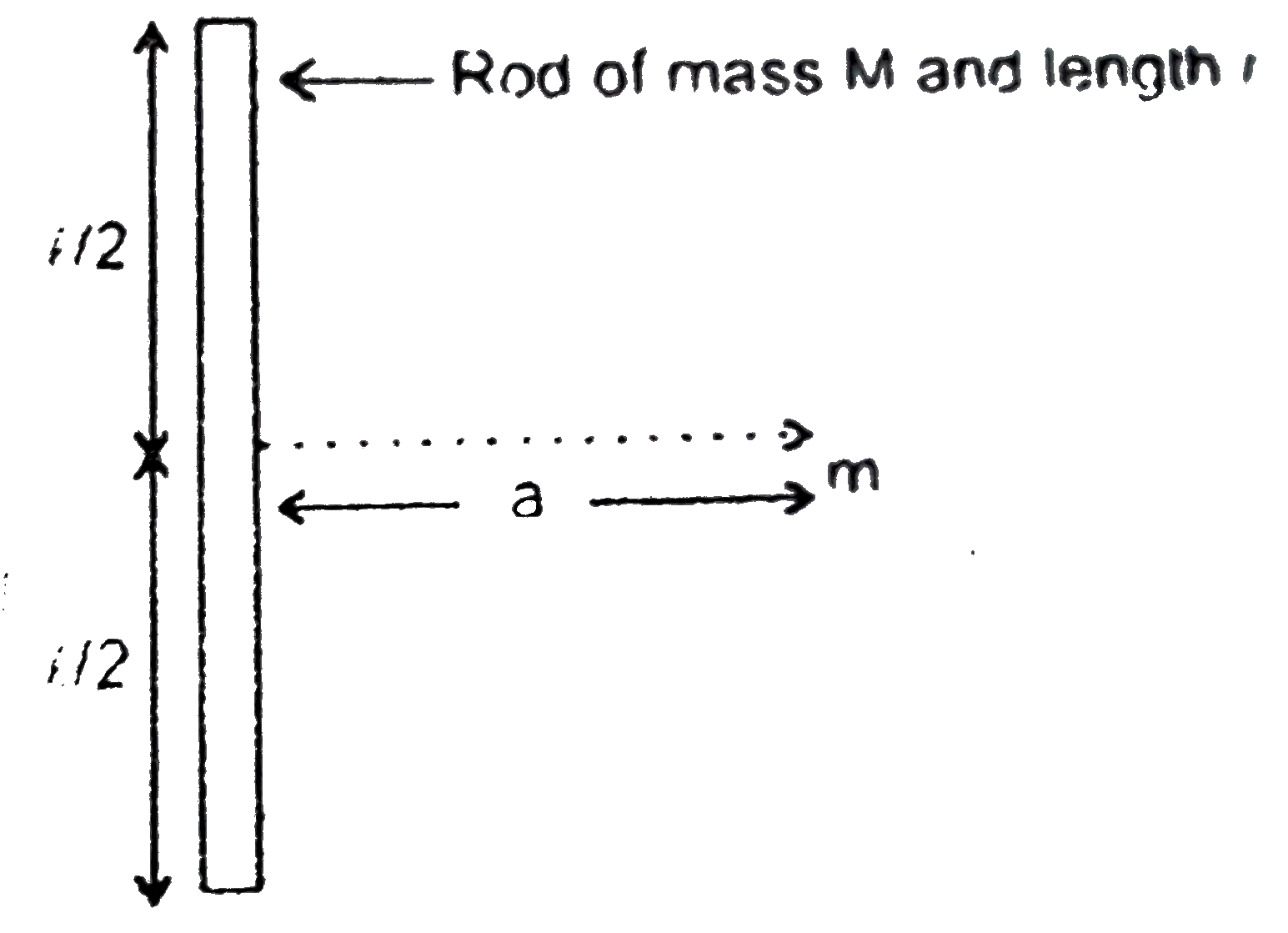
View SolutionCalculate the force exerted by point mass m on rod of uniformly distributed mass M and length l (Placed as shown in figure)
 .
.
View SolutionA non -uniform rod of length l having mass density lamda(x) = (A+Bx^2) is placed - x - axis with its ends at , (a,0) and (a+ l, 0) . The force it would exert on a point mass m kept at the origin is
View Solution