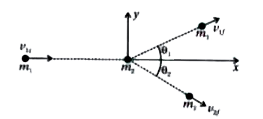
Consider the collision depicted in Figure, to be between two billiard balls with equal masses m1=m2. The first ball is called the cue and the second ball is called the target. The billiard player wants to sink the target ball in a corner pocket, which is at an angle θ2=ϕ=37∘. Assume that the collision is elastic and that friction and rotational motion are not important. Obtain θ1=θ .
35∘
50∘
55∘
60∘
The correct Answer is:C
θ1+θ2=90∘
⇒35+θ2=90∘
⇒θ2=55∘
Similar Questions
A billiards player hits a stationary ball by an identical ball to pocket the target ball in a corner pocket that is at an angle of 35∘ with respect to the direction of motion of the first ball. Assuming the collision as elastic and that friction and rotational motion are not important, the angle made by the target ball with repect to the incoming ball is
View Solutionमान लीजिए की चित्र में चित्रित संघट्ट बिलियर्ड की समान द्रव्यमान (m1=m2) वाली दो गेंदों के मध्य हुआ है। जिसमे प्रथम गेंद क्यू (डण्डा) कहलाती है और द्वितीय गेंद 'लक्ष्य' कहलाती है। खिलाडी लक्ष्य गेंद को θ2=37∘ के कोण पर कोने में लगी थैली में गिरना चाहता है। यहाँ मान लीजिए कि संघट्ट प्रत्यास्थ है तथा घर्षण और घूर्णन गति महत्त्वपूर्ण नहीं है। कोण θ1 ज्ञात कीजिए।
View SolutionConsider the collision depicted in Fig. 6.10. to be between two billiard balls with equal masses m1=m2. The first ball is called the cue while the second ball is called the target. The billiard player wants to 'sink' the target ball in a corner pocket, which is at an angle θ2=37∘. Assume that the collision is elastic and that friction and rotational motion are not important. Obtain θ1.
View SolutionConsider the collision depicted in Fig. 6.10. to be between two billiard balls with equal masses m_(1)= m_(2) . The first ball is called the cue while the second ball is called the target. The billiard player wants to 'sink' the target ball in a corner pocket, which is at an angle theta_(2)= 37^(@) . Assume that the collision is elastic and that friction and rotational motion are not important. Obtain theta_(1) .
View SolutionConsider the collision depicted in Fig. 6.10. to be between two billiard balls with equal masses m1=m2. The first ball is called the cue while the second ball is called the target. The billiard player wants to 'sink' the target ball in a corner pocket, which is at an angle θ2=37∘. Assume that the collision is elastic and that friction and rotational motion are not important. Obtain θ1.
View Solutionविलियर्ड में खिलाडी एक स्थिर बॉल को एक समान बॉल द्वारा पॉकेट को लक्ष्य करके टकराता है। लक्षित बॉल पॉकेट के निकट है , जो प्रथम बॉल की गति की दिशा से 35∘ का कोण बनाती है यह माना जाए की संघट्ट की प्रत्यास्थ है तथा घर्षण व घूर्णन गति महत्वपूर्ण नहीं है। अतः लक्षित बॉल द्वारा आने वाली बॉल (प्रथम ) के साथ अंतरित कोण है
View SolutionConsider the collision depicted in figure to be between two billiard balls are equal masses m_(1)=m_(2) . The first ball is called the cue while the second ball is called the target . The billiard player wants to 'sink ' the target ball in a corner pocket , which is at angle theta_(2) = 37^(@) .Assume that the collision is elastic and that friction and rotational motion are not important . Obtain theta_(1) .
View SolutionConsider the collision depicted in gif . To be between two billiard balls with equal masses second ball is called the target .The billiard player wants to 'sink' the target ball in corner pocket , what is at an angle θ2=32∘
Assume that collision is elastic and that friction and rotational motion are not important Obtain θ1.
View Solution