The stream of a river is flowing with a speed of 2 km/h. A swimmer can swim at a speed of 4 km/h. What should be the direction of the swimmer with respect to the flow of the river to cross the river straight?
90∘
120∘
60∘
150∘
The correct Answer is:B
→vmr=→vm−→vr
→vm=→vmr+→vr
sinθ=|→vr||→vmr|=24=12
θ=30∘

Angle with downstream is 120∘
Similar Questions
The stream of a river is flowing with a speed of 2 km/hr. A swimmer can swim at speed of 4 km/hr. What should be the direction of the swimmer with respect to the flow of the river to cross the river straight ?
View Solutionएक नदी की धारा 2km/h की गति से बह रही है। एक तैराक 4km/h की गति से तैर सकता है। तैराक का नदी के प्रति तैरने की वह दिशा, जिससे वह नदी को सीधा पार कर सके, क्या होगी?
View SolutionA swimmer's speed in the direction of flow of river is 16 km h^(- 1) . Against the direction of flow of river, the swimmer's speed is 8 km h^(-1) . The swimmer's speed in still water and the velocity of flow of the river respectively are
View SolutionIf the speed of a swimmer in still water is 9 km/h. Find the down stream speed of the swimmer,whenthe river is flowing with the speed of 6 km/h.
View SolutionA swimmer wishes to cross a 500m river flowing at 5kmh−1. His speed with respect to water is 3kmh−1. The shortest possible time to cross the river is.
View SolutionThe stream of a river is flowing with a speed of 2km/h. A swimmer can swim at a speed of 4km/h. What should be the direction of the swimmer with respect to the flow of the river ot cross the river straight ?
View SolutionA swimmer's speed in the direction of flow of river is 16 km/h. Against the direction of flow of river, the swimmer's speed is 8 km/h. The swimmer's speed in still water and the velocity of now of the river respectively are
View SolutionA river is flowing with a speed of 1kmh−1 A swimmer wants to go point C starting from A. He swims with a speed of 5kmh−1 at an angle θ w.r.t. the river flow. If AB=BC=400m, at what angle with the river bank should the swimmer swim ?
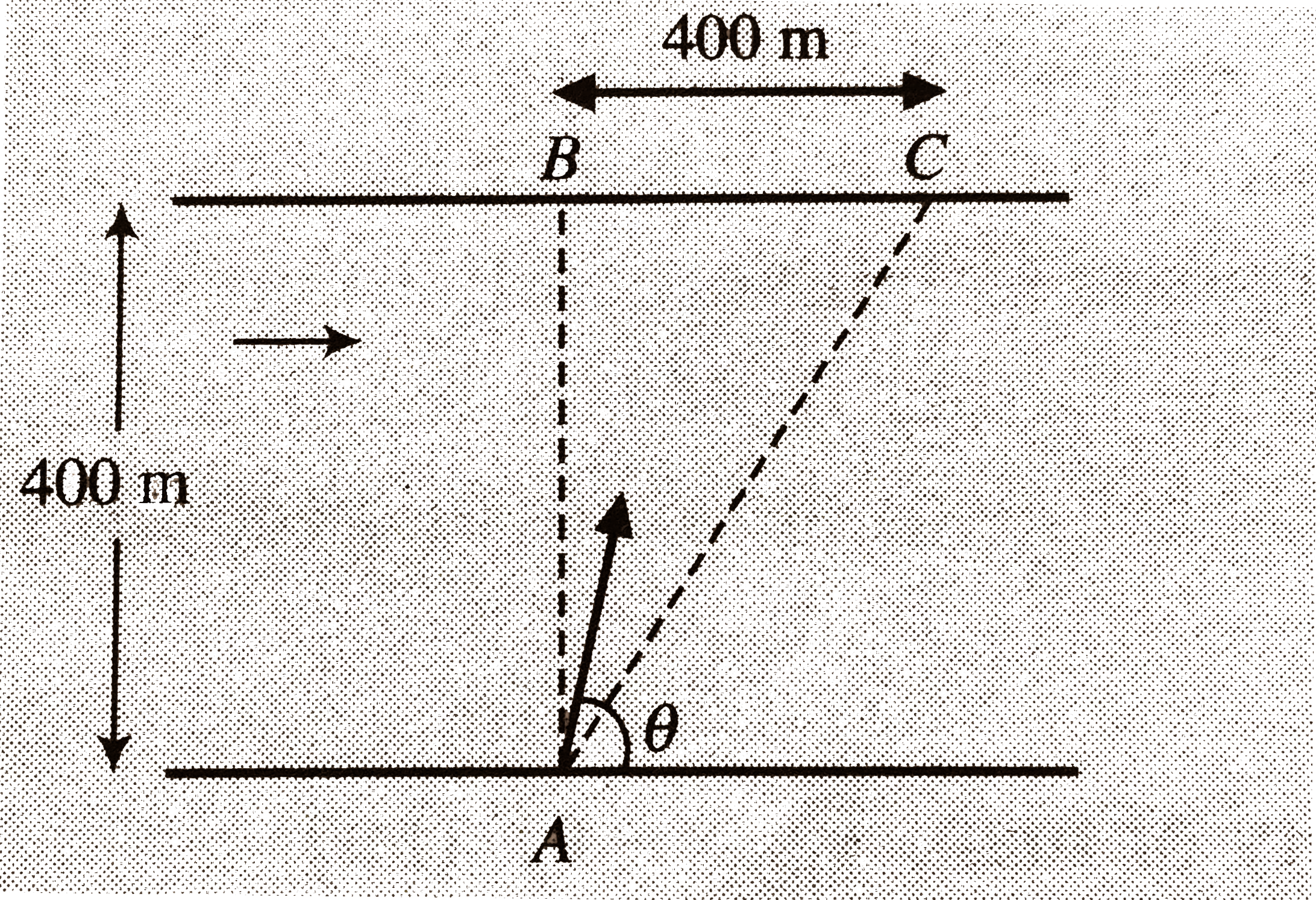 .
.
View Solution
