A machine gun is mounted on the top of a tower of height $h$ . At what angle should the gun be inclined to cover a maximum range of firing on the ground below ? The muzzle speed of bullet is $150 m s^{- 1}$ . Take $g = 10 m s^{- 2}$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $43.7$

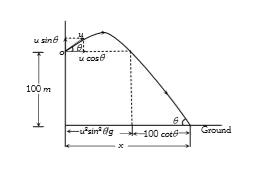
Let u be the muzzle speed of the bullet fired from the gun (on the top of the tower ) at an angle $θ$ with the horizontal as shown in Figure
Clearly, the total range of firing on the ground is
$x = \frac{u^{2} sin 2 θ}{g} + 100 cot θ$
$∴ \frac{d x}{d θ} = \frac{u^{2} \times 2 cos 2 θ}{g} + 100 \times (- cos e c^{2} θ)$
$= \frac{2 u^{2}}{g} (1 + 2 {sin}^{2} θ) - \frac{100}{{sin}^{2} θ}$
$= 4500 - 9000 {sin}^{2} θ - \frac{100}{{sin}^{2} θ}$

For x to be maximum
$\frac{d x}{d θ} = 0$
or $4500 - 9000 {sin}^{2} θ - \frac{100}{{sin}^{2} θ} = 0$
or $90 {sin}^{2} θ - 45 {sin}^{2} θ + 1 = 0$
${sin}^{2} θ = \frac{45 \pm \sqrt{{(- 45)}^{2} - 4 \times 90 \times 1}}{2 \times 90}$
$= \frac{45 \pm 40.80}{180}$
Taking only positive sign,
${sin}^{2} θ = 0.4767$
or $sin θ = 0.6904 or θ = 43 . 7^{\circ}$

|

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile