A solid sphere rolls without slipping, first horizontal and then up to a point X at height h on an inclined plane before rolling down, as shown.
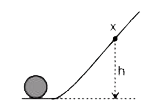
The initial horizontal speed of the sphere is
√10gh7
√7gh5
√5gh7
√2gh
The correct Answer is:A
mgh=12mv2+1225mR2v2R2⇒mgh=710mv2
⇒v=√10gh7.
Topper's Solved these Questions
- ROTATIONAL MOTIONBook:ERRORLESSChapter:ROTATIONAL MOTIONExercise:PAST YEARS QUESTIONS
Explore 70 Videos
- ROTATIONAL MOTIONBook:ERRORLESSChapter:ROTATIONAL MOTIONExercise:ASSERTION AND REASON
Explore 25 Videos
- ROTATIONAL MOTIONBook:ERRORLESSChapter:ROTATIONAL MOTIONExercise:NCERT BASED QUESTIONS (Work, Energy and Power)
Explore 44 Videos
- NEWTON'S LAWS OF MOTIONBook:ERRORLESSChapter:NEWTON'S LAWS OF MOTIONExercise:ASSERTION & REASON
Explore 18 Videos
- SIMPLE HARMONIC MOTIONBook:ERRORLESSChapter:SIMPLE HARMONIC MOTIONExercise:Assertion & Reason
Explore 15 Videos
Similar Questions
Knowledge Check
A solid sphere rolls down without slipping from rest on a 30∘ incline. Its linear acceleration is
A5g/7B5g/14C2g/3Dg/3A 1 Kg solid sphere rolls without slipping on a rough horizontal suface as shown in figure. Select incorrect alternative
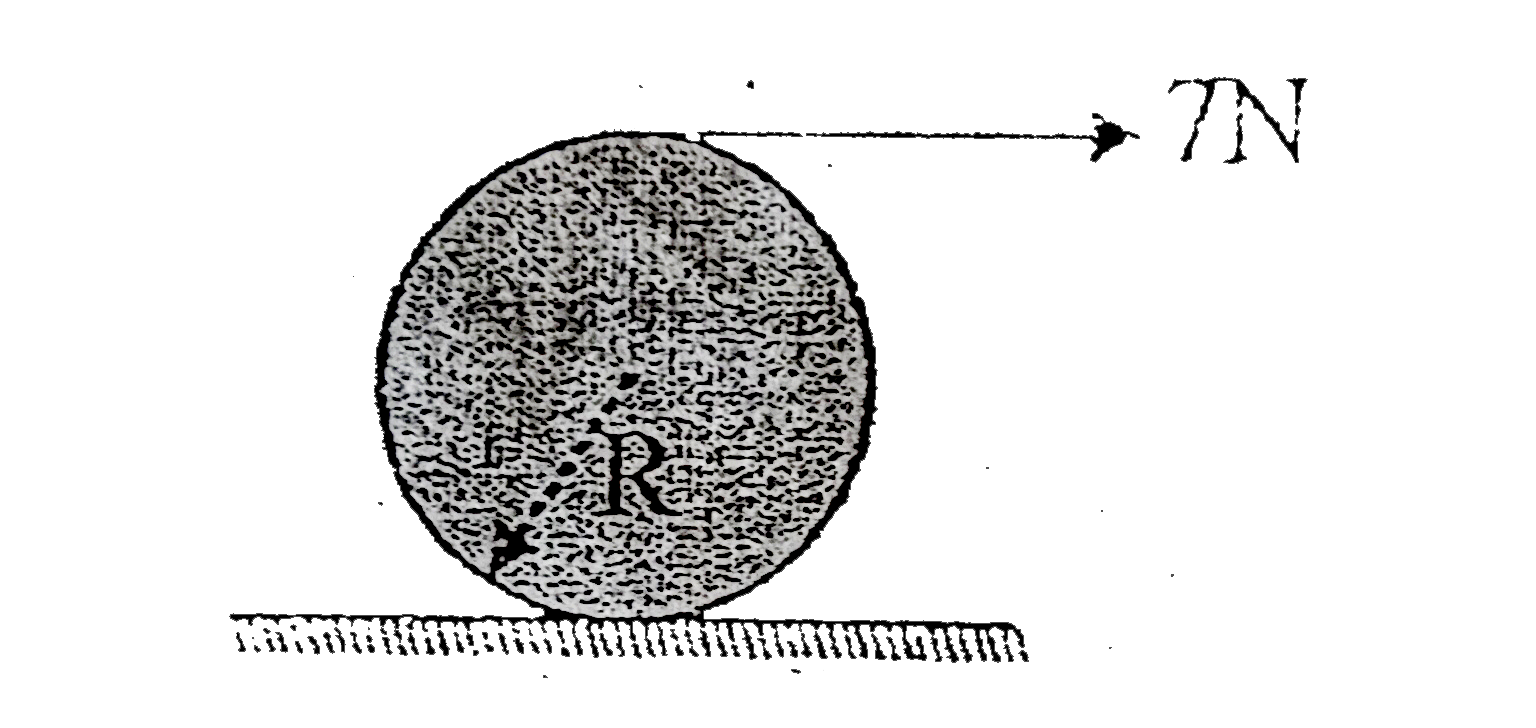 AThe acceleration of the centre of the sphere is 9.8m/s2BThe acceleration of the centre of the sphere is 10m/s2CThe direction of frictional force on the sphere is towards rightDThe value of frictional force is 3N
AThe acceleration of the centre of the sphere is 9.8m/s2BThe acceleration of the centre of the sphere is 10m/s2CThe direction of frictional force on the sphere is towards rightDThe value of frictional force is 3NA solid sphere rolls down two different inclined planes of the same height but of different inclinations
AIn both cases the final speeds and time of descent will be sameBThe final speeds will be same but time of descent will be differentCThe final speed will be different but time of descent will be sameDThe final speeds and time of descent both will be same
A solid sphere rolls on a smooth horizontal surface at 10m/s and then rolls up a smooth inclined plane of inclination 30∘ with horizontal. The mass of the sphere is 2kg. Find the height attained by the sphere before it stops (in m).
View SolutionA uniform solid sphere of radius R, rolling without sliding on a horizontal surface with an angular velocity ω0, meets a rough inclined plane of inclination θ=60∘. The sphere starts pure rolling up the plane with an angular velocity ω Find the value of ω.
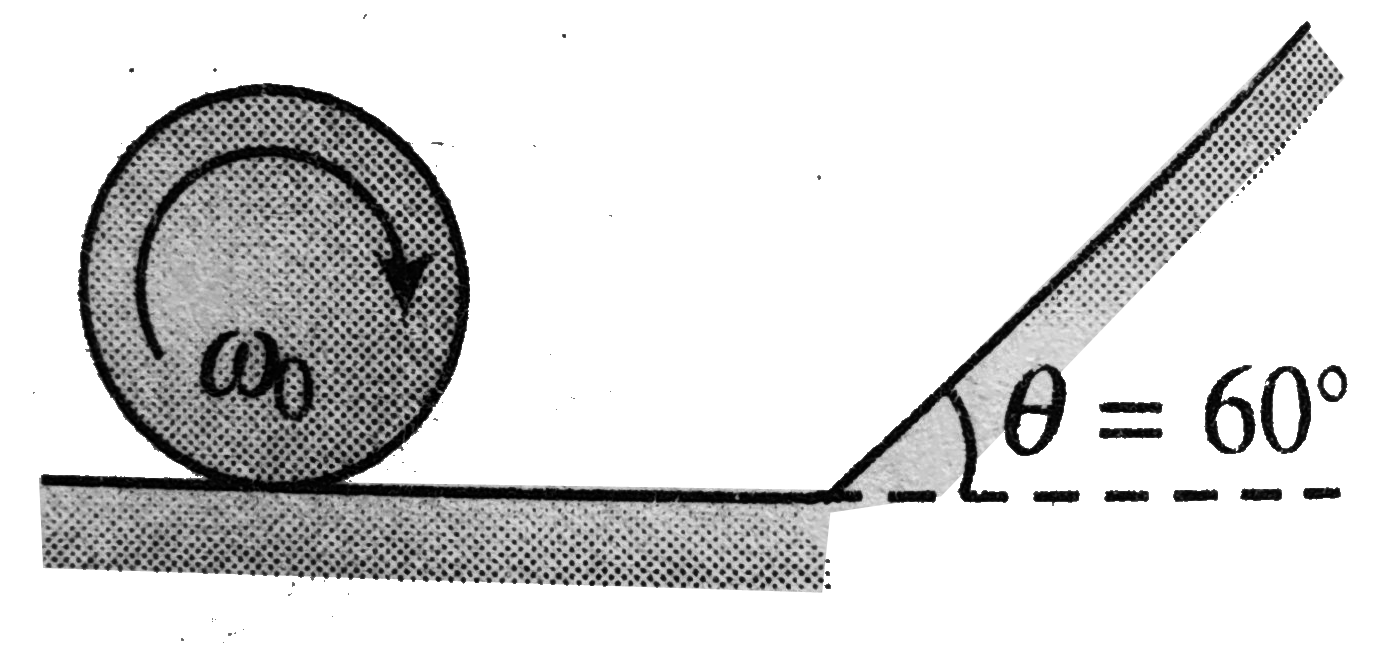
View SolutionA solid sphere rolls down two different inclined planes of the same height but of different inclinations
View SolutionA solid sphere of mass m rolls without slipping on an inclined plane of inclination θ. The linear acceleration of the sphere is
View SolutionA solid sphere rolls without slipping down a 30∘ inclined plane. If g=10ms−2 then the acceleration of the rolling sphere is
View Solution