The distance between the vertex and the center of mass of a uniform solid planar circular segment of angular size θ and radius R is given by -
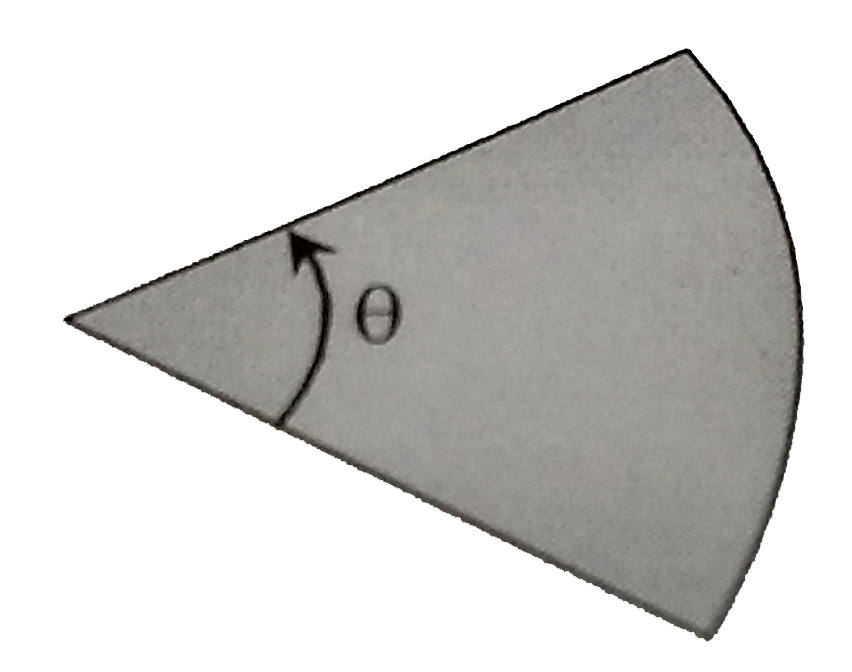
43Rsin(θ/2)θ
Rsin(θ/2)θ
43Rcos(θ2)
23Rcos(θ)
The correct Answer is:A
XCM=∫dmrsinθ/2θ/2∫dm[dm=σrθdr]
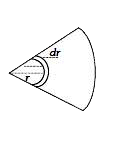
=2σsinθ/2∫R0r2drσθ∫R0rdr⇒43Rsin(θ/2)θ
Topper's Solved these Questions
- ROTATIONAL MOTIONBook:ERRORLESSChapter:ROTATIONAL MOTIONExercise:NCERT BASED QUESTIONS (Angular Displacement, Velocity and Acceleration)
Explore 34 Videos
- ROTATIONAL MOTIONBook:ERRORLESSChapter:ROTATIONAL MOTIONExercise:NCERT BASED QUESTIONS (Moment of Inertia)
Explore 139 Videos
- NEWTON'S LAWS OF MOTIONBook:ERRORLESSChapter:NEWTON'S LAWS OF MOTIONExercise:ASSERTION & REASON
Explore 18 Videos
- SIMPLE HARMONIC MOTIONBook:ERRORLESSChapter:SIMPLE HARMONIC MOTIONExercise:Assertion & Reason
Explore 15 Videos
Similar Questions
Knowledge Check
The moment of inertia of a uniform circular disc of mass M and of radius R about one of its diameter is
A14MR2B12MR2C23MR2D25MR2A uniform circular disc of radius a is taken. A circular portion of radius b has been removed from it as shown in the figure. If the center of hole is at a distance c from the center of the disc, the distance x2 of the center of mass of the remaining part from the initial center of mass O is given by
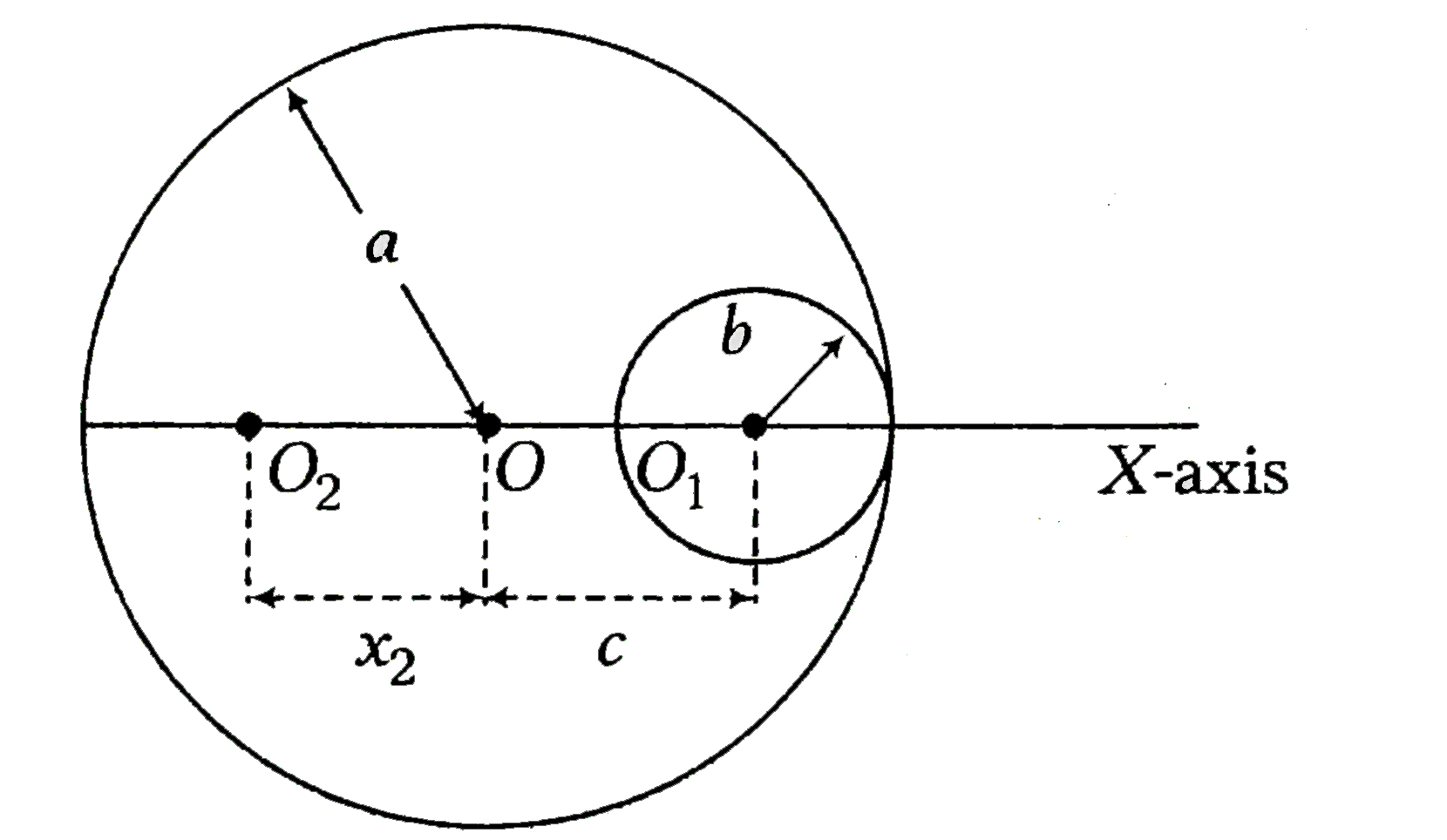 Aπb2a2−c2Bcb2a2−b2Cπc2a2−b2Dca2(c2−b2)
Aπb2a2−c2Bcb2a2−b2Cπc2a2−b2Dca2(c2−b2)The centre of mass of a solid cone along the line form the center of the base to the vertex is at
AOne-fourth of the heightBOne-third of the heightCOne-fifth of the heightDNone of these
Find moment of inertia of a uniform solid sphere (mass m, radius r) about a tangent.
View SolutionFind the distance of centre of mass of a uniform cone of height 'h' and base radius R, from the vertex on the line of symmetry .
View SolutionA uniform circular disc of radius a is taken. A circular portion of radius b has been removed from it as shown in the figure. If the center of hole is at a distance c from the center of the disc, the distance x2 of the center of mass of the remaining part from the initial center of mass O is given by

View SolutionThe magnetic field produced at the center of a current carrying circular coil of radius r, is
View Solutionr denotes the distance between the sun and the earth. Assume that the earth moves around the sun in a circular orbit of radius r. The angular momentum of the earth around the sun is proportional to
View Solution