Electric charges +q1,−q1,+q2 and −q2 are placed in free space and S is a spherical Gaussian surface. The electric flux passing over the surface S is:

due to +q2 only
due to all charges
zero
due to positive charges only
The correct Answer is:C

According to Gauss.s theorem the total flux coming out of a closed surface (S) is the ratio of the total charge contained in it and the permittivity of the free space (ε0).
Total flux (ϕ)=∑iqiε0
ϕ=(−q1+q2+q1−q2)ε0
ϕ=0
Similar Questions
A charge q is placed at the centre of a cube. What is the electric flux passing through the cube?
View Solutionq1,q2,q3 and q4 are point charges located at point as shown in the figure and s is a spherical Gaussian surface of radius R. Which of the following is true according to the Gauss's law

View Solution
Knowledge Check
A closed surface in vacuum encloses charges −qand+3q. The total electric flux emerging out of the surface is:
AzeroB2q/ε0C3q/ε0D4q/ε0
A charge q is placed at the centre of a cube of side l what is the electric flux passing through two opposite faces of the cube ?
View SolutionA charge q is placed at the centre of a cube of side l what is the electric flux passing through two opposite faces of the cube ?
View SolutionA charge q is placed at point D of the cube. Find the electric flux passing through the EFGH and face AEHD.
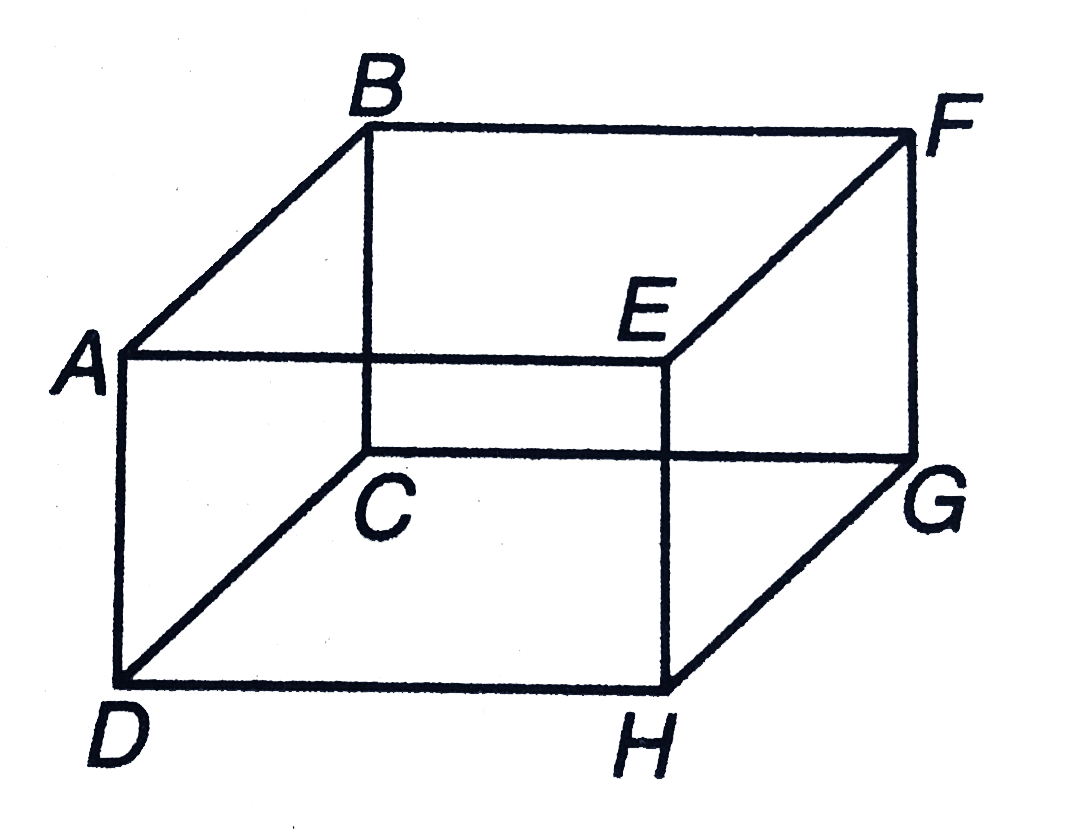
View SolutionFour electric charges +q,+q,−q and −q are placed at the corners of a square of side 2L (see figure). The electric potential at point A, mid-way between the two charges +q and +q, is
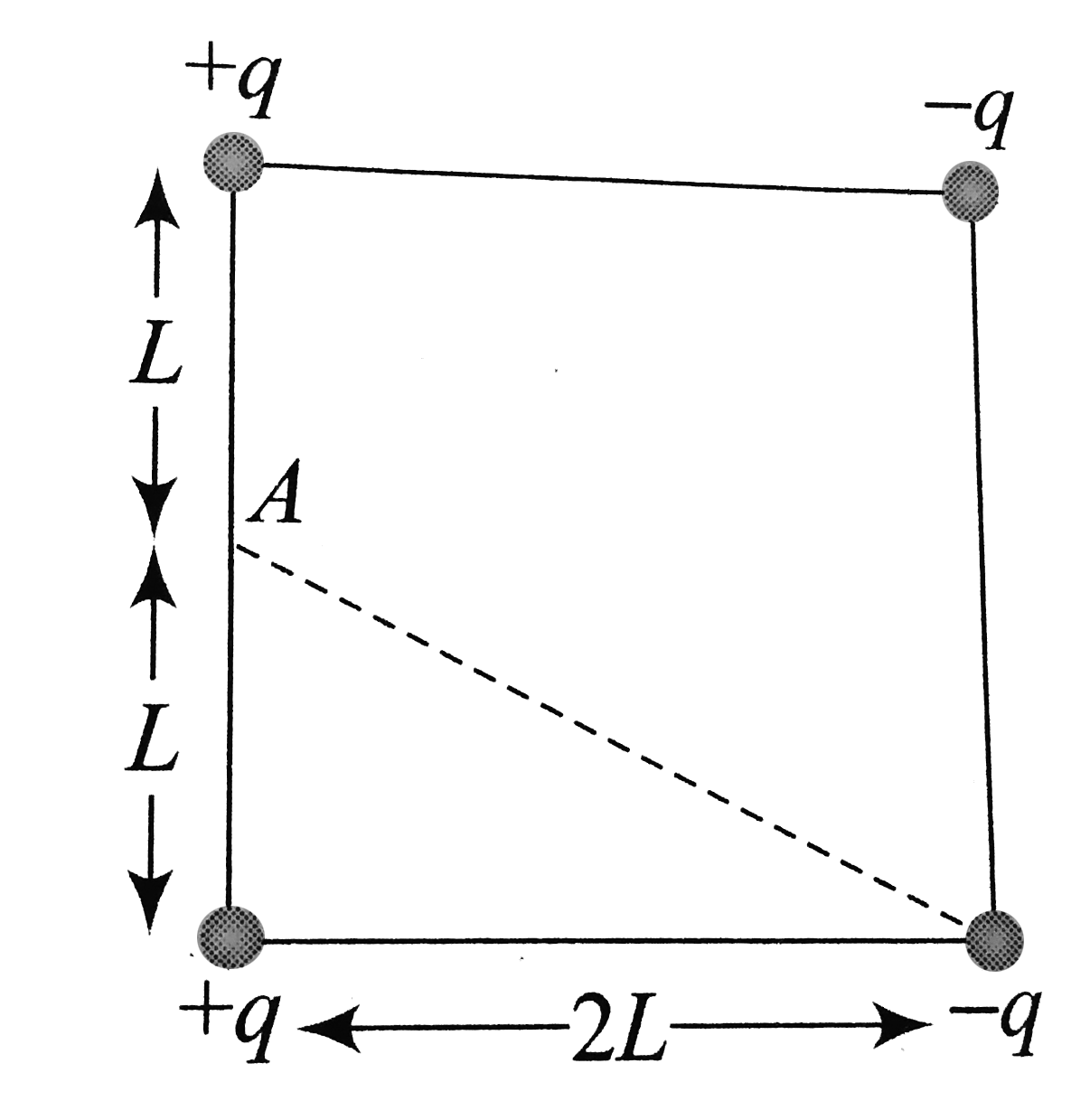
View SolutionTwo electric charges q1=qandq2=−2q are placed at a distance l = 6a apart. Find the locus of points in the plane of the charges where the field potential is zero
View SolutionThree charges −q1,+q2 and −q3 are placed as shown in the figure. The x-component of the force on −q1 is proportional to

View SolutionFigure shows three point charges +2q,-q and +3q. Two charges +2q and -q are enclosed within a surface .S.. What is the electric flux due to this configuration through the surface.S?
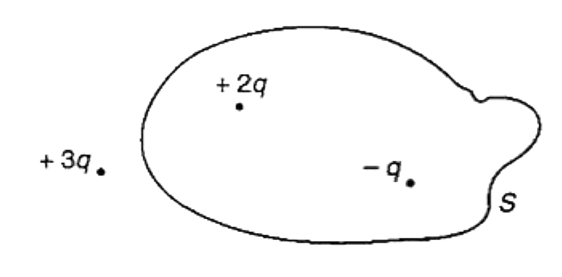
View Solution
