A block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.Solution in Gujrati
Supose the length of the spring is stretche by a length △l. The tension in the spring is k△l and this is the force by the spring on the block. The other force on the block is mg due to gravity. For equilibrium, mg=k△lor△l=mgk. Take this position of the block as x=0. If block is further displaced by x the resultant force is k(mgk+x)−mg=kx.
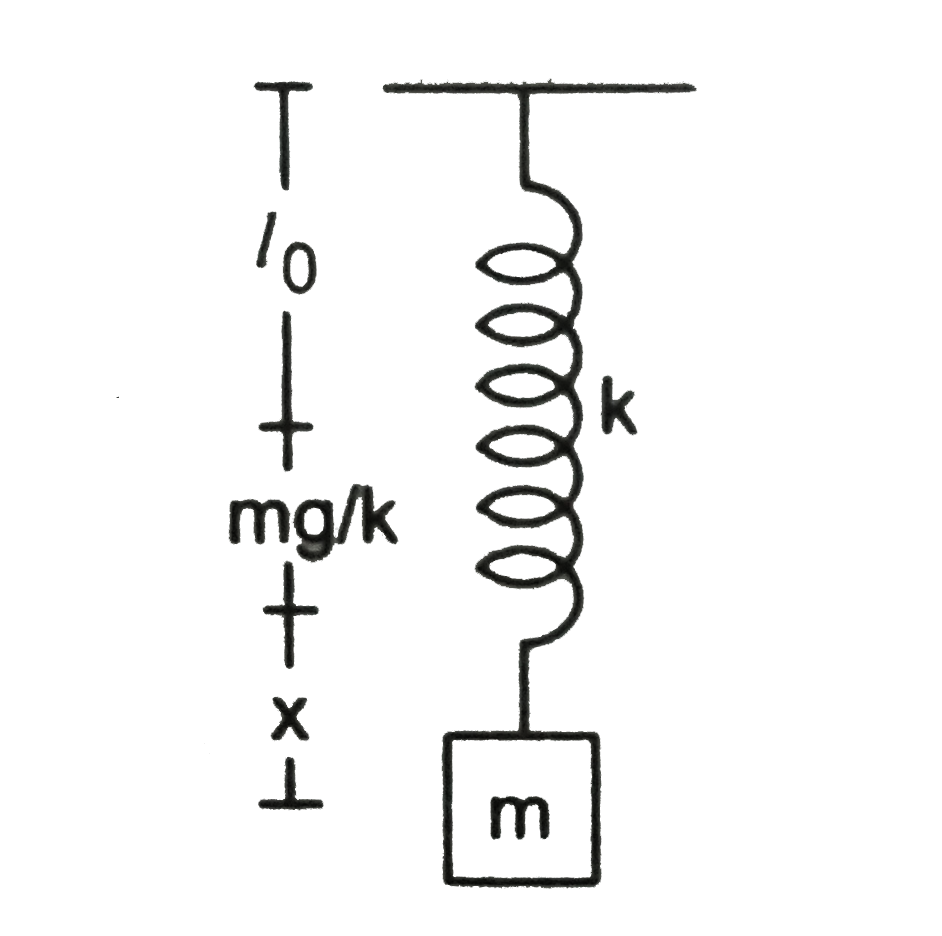
Thus the resultnt force is proportioN/Al to the displacement. The motion is simple harmonic with a the period T=2π√mk
We see that in vertical osciltions, gravity has no effect on time period. The only effect it has is to shift the equilibrium position by a distance mg/k s if the N/Atural length is increased (or decreased if the lower end of the spring is fixed) by mg/k.
Similar Questions
A ball of mass 5kg hangs from a spring of force constant k N/m and oscillates with certain time period. If the ball is removed, the spring shortens by
View SolutionA ball of mass 5kg hangs from a spring of force constant K N/m and oscillates tain time period. If the ball is removed, the spring shortens by
View SolutionA ball of mass 5kg hangs from a spring of force constant k N//m and oscillates with certain time period. If the ball is removed, the spring shortens by
View Solutionएक m द्रव्यमान का गुटका स्प्रिंग नियतांक k की स्प्रिंग से ऊर्ध्वाधर रूप से लटक रहा है। यदि द्रव्यमान को ऊर्ध्वाधर रूप से दोलन कराया जाता है, तो इसकी कुल ऊर्जा होती है
View SolutionA block of mass m is connected with two ideal pullies and a massless spring of spring constant K as shown in figure. The block is slightly displaced from its equilibrium position. If the time period of oscillation is mupisqrt(m/K) . Then find the value of mu .
View SolutionA block of mass m hangs from three springs having same spring constant k. If the mass is slightly displaced downwards, the time period of oscillation will be
View SolutionFind the time period of oscillation of block of mass m. Spring, and pulley are ideal. Spring constant is k.
View SolutionA body of mass 'm' hangs from three springs, each of spring constant 'k' as shown in the figure. If the mass is slightly displaced and let go, the system will oscillate with time period–
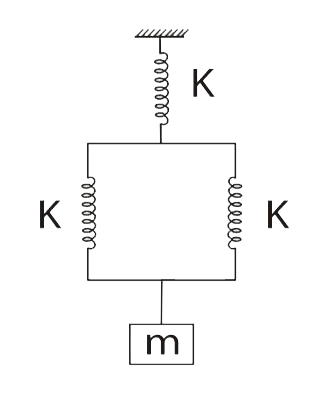
View Solution
